Subtracting Mixed Numbers With Regrouping Made Easy
Mastering the Art of Subtracting Mixed Numbers with Regrouping
When it comes to subtracting mixed numbers with regrouping, many students find themselves struggling to understand the concept. However, with the right approach and practice, this math operation can become a breeze. In this article, we will break down the steps to subtract mixed numbers with regrouping, making it easy for you to grasp and apply.
Understanding Mixed Numbers and Regrouping
Before diving into the subtraction process, let’s quickly review what mixed numbers and regrouping mean:
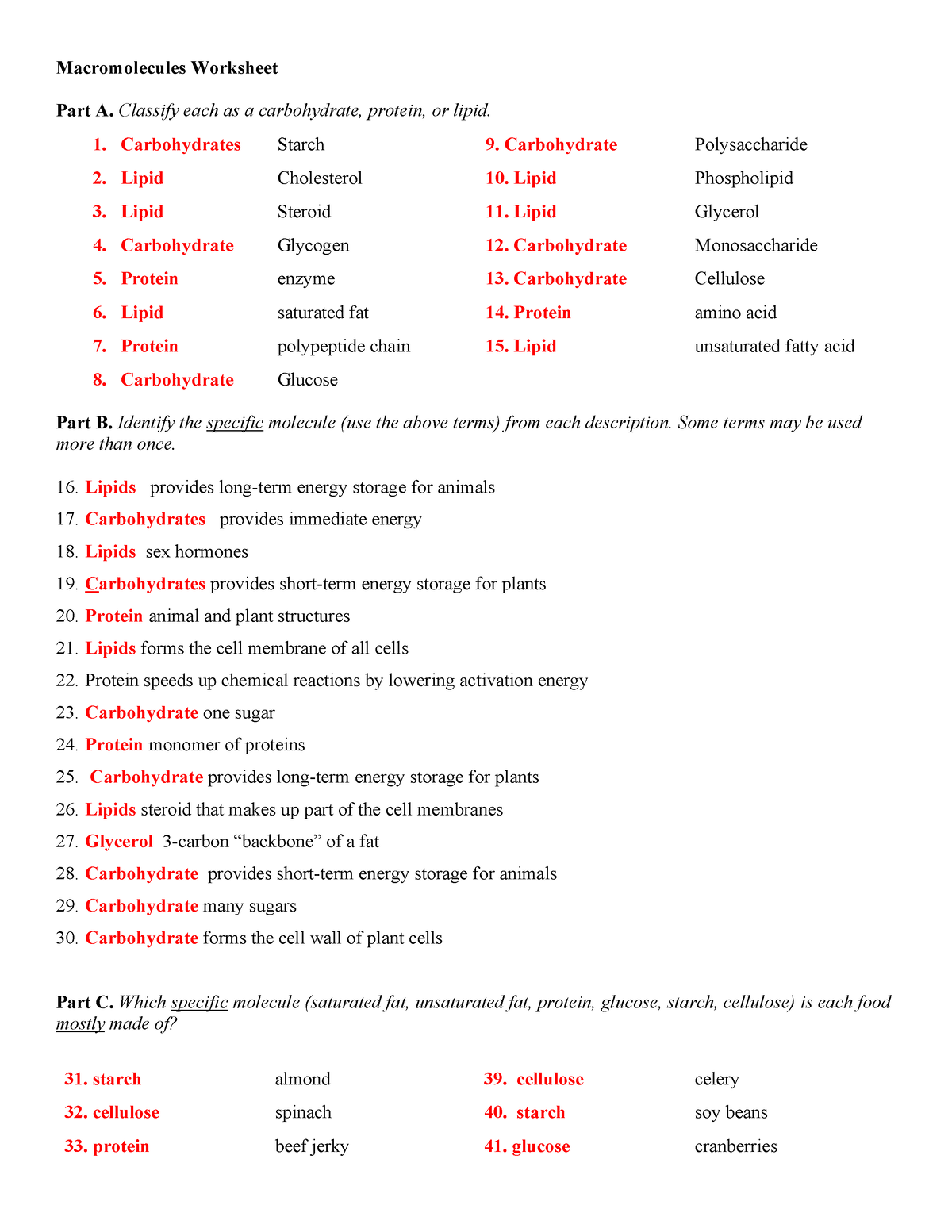
- A mixed number is a combination of a whole number and a fraction. For example, 2 1⁄2.
- Regrouping, also known as “borrowing,” is the process of exchanging one unit of a higher place value for multiple units of a lower place value. In the context of mixed numbers, regrouping occurs when we need to subtract a fraction from a whole number.
Step-by-Step Guide to Subtracting Mixed Numbers with Regrouping
Now that we have a solid understanding of mixed numbers and regrouping, let’s move on to the step-by-step process of subtracting mixed numbers with regrouping.
Step 1: Write the Mixed Numbers
Start by writing the two mixed numbers you want to subtract. Make sure to line up the whole numbers and fractions correctly.
Step 2: Check if Regrouping is Necessary
Determine if regrouping is necessary by comparing the fractions. If the fraction being subtracted is larger than the fraction in the minuend (the first mixed number), you will need to regroup.
Step 3: Regroup the Whole Number (if necessary)
If regrouping is necessary, exchange one whole unit from the minuend for the equivalent number of fractional units. For example, if you have 2 1⁄2 and you need to subtract 3⁄4, you can regroup 1 whole unit from the 2 to get 1 1⁄2 and 2⁄2, which is equivalent to 1 1⁄2 + 1.
Step 4: Subtract the Fractions
Subtract the fractions, making sure to line up the numerators and denominators correctly. If necessary, simplify the resulting fraction.
Step 5: Subtract the Whole Numbers
Subtract the whole numbers, taking into account the regrouping done in Step 3.
Step 6: Write the Final Answer
Combine the results from Steps 4 and 5 to write the final answer in mixed number form.
👉 Note: When subtracting mixed numbers with regrouping, it's essential to keep track of the place values and perform the operations in the correct order.
Example Problems
Let’s work through some example problems to illustrate the steps.
Example 1: Subtracting Mixed Numbers with Regrouping
Subtract 2 1⁄2 from 4 3⁄4.

| Whole Numbers | Fractions | |
|---|---|---|
| Minuend | 4 | 3/4 |
| Subtrahend | 2 | 1/2 |
Step 1: Write the Mixed Numbers
4 3⁄4 - 2 1⁄2
Step 2: Check if Regrouping is Necessary
Regrouping is necessary since 1⁄2 is smaller than 3⁄4.
Step 3: Regroup the Whole Number (if necessary)
Regroup 1 whole unit from the 4 to get 3 1⁄4.
Step 4: Subtract the Fractions
3⁄4 - 1⁄2 = 1⁄4
Step 5: Subtract the Whole Numbers
3 - 2 = 1
Step 6: Write the Final Answer
1 1⁄4
Example 2: Subtracting Mixed Numbers with Regrouping
Subtract 3 1⁄3 from 5 2⁄3.
| Whole Numbers | Fractions | |
|---|---|---|
| Minuend | 5 | 2/3 |
| Subtrahend | 3 | 1/3 |
Step 1: Write the Mixed Numbers
5 2⁄3 - 3 1⁄3
Step 2: Check if Regrouping is Necessary
Regrouping is necessary since 1⁄3 is smaller than 2⁄3.
Step 3: Regroup the Whole Number (if necessary)
Regroup 1 whole unit from the 5 to get 4 2⁄3.
Step 4: Subtract the Fractions
2⁄3 - 1⁄3 = 1⁄3
Step 5: Subtract the Whole Numbers
4 - 3 = 1
Step 6: Write the Final Answer
1 1⁄3
Common Challenges and Solutions
- Challenge 1: Forgetting to Regroup
Solution: Always check if regrouping is necessary by comparing the fractions. If the fraction being subtracted is larger, regroup the whole number.
- Challenge 2: Incorrectly Subtracting Fractions
Solution: Make sure to line up the numerators and denominators correctly when subtracting fractions. Simplify the resulting fraction if necessary.
- Challenge 3: Confusing Whole Numbers and Fractions
Solution: Keep track of the place values and perform the operations in the correct order. Separate the whole numbers and fractions, and subtract them individually.
👉 Note: Practice makes perfect. Make sure to work through various examples to become comfortable with subtracting mixed numbers with regrouping.
In conclusion, subtracting mixed numbers with regrouping requires attention to detail and a solid understanding of the concept. By following the step-by-step guide and practicing with example problems, you can master this math operation and become more confident in your math skills.
What is regrouping in math?
+Regrouping, also known as “borrowing,” is the process of exchanging one unit of a higher place value for multiple units of a lower place value. In the context of mixed numbers, regrouping occurs when we need to subtract a fraction from a whole number.
How do I know if regrouping is necessary when subtracting mixed numbers?
+Regrouping is necessary when the fraction being subtracted is larger than the fraction in the minuend (the first mixed number). You can determine this by comparing the fractions.
What are some common challenges when subtracting mixed numbers with regrouping?
+Common challenges include forgetting to regroup, incorrectly subtracting fractions, and confusing whole numbers and fractions. To overcome these challenges, it’s essential to follow the step-by-step guide and practice with example problems.
Related Terms:
- Subtracting fractions regrouping worksheets
- Regrouping mixed numbers