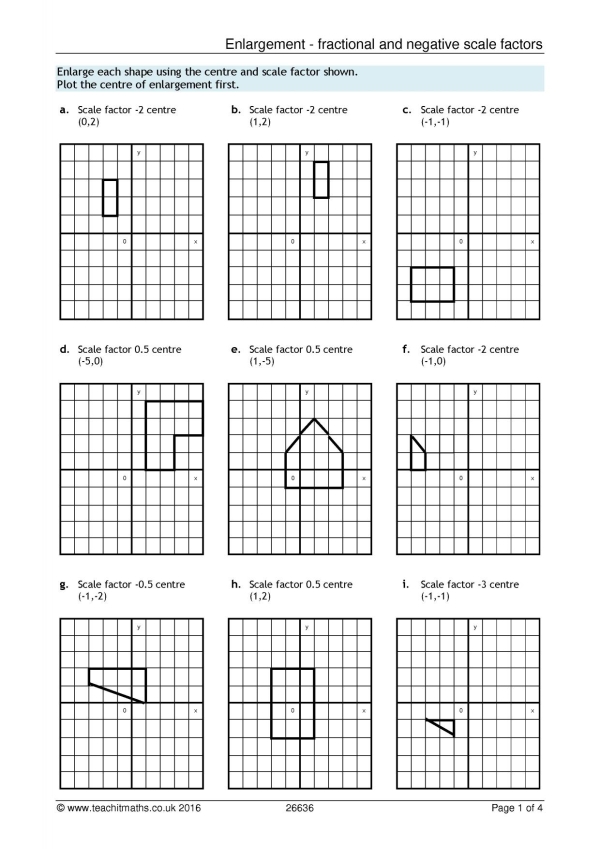
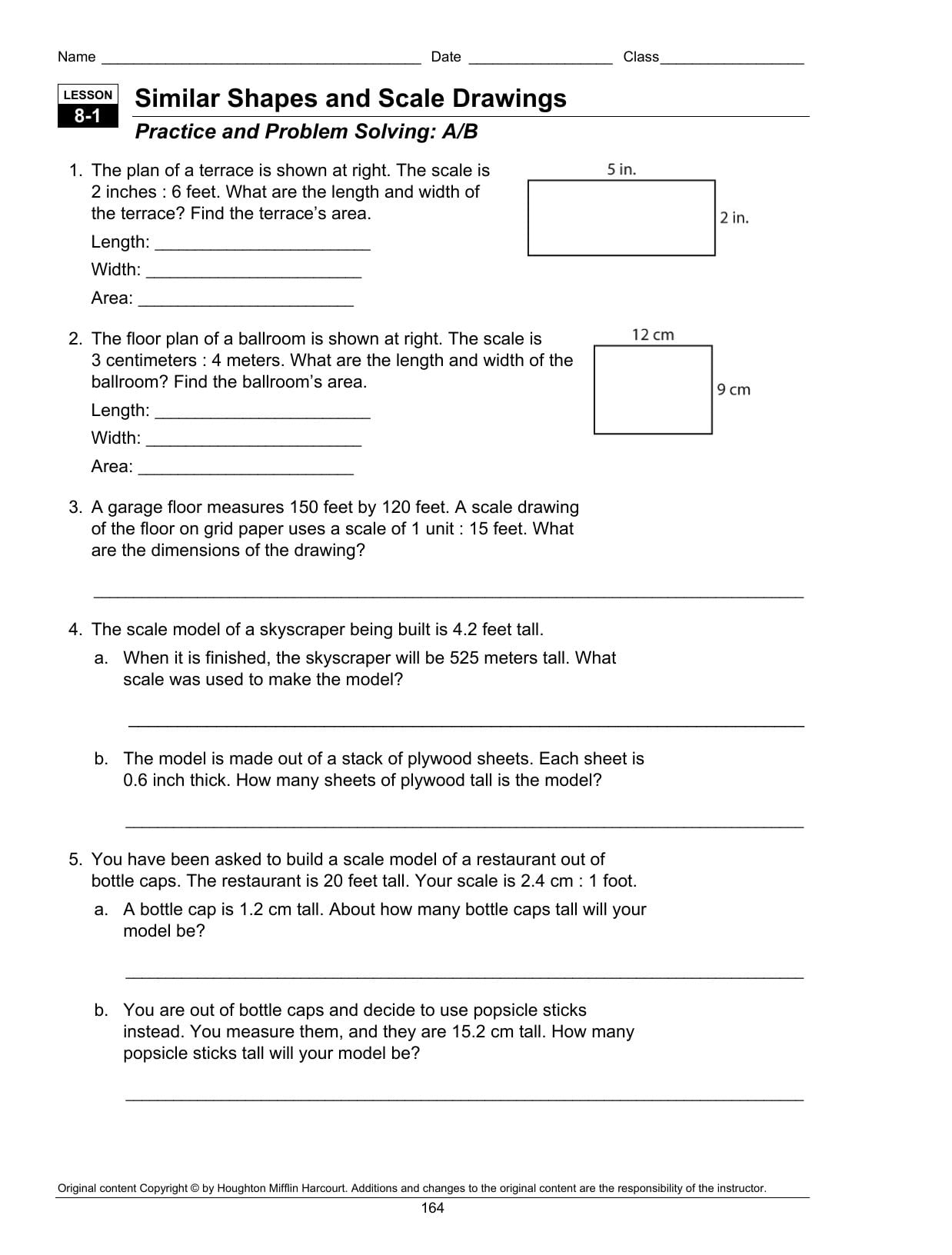
5 Ways to Master Scale Factor Worksheets
Unlocking the Secrets of Scale Factor Worksheets
Scale factor worksheets are an essential tool for students to master the concept of scaling and proportionality. However, many students struggle to understand the concept, leading to frustration and difficulty in solving problems. In this article, we will explore five ways to master scale factor worksheets, making it easier for students to grasp the concept and apply it to real-world problems.
Understanding the Basics of Scale Factor
Before diving into the ways to master scale factor worksheets, it’s essential to understand the basics of scale factor. A scale factor is a ratio of the corresponding sides of two similar figures. It’s a fundamental concept in geometry and is used to describe the relationship between two similar shapes. The scale factor can be used to enlarge or reduce a shape, and it’s essential to understand how to calculate and apply it.
1. Visualize the Problem
One of the most effective ways to master scale factor worksheets is to visualize the problem. Students should be encouraged to draw diagrams and pictures to represent the problem. This will help them understand the relationship between the two shapes and make it easier to calculate the scale factor.
Example: Draw a picture of two similar triangles, one with a side length of 6 cm and the other with a side length of 12 cm. What is the scale factor?
Answer: The scale factor is 2:1, as the corresponding sides of the two triangles are in the ratio of 2:1.
2. Use Real-World Examples
Using real-world examples is an excellent way to make scale factor worksheets more engaging and relevant. Students can use everyday objects to demonstrate the concept of scale factor. For example, they can use a map to demonstrate the scale factor between a real-world location and its representation on the map.
Example: A map has a scale of 1:100,000. If a road on the map measures 5 cm, how long is the actual road?
Answer: The actual road is 5 km long, as the scale factor is 1:100,000.
3. Practice, Practice, Practice
Practice is key to mastering scale factor worksheets. Students should be provided with a variety of worksheets that cover different types of problems, from simple to complex. The more they practice, the more confident they will become in applying the concept of scale factor.
Example: A scale factor worksheet with 10 problems, ranging from simple to complex, can help students practice and reinforce their understanding of the concept.
4. Use Technology to Your Advantage
Technology can be a valuable tool in mastering scale factor worksheets. There are many online resources and apps that provide interactive worksheets and games to help students practice and learn. These resources can make learning more engaging and fun, and can provide immediate feedback and assessment.
Example: An online worksheet generator can provide students with customized worksheets that cater to their needs and abilities.
5. Break Down Complex Problems
Finally, breaking down complex problems into simpler ones is an effective way to master scale factor worksheets. Students should be encouraged to identify the key elements of the problem and break them down into manageable parts. This will help them understand the problem better and make it easier to calculate the scale factor.
Example: A complex problem involving multiple shapes and scale factors can be broken down into simpler problems, making it easier to calculate the overall scale factor.
💡 Note: Breaking down complex problems into simpler ones can help students understand the problem better and make it easier to calculate the scale factor.
| Scale Factor | Description | Example |
|---|---|---|
| 1:1 | Equal ratio | Two identical shapes |
| 2:1 | Enlargement | A shape enlarged by a factor of 2 |
| 1:2 | Reduction | A shape reduced by a factor of 2 |
Conclusion
Mastering scale factor worksheets requires a combination of understanding the basics, visualizing the problem, using real-world examples, practicing regularly, using technology to your advantage, and breaking down complex problems. By following these tips, students can become proficient in solving scale factor problems and develop a deeper understanding of the concept. Remember, practice is key, and with persistence and dedication, anyone can master scale factor worksheets.
What is a scale factor?
+A scale factor is a ratio of the corresponding sides of two similar figures.
How do I calculate the scale factor?
+The scale factor is calculated by dividing the length of the corresponding side of the larger shape by the length of the corresponding side of the smaller shape.
What are some real-world examples of scale factor?
+Maps, blueprints, and architectural models are all examples of scale factor in real-world applications.