Solve Simultaneous Equations Easily with Our Worksheet
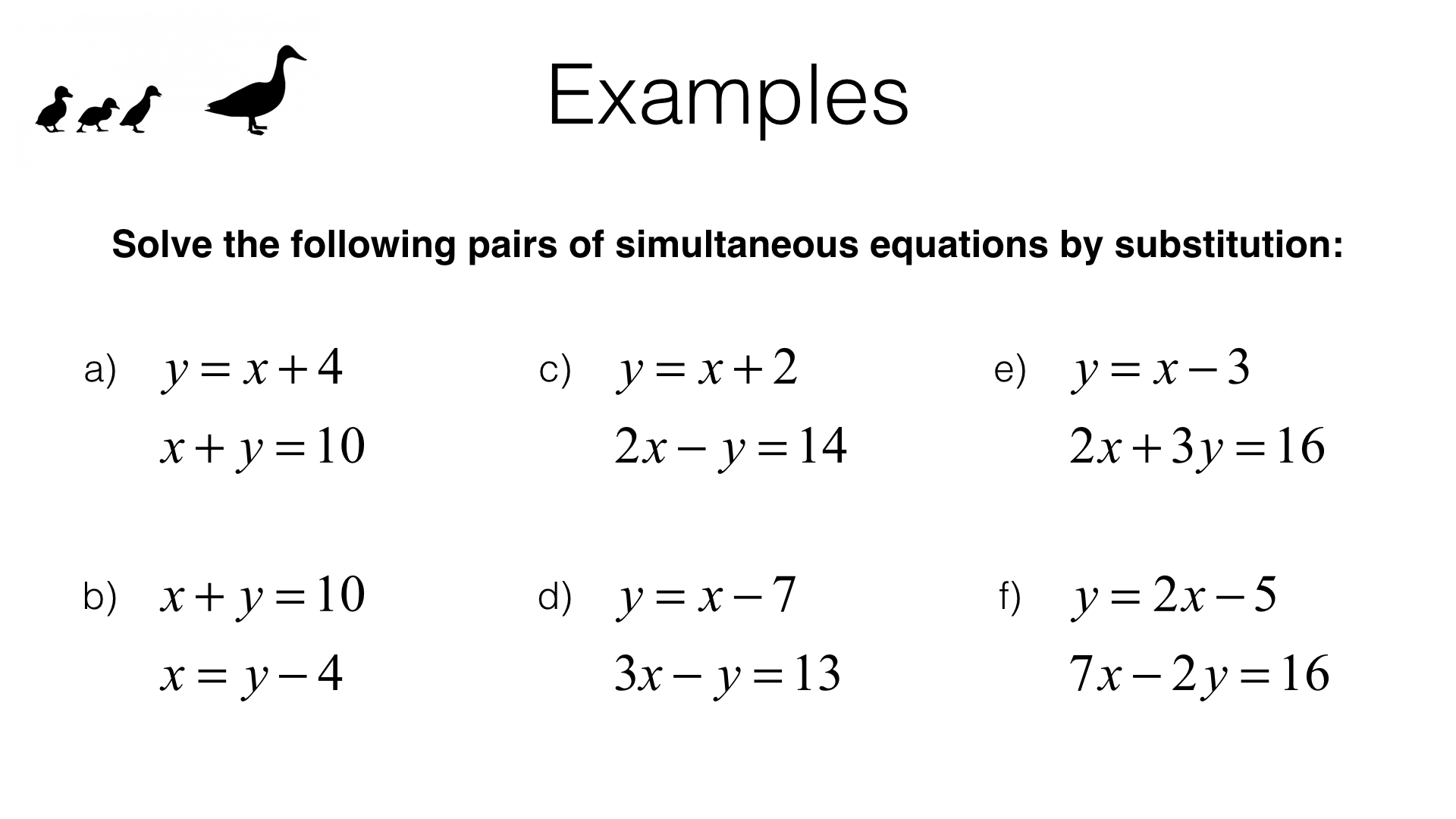
Why Solving Simultaneous Equations is Important
Solving simultaneous equations is an essential skill in mathematics, particularly in algebra. It involves finding the values of two or more variables that satisfy multiple equations simultaneously. This skill is crucial in various fields, including physics, engineering, economics, and computer science. In this blog post, we will explore the methods and techniques for solving simultaneous equations and provide a worksheet to help you practice.
Methods for Solving Simultaneous Equations
There are several methods for solving simultaneous equations, including:
- Substitution Method: This method involves solving one equation for one variable and then substituting that expression into the other equation.
- Elimination Method: This method involves adding or subtracting the equations to eliminate one variable.
- Graphical Method: This method involves graphing the equations on a coordinate plane and finding the point of intersection.
📝 Note: The substitution method is often the easiest method to use, especially when one equation is already solved for one variable.
Step-by-Step Guide to Solving Simultaneous Equations
Here’s a step-by-step guide to solving simultaneous equations using the substitution method:
- Write down the equations and identify the variables.
- Solve one equation for one variable.
- Substitute the expression from step 2 into the other equation.
- Solve for the other variable.
- Check your solution by plugging it back into both equations.
Example: Solving Simultaneous Equations
Suppose we want to solve the following simultaneous equations:
2x + 3y = 7 x - 2y = -3
We can solve this system using the substitution method.
Step 1: Write down the equations and identify the variables.
- 2x + 3y = 7
- x - 2y = -3
Step 2: Solve one equation for one variable.
- Solve the second equation for x: x = -3 + 2y
Step 3: Substitute the expression from step 2 into the other equation.
- Substitute x into the first equation: 2(-3 + 2y) + 3y = 7
Step 4: Solve for the other variable.
- Simplify the equation: -6 + 4y + 3y = 7
- Combine like terms: 7y = 13
- Solve for y: y = 13⁄7
Step 5: Check your solution by plugging it back into both equations.
- Plug y into the first equation: 2x + 3(13⁄7) = 7
- Simplify: 2x + 39⁄7 = 7
- Solve for x: x = 1
Worksheet: Solving Simultaneous Equations
Here is a worksheet to help you practice solving simultaneous equations:

| Equations | Solution | |
|---|---|---|
| x + 2y = 4 | x - y = 1 | |
| 3x - 2y = 5 | x + 3y = 7 | |
| 2x + 5y = 11 | x - 4y = -3 |
Tips and Tricks
Here are some tips and tricks to help you solve simultaneous equations:
- Use the substitution method: This method is often the easiest method to use, especially when one equation is already solved for one variable.
- Check your solution: Always check your solution by plugging it back into both equations.
- Use a table or graph: Using a table or graph can help you visualize the solution and make it easier to find.
In conclusion, solving simultaneous equations is an important skill in mathematics. By following the steps outlined in this blog post and practicing with the worksheet, you can become proficient in solving simultaneous equations.
What is the substitution method?
+The substitution method involves solving one equation for one variable and then substituting that expression into the other equation.
What is the elimination method?
+The elimination method involves adding or subtracting the equations to eliminate one variable.
Why is it important to check your solution?
+Checking your solution ensures that it satisfies both equations and is therefore a valid solution.
Related Terms:
- Percentage change worksheet pdf
- Quadratic simultaneous equations
- Linear graph Worksheet pdf
- Word problem simultaneous equations