7 Ways to Simplify Radical Expressions
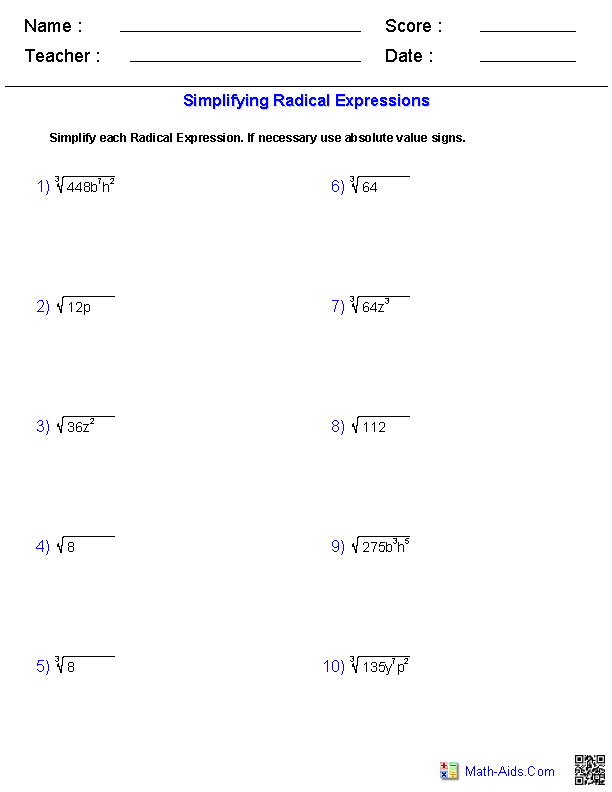
Simplifying radical expressions is a fundamental concept in mathematics, particularly in algebra and geometry. Radical expressions, also known as radicals, involve the square root of a number or an expression. Simplifying these expressions involves expressing them in the most straightforward form possible, without changing their value. Here are seven ways to simplify radical expressions:
1. Factor Out Perfect Squares
When simplifying radical expressions, the first step is to look for perfect squares that can be factored out. A perfect square is a number that can be expressed as the square of an integer, such as 4, 9, or 16. By factoring out perfect squares, you can simplify the radical expression and make it easier to work with.
For example, the expression √(4x^2) can be simplified by factoring out the perfect square:
√(4x^2) = √(2^2 * x^2) = 2x
2. Use the Quotient Rule
The quotient rule is a method for simplifying radical expressions that involve division. According to the quotient rule, the square root of a quotient is equal to the quotient of the square roots:
√(a/b) = √a / √b
For example, the expression √(16⁄9) can be simplified using the quotient rule:
√(16⁄9) = √16 / √9 = 4⁄3
3. Use the Product Rule
The product rule is a method for simplifying radical expressions that involve multiplication. According to the product rule, the square root of a product is equal to the product of the square roots:
√(ab) = √a * √b
For example, the expression √(4*9) can be simplified using the product rule:
√(4*9) = √4 * √9 = 2*3 = 6
4. Simplify Radical Expressions with Coefficients
Radical expressions can also have coefficients, which are numbers that are multiplied by the radical expression. To simplify radical expressions with coefficients, you can factor out the coefficient and simplify the remaining expression.
For example, the expression 2√(4x^2) can be simplified by factoring out the coefficient:
2√(4x^2) = 2 * 2x = 4x
5. Rationalize the Denominator
Rationalizing the denominator is a method for simplifying radical expressions that involve division, where the denominator contains a radical. To rationalize the denominator, you can multiply the numerator and denominator by the radical in the denominator.
For example, the expression 1/√2 can be rationalized by multiplying the numerator and denominator by √2:
1/√2 = (√2/√2) / (√2/√2) = √2 / 2
6. Use Conjugates to Simplify Radical Expressions
Conjugates are pairs of binomials that have the same terms, but opposite signs. To simplify radical expressions using conjugates, you can multiply the numerator and denominator by the conjugate of the binomial.
For example, the expression (√2 + 1) / (√2 - 1) can be simplified by multiplying the numerator and denominator by the conjugate of the denominator:
(√2 + 1) / (√2 - 1) = ((√2 + 1) * (√2 + 1)) / ((√2 - 1) * (√2 + 1)) = (√2 + 1)^2 / (2 - 1) = (√2 + 1)^2
7. Simplify Radical Expressions with Variables
Radical expressions can also involve variables, which can be simplified using the same methods as above. When simplifying radical expressions with variables, it’s essential to be careful with the signs and exponents.
For example, the expression √(x^2 + 4) can be simplified by factoring out the perfect square:
√(x^2 + 4) = √(x^2 + 2^2) = √((x + 2)^2) = x + 2
📝 Note: When simplifying radical expressions with variables, make sure to check the domain of the expression to ensure that it is defined for all values of the variable.
To summarize, simplifying radical expressions involves using various methods, such as factoring out perfect squares, using the quotient and product rules, simplifying expressions with coefficients, rationalizing the denominator, using conjugates, and simplifying expressions with variables. By mastering these methods, you can simplify radical expressions and make them easier to work with.
Some key points to remember:
• Always look for perfect squares that can be factored out. • Use the quotient and product rules to simplify expressions that involve division and multiplication. • Rationalize the denominator when working with expressions that involve division. • Use conjugates to simplify expressions with binomials. • Be careful with signs and exponents when working with variables.
By following these tips and practicing regularly, you can become proficient in simplifying radical expressions and develop a deeper understanding of mathematics.
What is a radical expression?
+A radical expression is an expression that involves the square root of a number or an expression.
Why is it essential to simplify radical expressions?
+Simplifying radical expressions makes them easier to work with and understand, which is essential for solving equations and problems in mathematics.
Can radical expressions involve variables?
+Yes, radical expressions can involve variables, and simplifying them requires careful attention to signs and exponents.
Related Terms:
- Simplifying Radicals Worksheet pdf
- Simplifying radical Expressions worksheet
- Simplifying Radicals Worksheet Kuta Software