5 Ways to Master Triangle Inequality Theorem
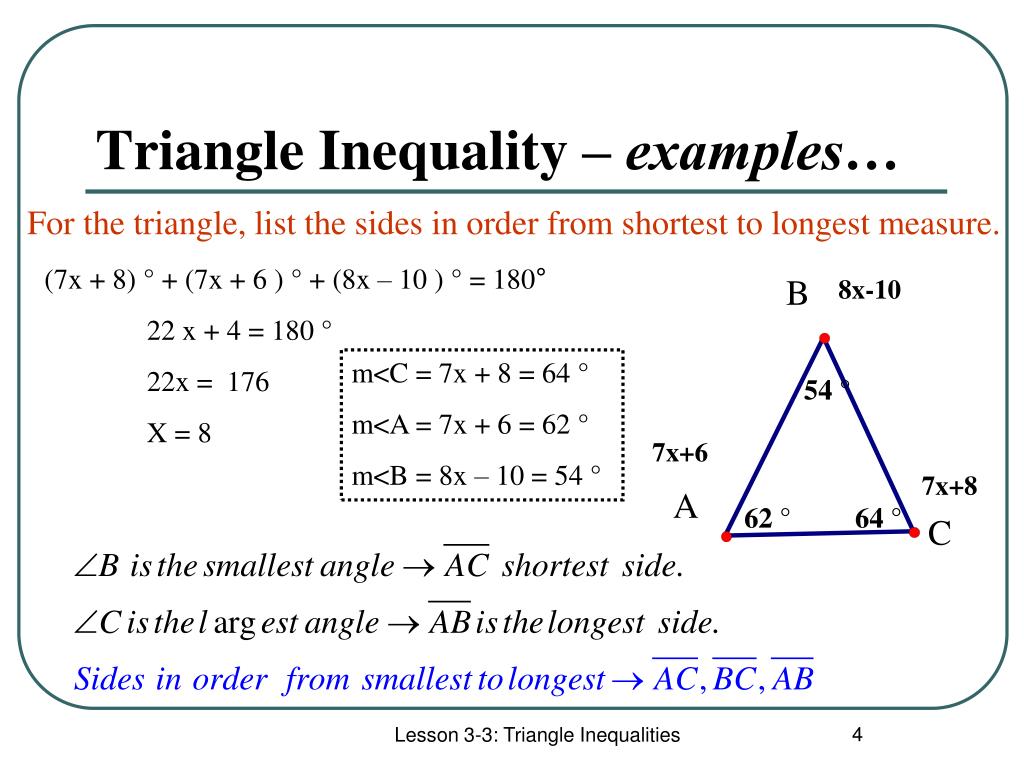
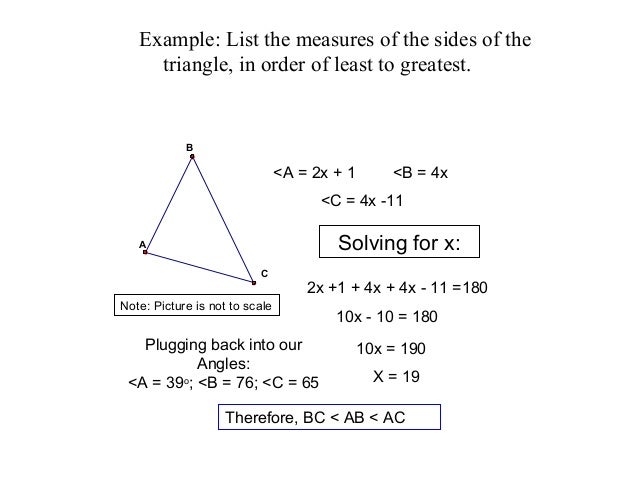
Understanding the Triangle Inequality Theorem
The Triangle Inequality Theorem is a fundamental concept in geometry that describes the relationship between the lengths of the sides of a triangle. It states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. This theorem is essential in various mathematical and real-world applications, including trigonometry, geometry, and physics. Mastering the Triangle Inequality Theorem can help you solve problems and prove theorems in mathematics and other fields.
What is the Triangle Inequality Theorem?
The Triangle Inequality Theorem can be stated as follows:
Theorem: The sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
Mathematically, this can be expressed as:
a + b > c
where a, b, and c are the lengths of the sides of the triangle.
5 Ways to Master Triangle Inequality Theorem
Mastering the Triangle Inequality Theorem requires practice, patience, and a deep understanding of its applications. Here are five ways to help you master this theorem:
1. Visualize the Theorem
Visualizing the theorem is an excellent way to understand its concept. Draw a triangle and label its sides. Then, try to move the vertices of the triangle to create different shapes and sizes. Observe how the lengths of the sides change and how they relate to each other. This visualization will help you understand the theorem intuitively.
2. Practice with Examples
Practice is key to mastering the Triangle Inequality Theorem. Start with simple examples, such as finding the length of the third side of a triangle given the lengths of the other two sides. Gradually move on to more complex problems, such as determining whether a triangle can be formed with given side lengths. You can find many examples online or in geometry textbooks.
3. Use Algebraic Manipulation
Algebraic manipulation can help you prove the theorem and solve problems related to it. Use the equation a + b > c to derive other inequalities, such as a + c > b and b + c > a. This will help you understand the relationships between the sides of a triangle.
4. Apply the Theorem to Real-World Problems
The Triangle Inequality Theorem has numerous applications in real-world problems, such as physics, engineering, and computer science. Try to find problems that involve the theorem and solve them using its principles. This will help you understand the practical significance of the theorem.
5. Teach Someone Else
Teaching someone else is an excellent way to master a concept. Explain the Triangle Inequality Theorem to a friend or family member and help them understand its applications. This will help you retain the information and develop your own understanding of the theorem.
Table of Triangle Inequality Theorem Applications
| Application | Description |
|---|---|
| Trigonometry | The theorem is used to derive trigonometric identities and solve triangles. |
| Geometry | The theorem is used to prove theorems and solve problems related to triangles and other polygons. |
| Physics | The theorem is used to calculate distances and velocities in physics problems. |
| Engineering | The theorem is used to design and optimize systems, such as bridges and buildings. |
| Computer Science | The theorem is used in algorithms and data structures, such as graph theory and computational geometry. |
📝 Note: The Triangle Inequality Theorem has many other applications in mathematics and real-world problems. The above table is not exhaustive.
By following these five ways, you can master the Triangle Inequality Theorem and develop a deep understanding of its applications. Remember to practice regularly and apply the theorem to real-world problems to reinforce your understanding.
The concept of the Triangle Inequality Theorem is a fundamental building block in mathematics and has numerous applications in various fields. Mastering this theorem can help you develop problem-solving skills and think critically about mathematical concepts. With practice and persistence, you can become proficient in applying the Triangle Inequality Theorem to a wide range of problems.
What is the Triangle Inequality Theorem?
+The Triangle Inequality Theorem states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
How can I master the Triangle Inequality Theorem?
+To master the Triangle Inequality Theorem, practice with examples, use algebraic manipulation, apply the theorem to real-world problems, teach someone else, and visualize the theorem.
What are some applications of the Triangle Inequality Theorem?
+The Triangle Inequality Theorem has numerous applications in trigonometry, geometry, physics, engineering, and computer science.