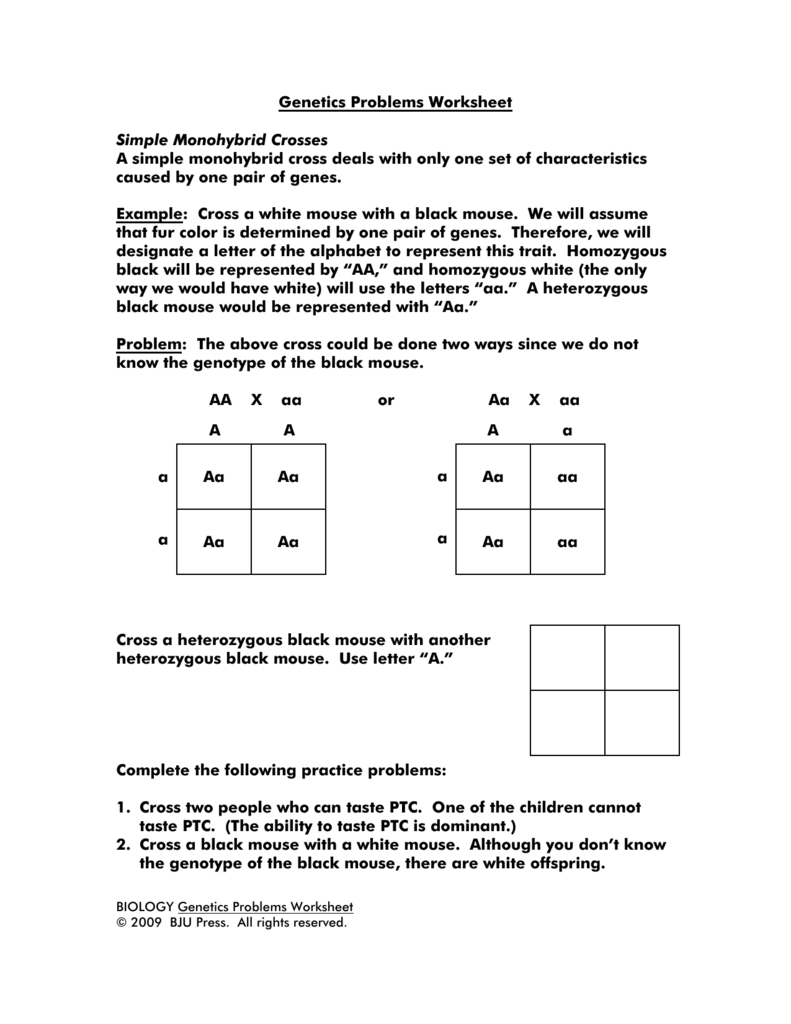
5 Ways to Multiply Polynomials with Ease
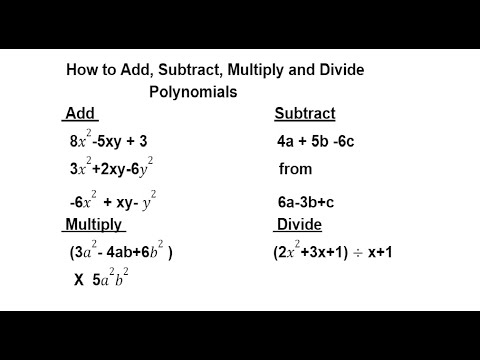
Understanding the Basics of Polynomial Multiplication
Multiplying polynomials can be a daunting task, especially for those who are new to algebra. However, with a solid understanding of the basics and some practice, you can master this skill with ease. In this article, we will explore five ways to multiply polynomials, including the distributive property, the FOIL method, and more.
The Distributive Property: A Fundamental Concept
The distributive property is a fundamental concept in algebra that allows you to expand a product of two binomials. It states that for any real numbers a, b, and c:
a(b + c) = ab + ac
This property can be applied to multiply polynomials by distributing each term in one polynomial to each term in the other polynomial.
Example:
Multiply 2x + 3 and x + 4 using the distributive property.
(2x + 3)(x + 4) = 2x(x + 4) + 3(x + 4) = 2x^2 + 8x + 3x + 12 = 2x^2 + 11x + 12
🤔 Note: When multiplying polynomials, make sure to combine like terms by adding or subtracting coefficients of the same variable.
The FOIL Method: A Handy Shortcut
The FOIL method is a handy shortcut for multiplying two binomials. FOIL stands for First, Outer, Inner, Last, which refers to the order in which you multiply the terms.
Example:
Multiply x + 3 and x + 5 using the FOIL method.
(x + 3)(x + 5) = x*x + x*5 + 3*x + 3*5 = x^2 + 5x + 3x + 15 = x^2 + 8x + 15
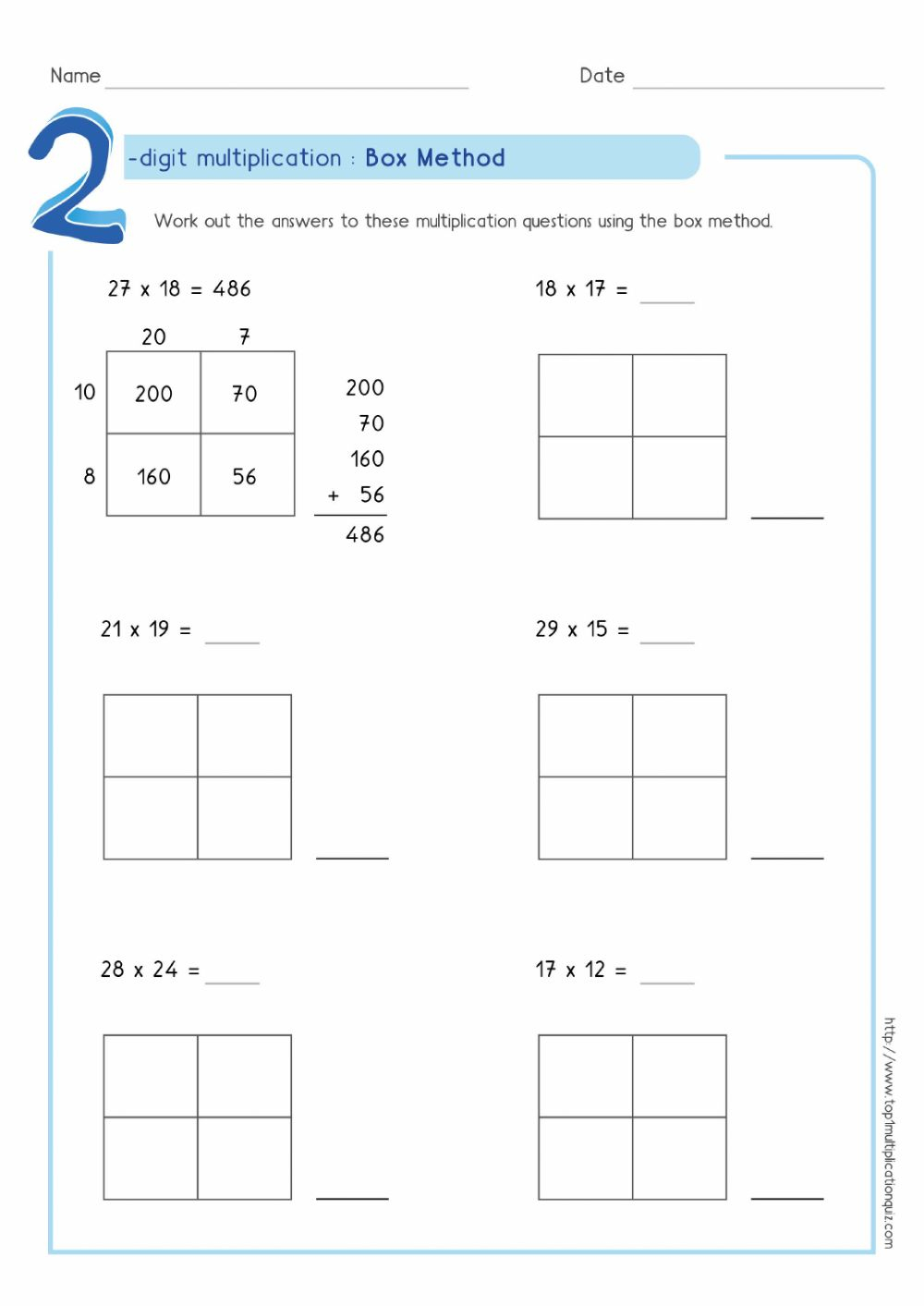
Using the Box Method: A Visual Approach
The box method is a visual approach to multiplying polynomials. It involves creating a table or grid to organize the terms and coefficients.
Example:
Multiply x^2 + 2x + 1 and x + 3 using the box method.
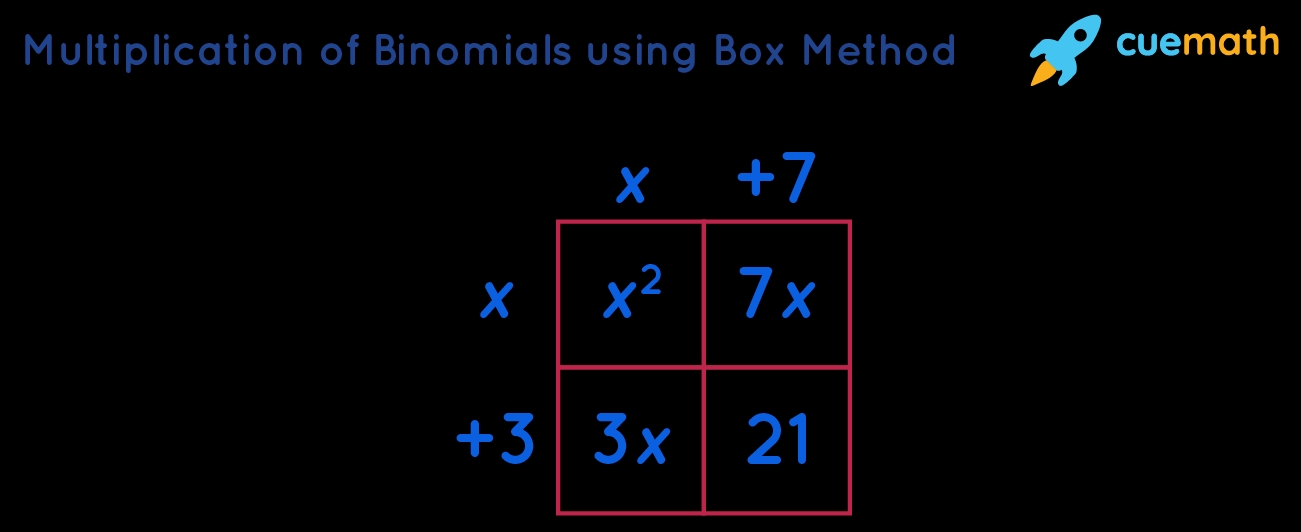
| x | 3 | |
|---|---|---|
| x^2 | x^3 | 3x^2 |
| 2x | 2x^2 | 6x |
| 1 | x | 3 |
(x^2 + 2x + 1)(x + 3) = x^3 + 3x^2 + 2x^2 + 6x + x + 3 = x^3 + 5x^2 + 7x + 3
Multiplying Polynomials with Multiple Terms
When multiplying polynomials with multiple terms, it’s essential to use the distributive property and combine like terms.
Example:
Multiply x^2 + 2x + 1 and x^2 + 3x - 2.
(x^2 + 2x + 1)(x^2 + 3x - 2) = x^2(x^2 + 3x - 2) + 2x(x^2 + 3x - 2) + 1(x^2 + 3x - 2) = x^4 + 3x^3 - 2x^2 + 2x^3 + 6x^2 - 4x + x^2 + 3x - 2 = x^4 + 5x^3 + 5x^2 - x - 2
Using Algebra Tiles: A Hands-On Approach
Algebra tiles are a hands-on tool for visualizing and multiplying polynomials. They can help you understand the concept of combining like terms and distributing coefficients.
Example:
Multiply x^2 + 2x + 1 and x + 3 using algebra tiles.
(x^2 + 2x + 1)(x + 3) = x^3 + 3x^2 + 2x^2 + 6x + x + 3
By arranging the tiles, you can see the terms and coefficients being combined.
What is the distributive property in algebra?
+The distributive property is a fundamental concept in algebra that allows you to expand a product of two binomials. It states that for any real numbers a, b, and c: a(b + c) = ab + ac.
What is the FOIL method in algebra?
+The FOIL method is a handy shortcut for multiplying two binomials. FOIL stands for First, Outer, Inner, Last, which refers to the order in which you multiply the terms.
How do I multiply polynomials with multiple terms?
+When multiplying polynomials with multiple terms, use the distributive property and combine like terms. Make sure to distribute each term in one polynomial to each term in the other polynomial.
In conclusion, multiplying polynomials can be a challenging task, but with practice and the right techniques, you can master it. By understanding the distributive property, using the FOIL method, and applying other techniques, you can simplify complex polynomial multiplication problems with ease.
Related Terms:
- Multiplying Polynomials worksheet answers
- Multiplying Polynomials Worksheet Algebra 2
- Dividing Polynomials Worksheet PDF