5 Ways to Solve Two Step Equation Worksheets
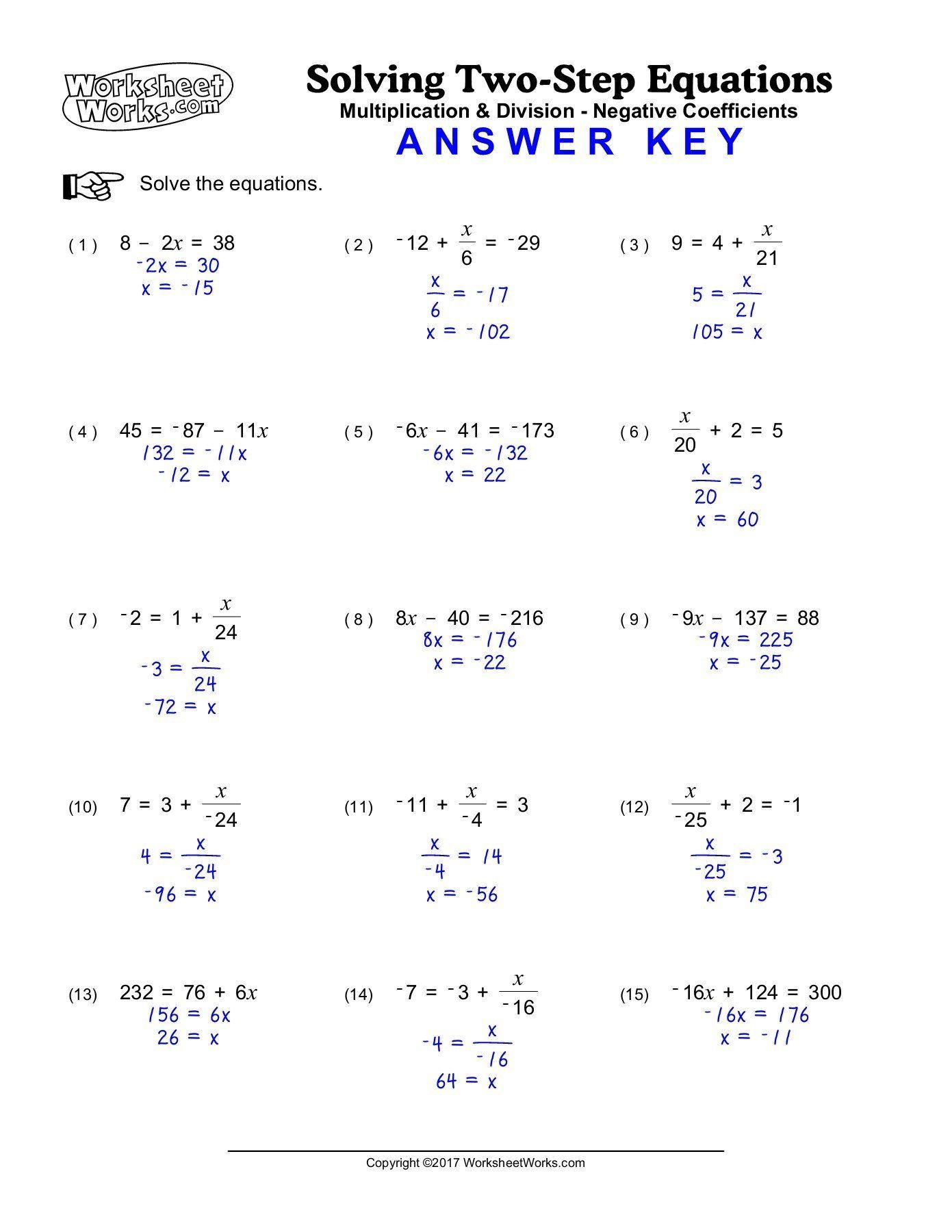
Unlocking the Secrets of Two-Step Equation Worksheets
Are you struggling to solve two-step equation worksheets? Do you find yourself getting stuck on the same problems over and over again? Don’t worry, you’re not alone! Two-step equations can be challenging, but with the right strategies and techniques, you can master them in no time. In this article, we’ll explore five ways to solve two-step equation worksheets, along with tips and tricks to help you succeed.
Method 1: The Order of Operations
When solving two-step equations, it’s essential to follow the order of operations (PEMDAS):
- Parentheses: Evaluate expressions inside parentheses first.
- Exponents: Evaluate any exponential expressions next (e.g., 2^3).
- Multiplication and Division: Evaluate any multiplication and division operations from left to right.
- Addition and Subtraction: Finally, evaluate any addition and subtraction operations from left to right.
By following the order of operations, you can ensure that you’re solving the equation correctly.
💡 Note: Make sure to evaluate expressions inside parentheses first, as this can affect the rest of the equation.
Method 2: Using Inverse Operations
Two-step equations often involve inverse operations, such as addition and subtraction or multiplication and division. To solve these equations, you need to use the inverse operation to isolate the variable.
For example, consider the equation:
2x + 5 = 11
To solve for x, you need to use the inverse operation of addition, which is subtraction. Subtract 5 from both sides of the equation to get:
2x = 11 - 5 2x = 6
Next, use the inverse operation of multiplication, which is division, to solve for x:
x = 6 ÷ 2 x = 3
Method 3: Using the Balancing Method
The balancing method involves adding, subtracting, multiplying, or dividing both sides of the equation by the same value to keep the equation balanced.
For example, consider the equation:
x - 3 = 7
To solve for x, you can add 3 to both sides of the equation to get:
x = 7 + 3 x = 10
📝 Note: Make sure to add or subtract the same value from both sides of the equation to keep it balanced.
Method 4: Using the Distributive Property
The distributive property states that a(b + c) = ab + ac. You can use this property to solve two-step equations involving parentheses.
For example, consider the equation:
2(x + 3) = 12
Use the distributive property to expand the equation:
2x + 6 = 12
Next, subtract 6 from both sides of the equation to get:
2x = 12 - 6 2x = 6
Finally, divide both sides of the equation by 2 to solve for x:
x = 6 ÷ 2 x = 3
Method 5: Checking Your Work
Finally, it’s essential to check your work when solving two-step equations. Plug the value of x back into the original equation to make sure it’s true.
For example, consider the equation:
x + 2 = 9
Solve for x:
x = 9 - 2 x = 7
Plug x back into the original equation to check:
7 + 2 = 9
If the equation is true, then you’ve solved it correctly!
Table: Common Two-Step Equation Formulas
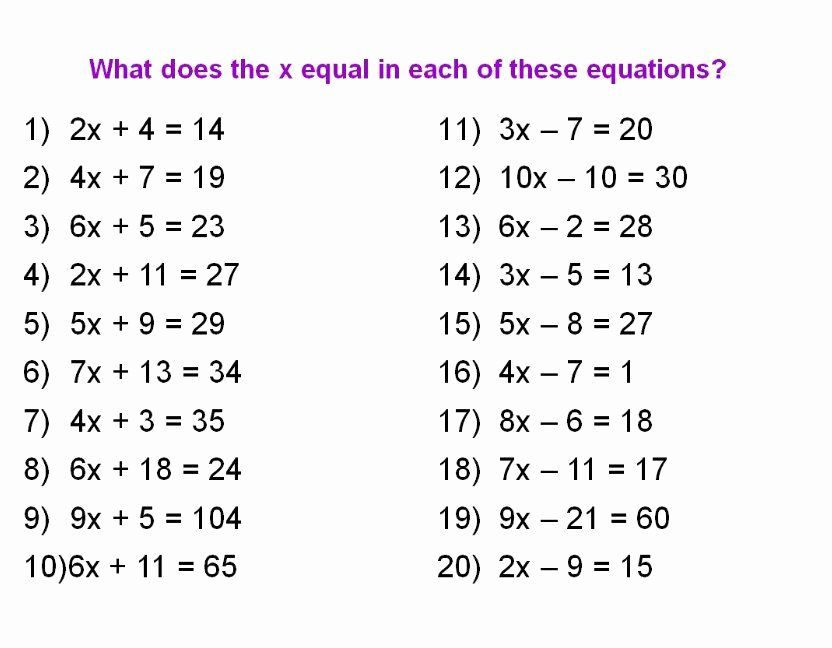
| Formula | Example | Solution |
|---|---|---|
| x + a = b | x + 2 = 7 | x = 7 - 2 |
| x - a = b | x - 3 = 4 | x = 4 + 3 |
| ax + b = c | 2x + 5 = 11 | x = (11 - 5) ÷ 2 |
| x/a = b | x/2 = 3 | x = 3 × 2 |
Now that you’ve learned five ways to solve two-step equation worksheets, it’s time to put your skills to the test! Practice solving different types of two-step equations, and don’t be afraid to ask for help if you get stuck.
What is the order of operations for solving two-step equations?
+The order of operations is: Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right).
What is the inverse operation of addition?
+The inverse operation of addition is subtraction.
What is the distributive property?
+The distributive property states that a(b + c) = ab + ac.
By mastering these five methods, you’ll become a pro at solving two-step equation worksheets in no time! Remember to practice regularly and check your work to ensure accuracy. Happy solving!