Solving Two Step Equations Made Easy

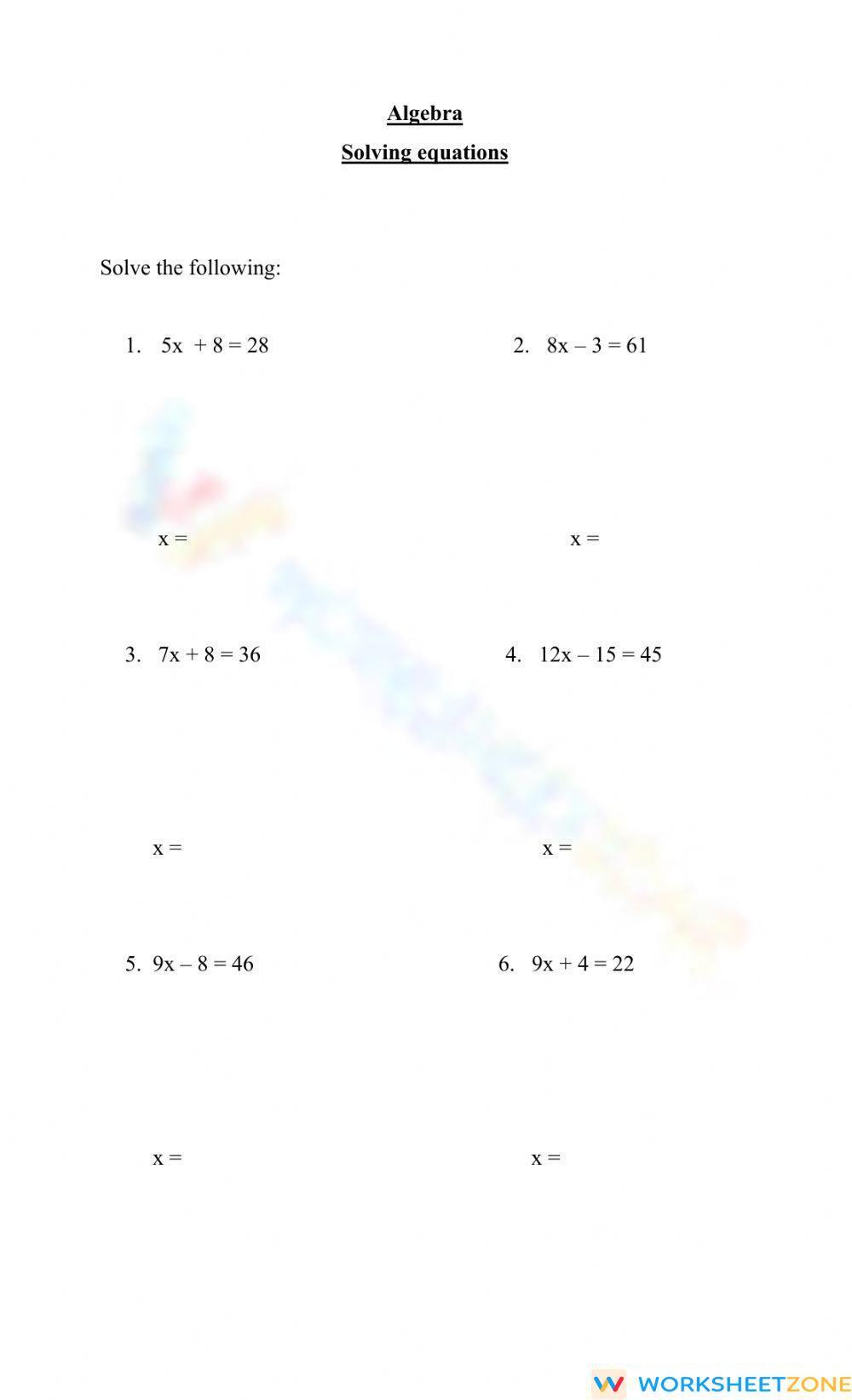
Breaking Down Two-Step Equations: A Comprehensive Guide

When it comes to solving equations, many students find two-step equations to be particularly challenging. However, with a solid understanding of the concepts and a step-by-step approach, these equations can be solved with ease. In this article, we will delve into the world of two-step equations, exploring what they are, how to solve them, and providing examples to help solidify your understanding.

What are Two-Step Equations?

A two-step equation is a linear equation that requires two operations to solve. These equations typically involve a combination of addition, subtraction, multiplication, and division. The goal is to isolate the variable, usually represented by a letter, on one side of the equation.

How to Solve Two-Step Equations

Solving two-step equations involves two main steps (hence the name!). The first step is to simplify the equation by performing one operation, and the second step is to isolate the variable by performing another operation.

Step 1: Simplify the Equation

Begin by evaluating any expressions inside parentheses, if present. Then, look for any constants that can be added or subtracted from both sides of the equation. This will help simplify the equation.

Step 2: Isolate the Variable

Once the equation is simplified, focus on isolating the variable. If the variable is multiplied or divided by a constant, perform the inverse operation to get the variable alone.

Examples of Two-Step Equations

Let’s dive into some examples to illustrate the process:

Example 1: 2x + 5 = 11

To solve this equation, we need to isolate the variable x.

Step 1: Subtract 5 from both sides of the equation. 2x = 11 - 5 2x = 6

Step 2: Divide both sides of the equation by 2. x = 6 ÷ 2 x = 3

Example 2: x/4 - 2 = 7

In this example, we need to isolate the variable x.

Step 1: Add 2 to both sides of the equation. x/4 = 7 + 2 x/4 = 9

Step 2: Multiply both sides of the equation by 4. x = 9 × 4 x = 36

Tips and Tricks for Solving Two-Step Equations

• Always read the equation carefully and identify the operations involved.
• Simplify the equation by performing any necessary calculations inside parentheses.
• Look for constants that can be added or subtracted from both sides of the equation.
• When isolating the variable, perform the inverse operation to get the variable alone.
• Check your solution by plugging it back into the original equation.

📝 Note: When solving two-step equations, it's essential to perform the operations in the correct order. Failure to do so can result in incorrect solutions.

Common Mistakes to Avoid

• Not simplifying the equation before isolating the variable.
• Performing operations out of order.
• Not checking the solution by plugging it back into the original equation.

Real-World Applications of Two-Step Equations

Two-step equations have numerous real-world applications, including:

• Science: Solving equations to calculate quantities such as velocity, acceleration, and force.
• Finance: Balancing budgets and calculating interest rates.
• Engineering: Designing systems and structures that require precise calculations.

Conclusion

Solving two-step equations requires a solid understanding of mathematical operations and a step-by-step approach. By following the tips and tricks outlined in this article, you’ll become proficient in solving these equations with ease. Remember to always simplify the equation, isolate the variable, and check your solution. With practice and patience, you’ll master two-step equations in no time.