Fraction Review Worksheet Made Easy
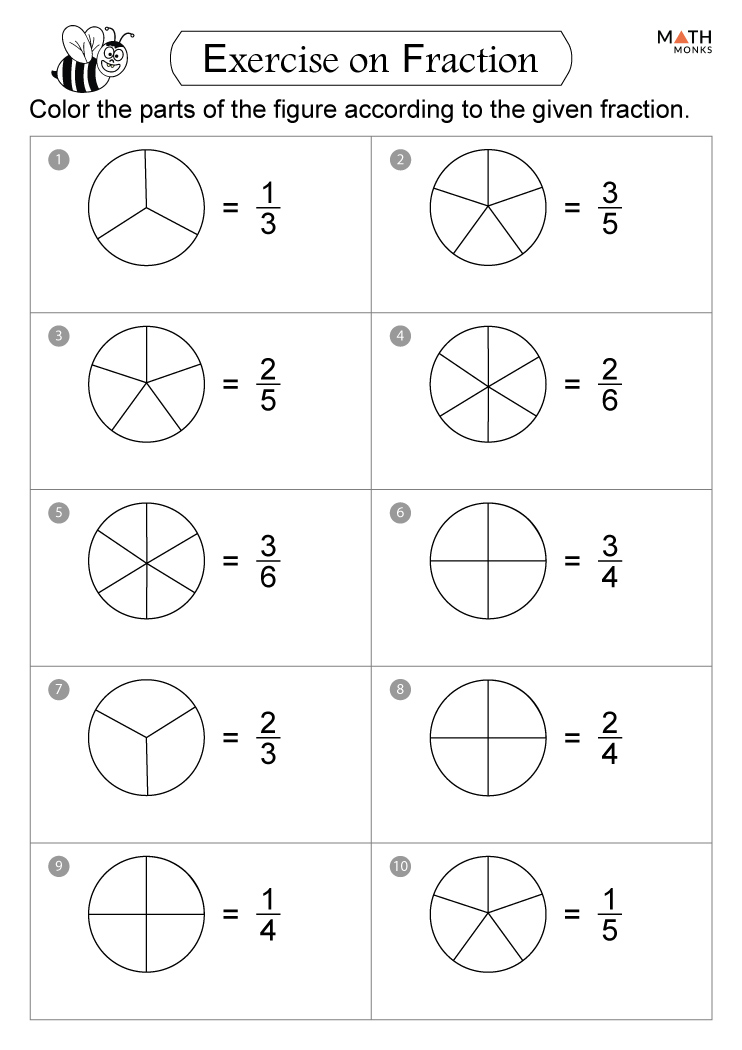
Introduction to Fractions
Fractions are a fundamental concept in mathematics that can seem intimidating at first, but with practice and patience, anyone can master them. In this review worksheet, we will cover the basics of fractions, including what they are, how to read them, and how to perform operations with them.
What are Fractions?
A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into.
For example, the fraction 1⁄2 represents one part out of a total of two parts.
Types of Fractions
There are three main types of fractions:
- Proper Fractions: These are fractions where the numerator is less than the denominator. Examples: 1⁄2, 3⁄4, 2⁄3.
- Improper Fractions: These are fractions where the numerator is greater than or equal to the denominator. Examples: 3⁄2, 5⁄4, 7⁄3.
- Mixed Fractions: These are fractions that have a whole number part and a fractional part. Examples: 2 1⁄2, 3 3⁄4, 1 1⁄3.
Reading Fractions
To read a fraction, we need to know what the numerator and denominator are. For example, the fraction 3⁄4 is read as “three-quarters”.
Comparing Fractions
To compare fractions, we need to make sure they have the same denominator. If they don’t, we can convert them to have the same denominator by multiplying the numerator and denominator by the same number.
For example, to compare 1⁄2 and 2⁄3, we can convert them to have the same denominator of 6:
1⁄2 = 3⁄6 2⁄3 = 4⁄6
Now we can see that 2⁄3 is greater than 1⁄2.
Adding and Subtracting Fractions
To add and subtract fractions, we need to make sure they have the same denominator. If they don’t, we can convert them to have the same denominator by multiplying the numerator and denominator by the same number.
For example, to add 1⁄2 and 1⁄3, we can convert them to have the same denominator of 6:
1⁄2 = 3⁄6 1⁄3 = 2⁄6
Now we can add them:
3⁄6 + 2⁄6 = 5⁄6
Multiplying and Dividing Fractions
To multiply fractions, we multiply the numerators and denominators separately:
1⁄2 x 3⁄4 = 3⁄8
To divide fractions, we invert the second fraction (i.e., flip the numerator and denominator) and multiply:
1⁄2 ÷ 3⁄4 = 1⁄2 x 4⁄3 = 4⁄6
Reducing Fractions
A fraction is in its simplest form when the numerator and denominator have no common factors. To reduce a fraction, we divide both the numerator and denominator by their greatest common factor (GCF).
For example, the fraction 6⁄8 can be reduced by dividing both the numerator and denominator by 2:
6 ÷ 2 = 3 8 ÷ 2 = 4
So, the reduced fraction is 3⁄4.
Practice Exercises
Now it’s time to practice! Try these exercises to test your understanding of fractions:
- What is the numerator and denominator of the fraction 2⁄3?
- What is the simplest form of the fraction 4⁄8?
- Add 1⁄2 and 1⁄4.
- Multiply 2⁄3 and 3⁄4.
- Divide 1⁄2 by 3⁄4.
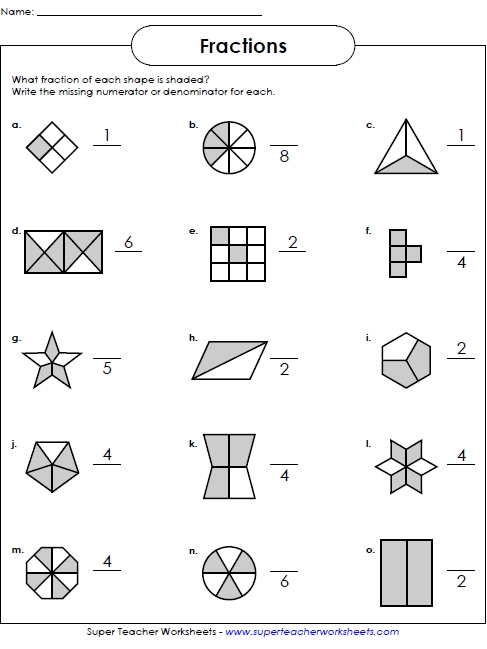
| Fraction | Numerator | Denominator |
|---|---|---|
| 1/2 | 1 | 2 |
| 2/3 | 2 | 3 |
| 3/4 | 3 | 4 |
📝 Note: Make sure to check your answers to the practice exercises to ensure you understand the concepts.
Conclusion
Fractions are an essential part of mathematics, and understanding them can help you solve a wide range of problems. With practice and patience, you can master the basics of fractions and become more confident in your math skills.
In this review worksheet, we covered the basics of fractions, including what they are, how to read them, and how to perform operations with them. We also practiced reducing fractions and solving problems.
I hope this review worksheet has been helpful in refreshing your knowledge of fractions. Remember to keep practicing, and you’ll become a master of fractions in no time!
What is a fraction?
+A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number).
How do I add fractions?
+To add fractions, we need to make sure they have the same denominator. If they don’t, we can convert them to have the same denominator by multiplying the numerator and denominator by the same number.
How do I reduce a fraction?
+A fraction is in its simplest form when the numerator and denominator have no common factors. To reduce a fraction, we divide both the numerator and denominator by their greatest common factor (GCF).