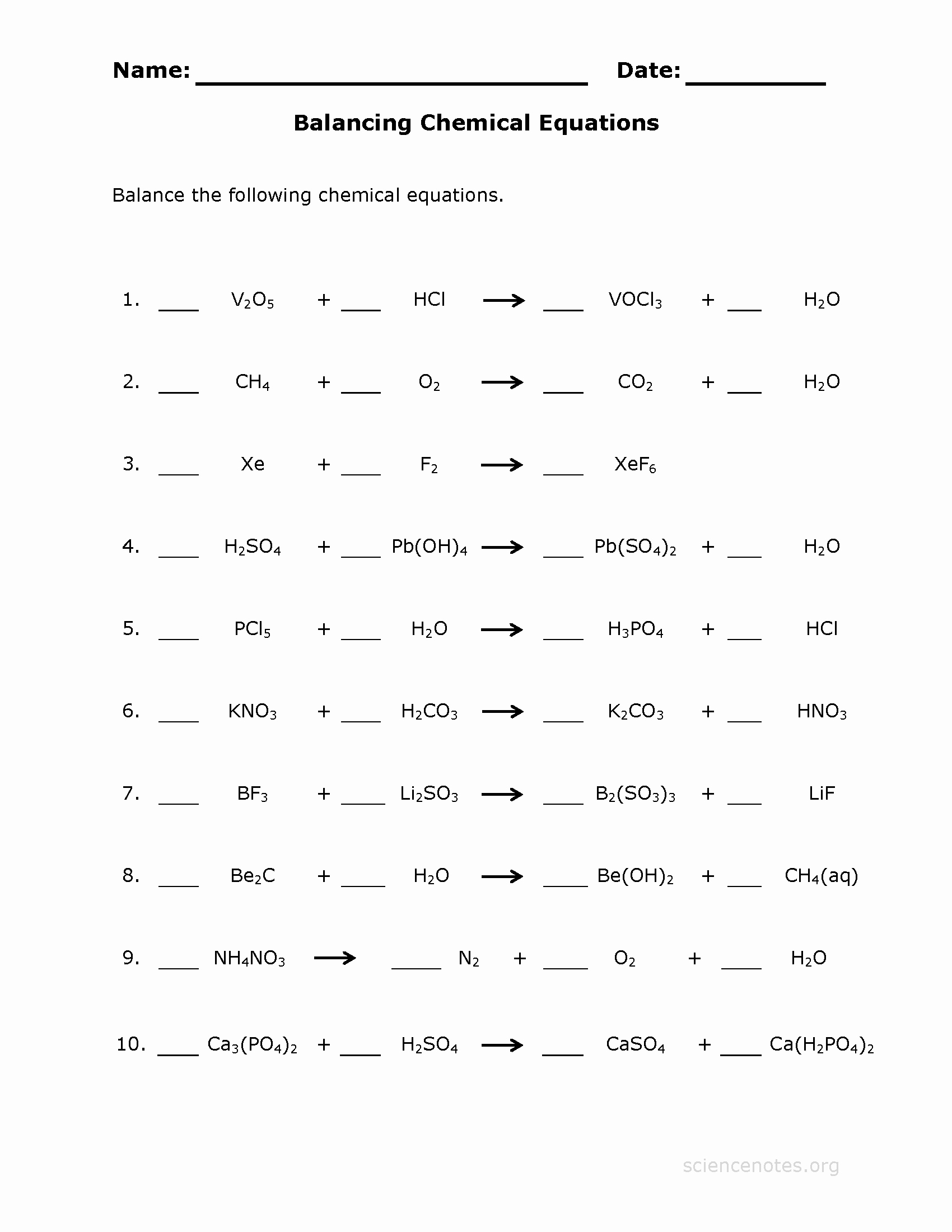
Find the Missing Angle Worksheet Solutions

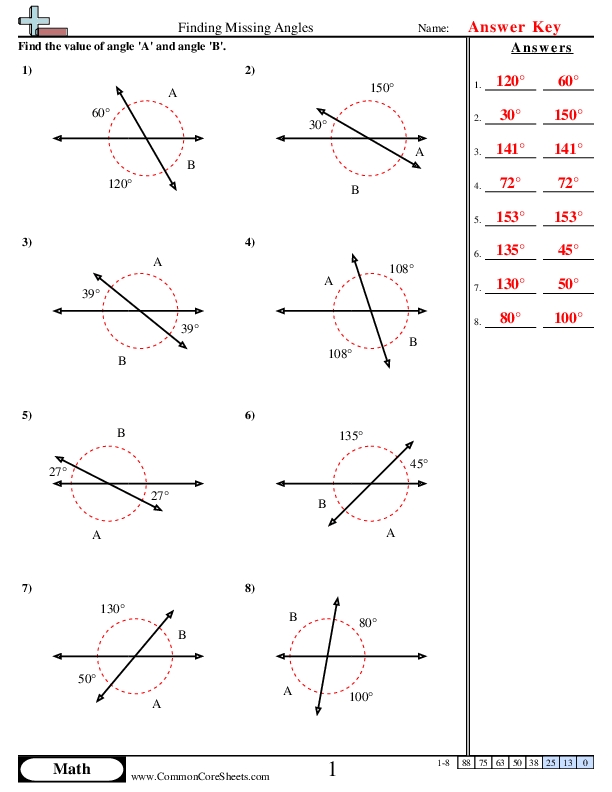
Find the Missing Angle Worksheet Solutions

Are you struggling to find the missing angles in triangles and quadrilaterals? Look no further! In this comprehensive guide, we will provide step-by-step solutions to finding missing angles in various geometric shapes. Our solutions will cover a range of problems, from basic to advanced, and include examples, explanations, and tips to help you master this essential math skill.

Angles in Triangles

When working with triangles, it’s essential to remember that the sum of the interior angles is always 180 degrees. This fundamental property can be used to find missing angles in triangles.

Example 1: Find the missing angle in the triangle below.

Triangle with two angles: 30° and 60°

Solution: Since the sum of the interior angles in a triangle is 180 degrees, we can use the following equation to find the missing angle:

30° + 60° + x = 180°

Combine like terms:

90° + x = 180°

Subtract 90° from both sides:

x = 90°

Therefore, the missing angle is 90°.

Angles in Quadrilaterals

Quadrilaterals, including rectangles, squares, and trapezoids, have interior angles that add up to 360 degrees. This property can be used to find missing angles in quadrilaterals.

Example 2: Find the missing angle in the quadrilateral below.

Quadrilateral with three angles: 90°, 120°, and 60°

Solution: Since the sum of the interior angles in a quadrilateral is 360 degrees, we can use the following equation to find the missing angle:

90° + 120° + 60° + x = 360°

Combine like terms:

270° + x = 360°

Subtract 270° from both sides:

x = 90°

Therefore, the missing angle is 90°.

Using Angle Relationships

In addition to using the sum of interior angles, you can also use angle relationships to find missing angles.

Example 3: Find the missing angle in the triangle below.

Triangle with two angles: 30° and x°

The triangle also has a right angle (90°).

Solution: Since the triangle has a right angle, we know that the sum of the other two angles is 90°.

30° + x = 90°

Subtract 30° from both sides:

x = 60°

Therefore, the missing angle is 60°.

Example 4: Find the missing angle in the quadrilateral below.

Quadrilateral with three angles: 90°, 120°, and x°

The quadrilateral also has a pair of opposite angles that are supplementary (add up to 180°).

Solution: Since the pair of opposite angles is supplementary, we can set up the following equation:

90° + x = 180°

Subtract 90° from both sides:

x = 90°

Therefore, the missing angle is 90°.

Additional Tips and Tricks

When finding missing angles, it’s essential to:

• Use the sum of interior angles in triangles (180 degrees) and quadrilaterals (360 degrees).
• Use angle relationships, such as supplementary and complementary angles.
• Read the problem carefully and identify any additional information, such as right angles or pairs of opposite angles.

Common Mistakes to Avoid

• Forgetting to use the sum of interior angles.
• Not considering angle relationships.
• Not reading the problem carefully.

🤔 Note: When working with angles, it's essential to be precise and careful. Double-check your calculations and make sure you're using the correct formulas and relationships.

Conclusion

Finding missing angles in triangles and quadrilaterals is a fundamental math skill that requires attention to detail and a solid understanding of geometric properties. By using the sum of interior angles, angle relationships, and additional tips and tricks, you can become proficient in finding missing angles and tackle a range of math problems with confidence.

FAQ