Simplifying Radical Expressions Made Easy Worksheet Answers
Mastering Simplifying Radical Expressions: A Step-by-Step Guide
Radical expressions can be intimidating, especially when it comes to simplifying them. However, with a solid understanding of the rules and principles, you can easily simplify even the most complex radical expressions. In this article, we will provide a comprehensive guide on how to simplify radical expressions, along with a worksheet and answers to help you practice and reinforce your understanding.
Understanding Radical Expressions
A radical expression is an algebraic expression that contains a square root, cube root, or other root symbol. The most common type of radical expression is the square root expression, denoted by the symbol √. Radical expressions can be simplified by factoring out perfect squares or perfect cubes from the radicand.
Rules for Simplifying Radical Expressions
Here are the basic rules for simplifying radical expressions:
- Rule 1: If the radicand contains a perfect square, you can factor out the perfect square and simplify the expression.
- Rule 2: If the radicand contains a perfect cube, you can factor out the perfect cube and simplify the expression.
- Rule 3: If the radicand contains a product of two or more terms, you can factor out the greatest common factor (GCF) of the terms.
Step-by-Step Simplification Process
Here’s a step-by-step guide on how to simplify radical expressions:
- Step 1: Identify the radicand and determine if it contains any perfect squares or perfect cubes.
- Step 2: Factor out any perfect squares or perfect cubes from the radicand.
- Step 3: Simplify the expression by combining any like terms.
- Step 4: If the expression contains any remaining radicals, repeat the process until the expression is fully simplified.
Worksheet: Simplifying Radical Expressions
Try simplifying the following radical expressions:
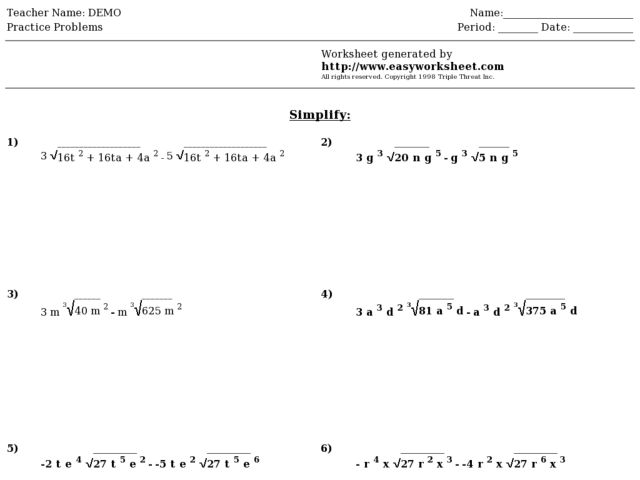
| Expression | Simplified Expression |
|---|---|
| √(16x^2) | |
| √(25x^4) | |
| √(36x^6) | |
| √(9x^3) | |
| √(4x^2 + 9) |
Answers
Here are the answers to the worksheet:
| Expression | Simplified Expression |
|---|---|
| √(16x^2) | 4x |
| √(25x^4) | 5x^2 |
| √(36x^6) | 6x^3 |
| √(9x^3) | 3x√x |
| √(4x^2 + 9) | No simplification possible |
📝 Note: In the last expression, √(4x^2 + 9), there are no perfect squares or perfect cubes that can be factored out, so the expression cannot be simplified further.
Additional Tips and Tricks
- Always look for perfect squares or perfect cubes in the radicand before simplifying.
- Factor out the greatest common factor (GCF) of the terms in the radicand.
- Simplify any remaining radicals by combining like terms.
Mastery of Simplifying Radical Expressions
With practice and patience, you can master the art of simplifying radical expressions. Remember to always follow the rules and principles outlined in this guide, and you’ll be well on your way to becoming a pro at simplifying radical expressions.
What is the purpose of simplifying radical expressions?
+Simplifying radical expressions helps to make complex expressions more manageable and easier to work with. It also helps to reveal the underlying structure of the expression and make it easier to perform algebraic operations.
How do I know if an expression can be simplified?
+Look for perfect squares or perfect cubes in the radicand, and check if the expression can be factored. If the expression meets these conditions, it can be simplified.
What is the difference between a perfect square and a perfect cube?
+A perfect square is an expression that can be written in the form x^2, where x is an integer. A perfect cube is an expression that can be written in the form x^3, where x is an integer.