Mastering Radicals: Adding and Subtracting Made Easy
Mastering Radicals: A Comprehensive Guide to Adding and Subtracting
Radicals, also known as roots, are a fundamental concept in mathematics, and mastering them is essential for success in various mathematical operations. In this article, we will delve into the world of radicals and explore how to add and subtract them with ease.
Understanding Radicals
Before we dive into the process of adding and subtracting radicals, it’s essential to understand the basics. A radical is a symbol used to represent a root of a number. The most common radical is the square root, which is represented by the symbol √. Other types of radicals include cube roots (∛), fourth roots (∜), and so on.
Like Radicals
To add or subtract radicals, we need to work with like radicals. Like radicals are radicals that have the same index (the number outside the radical symbol) and the same radicand (the number inside the radical symbol). For example:
- 2√3 and 3√3 are like radicals
- 2√5 and 3√7 are not like radicals
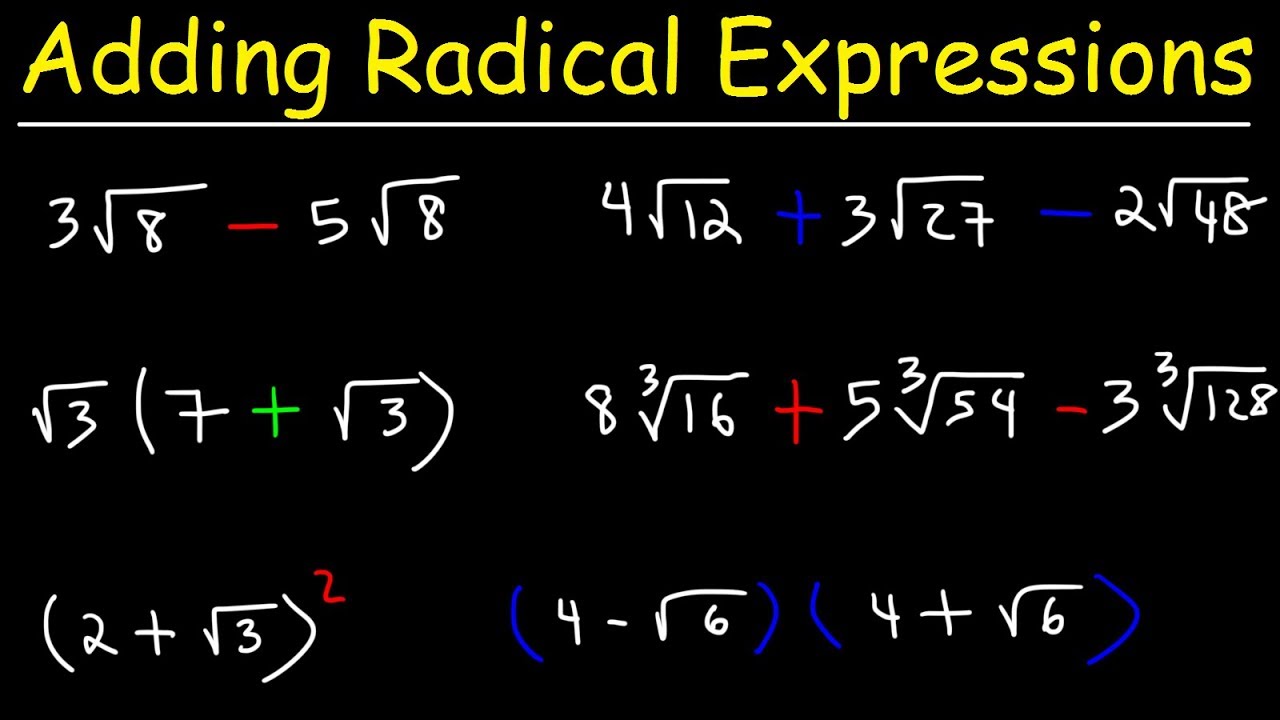
Adding Radicals
Adding radicals is a straightforward process when working with like radicals. Here are the steps:
- Check if the radicals are like radicals. If they are not, you cannot add them.
- Combine the coefficients (the numbers outside the radical symbol).
- Keep the same radicand and index.
Example:
- 2√3 + 3√3 = (2 + 3)√3 = 5√3
Subtracting Radicals
Subtracting radicals is similar to adding radicals. Here are the steps:
- Check if the radicals are like radicals. If they are not, you cannot subtract them.
- Combine the coefficients (the numbers outside the radical symbol).
- Keep the same radicand and index.
Example:
- 5√2 - 2√2 = (5 - 2)√2 = 3√2
Adding and Subtracting Radicals with Different Indexes
When working with radicals that have different indexes, we need to rationalize the denominator. Rationalizing the denominator involves multiplying the numerator and denominator by a radical that will eliminate the radical in the denominator.
Example:
- 2√3 + 3√5 =?
To add these radicals, we need to rationalize the denominator. We can do this by multiplying both the numerator and denominator by √15:
- 2√3 + 3√5 = 2√3 × √15 / √15 + 3√5 × √15 / √15
- = 2√45 / √15 + 3√75 / √15
- = (2√45 + 3√75) / √15
Table of Common Radical Operations
| Operation | Example | Result |
|---|---|---|
| Adding like radicals | 2√3 + 3√3 | (2 + 3)√3 = 5√3 |
| Subtracting like radicals | 5√2 - 2√2 | (5 - 2)√2 = 3√2 |
| Rationalizing the denominator | 2√3 + 3√5 | (2√45 + 3√75) / √15 |
🤔 Note: When working with radicals, it's essential to check if the radicals are like radicals before adding or subtracting.
Conclusion
Mastering radicals is a crucial skill in mathematics, and adding and subtracting radicals is a fundamental operation. By understanding the basics of radicals and following the steps outlined in this article, you can become proficient in adding and subtracting radicals with ease. Remember to always check if the radicals are like radicals and to rationalize the denominator when working with radicals that have different indexes.
What is a radical?
+A radical is a symbol used to represent a root of a number. The most common radical is the square root, which is represented by the symbol √.
How do I add radicals?
+To add radicals, check if the radicals are like radicals. If they are, combine the coefficients (the numbers outside the radical symbol) and keep the same radicand and index.
How do I subtract radicals?
+To subtract radicals, check if the radicals are like radicals. If they are, combine the coefficients (the numbers outside the radical symbol) and keep the same radicand and index.