5 Ways to Master Similar Figures
Unlocking the Secrets of Similar Figures in Geometry
Similar figures are a fundamental concept in geometry, and mastering them can help you solve a wide range of problems with ease. In this article, we will explore five ways to master similar figures, including understanding the definition, identifying similar triangles, using proportions, applying similarity theorems, and practicing with real-world examples.
1. Understanding the Definition of Similar Figures
Before we dive into the ways to master similar figures, it’s essential to understand what they are. Similar figures are two-dimensional shapes that have the same shape but not necessarily the same size. In other words, they have the same angles and proportional side lengths. For example, two triangles are similar if their corresponding angles are equal and their corresponding sides are proportional.
🔍 Note: Similar figures can be similar in two ways: directly similar or indirectly similar. Directly similar figures have the same orientation, while indirectly similar figures have a different orientation.
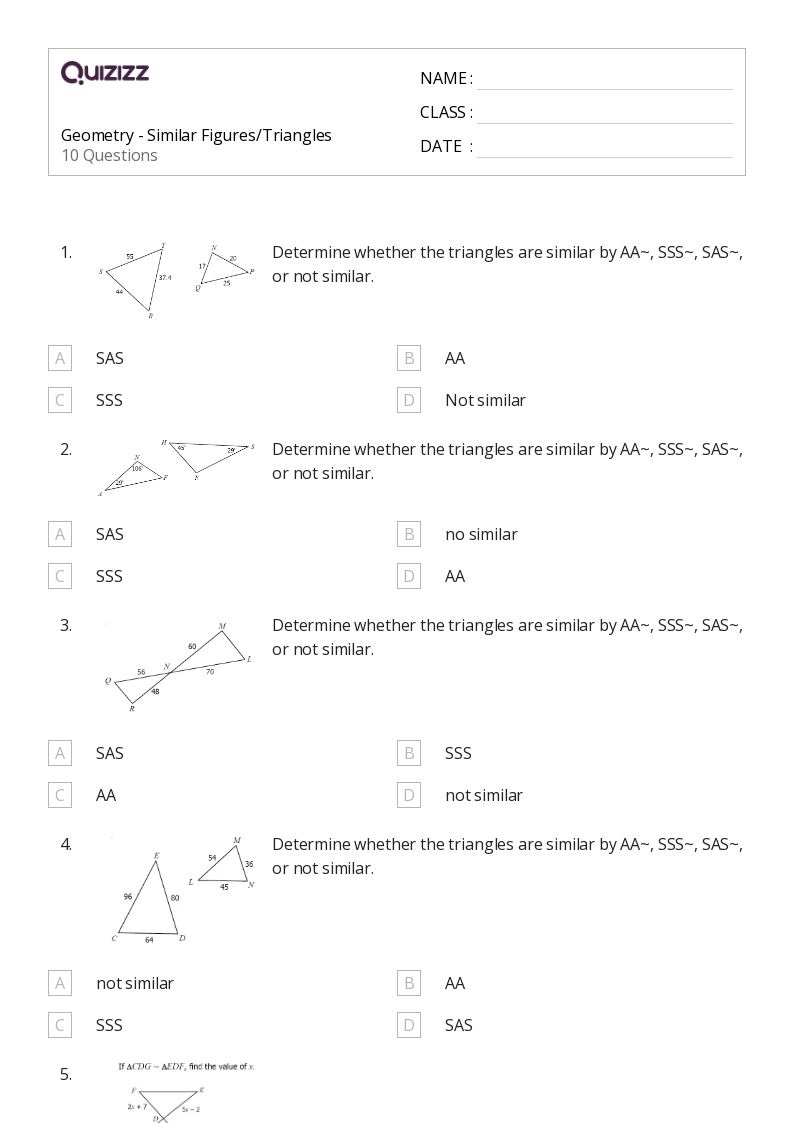
2. Identifying Similar Triangles
Similar triangles are a special type of similar figure. To identify similar triangles, you need to look for the following characteristics:
- AA Similarity: If two triangles have two pairs of congruent angles, then they are similar.
- SSS Similarity: If two triangles have three pairs of congruent sides, then they are similar.
- SAS Similarity: If two triangles have two pairs of congruent sides and the included angle is congruent, then they are similar.
For example, consider two triangles ΔABC and ΔDEF. If ∠A = ∠D, ∠B = ∠E, and ∠C = ∠F, then the triangles are similar by AA similarity.
3. Using Proportions to Solve Similar Figure Problems
One of the most powerful tools for solving similar figure problems is proportions. A proportion is a statement that two ratios are equal. In similar figures, corresponding sides are proportional, which means that the ratio of the lengths of corresponding sides is equal.
For example, consider two similar triangles ΔABC and ΔDEF. If AB = 3x and DE = 4x, then the ratio of AB to DE is 3:4. This proportion can be used to solve problems involving similar triangles.
4. Applying Similarity Theorems
There are several similarity theorems that can help you solve problems involving similar figures. Some of the most common similarity theorems include:
- SAS Similarity Theorem: If two triangles have two pairs of congruent sides and the included angle is congruent, then they are similar.
- SSS Similarity Theorem: If two triangles have three pairs of congruent sides, then they are similar.
- AA Similarity Theorem: If two triangles have two pairs of congruent angles, then they are similar.
For example, consider two triangles ΔABC and ΔDEF. If AB = 3x, BC = 4x, and AC = 5x, and DE = 6x, EF = 8x, and DF = 10x, then the triangles are similar by SSS similarity.
5. Practicing with Real-World Examples
The best way to master similar figures is to practice with real-world examples. Here are a few examples to get you started:
| Example | Description |
|---|---|
| 1 | Two similar triangles have side lengths 3x, 4x, and 5x. Find the ratio of the corresponding sides. |
| 2 | A photographer takes a picture of a building with a similar figure in the foreground. If the building is 100 feet tall and the similar figure is 20 feet tall, what is the scale factor? |
| 3 | Two similar triangles are used to design a roof. If one triangle has side lengths 6 feet, 8 feet, and 10 feet, and the other triangle has side lengths 3 feet, 4 feet, and 5 feet, are the triangles similar? |
As you can see, similar figures are an essential concept in geometry, and mastering them can help you solve a wide range of problems. By understanding the definition, identifying similar triangles, using proportions, applying similarity theorems, and practicing with real-world examples, you can become a master of similar figures.
What is the difference between similar figures and congruent figures?
+Congruent figures have the same size and shape, while similar figures have the same shape but not necessarily the same size.
How do I determine if two triangles are similar?
+You can use the AA, SSS, or SAS similarity theorems to determine if two triangles are similar.
What is the scale factor of two similar figures?
+The scale factor is the ratio of the corresponding sides of the two similar figures.