Solve Quadratic Word Problems in 5 Easy Steps

Solving Quadratic Word Problems: A Step-by-Step Guide

Quadratic word problems can be daunting, but breaking them down into manageable steps can make all the difference. In this article, we’ll explore a 5-step approach to solving quadratic word problems with ease.

Step 1: Read and Understand the Problem

The first step in solving any word problem is to read and understand what’s being asked. Take your time to read the problem carefully, and identify the key elements:

• Variables: What are the unknowns in the problem?
• Constants: What are the given values?
• Relationships: How are the variables and constants related?

Look for keywords that indicate a quadratic relationship, such as “area,” “volume,” “distance,” or “time.”

Step 2: Translate the Problem into an Equation

Once you’ve identified the key elements, translate the problem into a quadratic equation. Use the following steps to help you:

• Identify the quadratic formula: If the problem involves a quadratic relationship, identify the formula that best represents it. For example, the area of a rectangle (A = length × width) or the volume of a cube (V = side^3).
• Assign variables: Assign variables to the unknowns in the problem. Make sure to label them clearly.
• Write the equation: Use the variables and constants to write a quadratic equation that represents the problem.

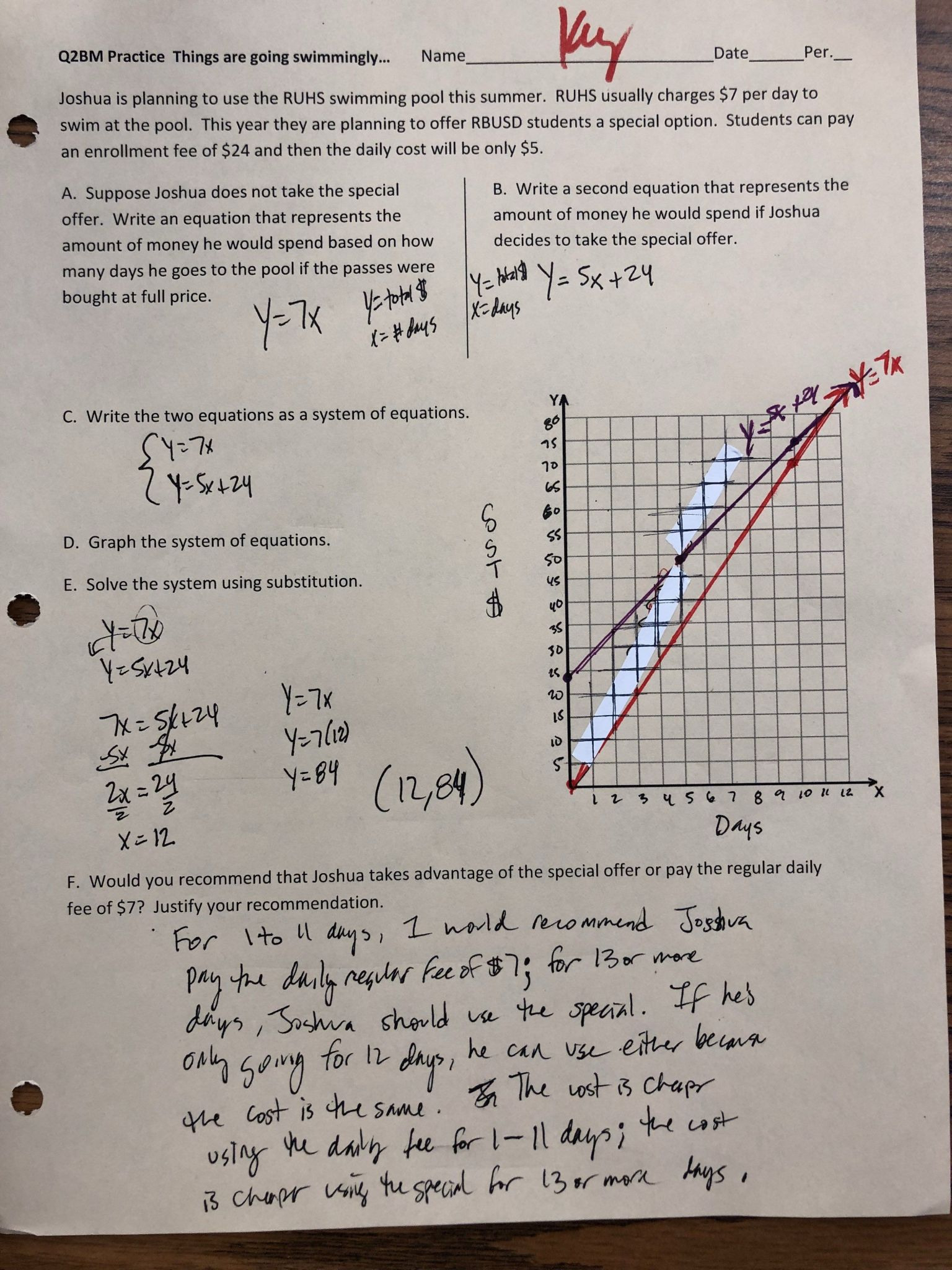
For example, let’s say we have a problem that states: “A farmer wants to enclose a rectangular garden with 100 meters of fencing. What dimensions should the garden be to maximize its area?”

We can translate this problem into the equation: A = xy, where A is the area, x is the length, and y is the width.

Step 3: Solve the Quadratic Equation

Now that we have our quadratic equation, it’s time to solve it. Use the following methods to solve the equation:

• Factoring: If the equation can be factored, use this method to find the solutions.
• Quadratic Formula: If the equation cannot be factored, use the quadratic formula: x = (-b ± √(b^2 - 4ac)) / 2a.
• Graphing: If you have a graphing calculator, use it to visualize the equation and find the solutions.

For our example problem, we can use the quadratic formula to solve for x and y:

x = (-b ± √(b^2 - 4ac)) / 2a x = (-(0) ± √((0)^2 - 4(1)(100))) / 2(1) x = (0 ± √(-400)) / 2 x = (0 ± 20i) / 2

Since we’re dealing with a real-world problem, we can ignore the imaginary solutions and focus on the real solutions.

Step 4: Interpret the Solutions

Once you’ve solved the quadratic equation, interpret the solutions in the context of the problem. Ask yourself:

• What do the solutions represent?: What do the solutions represent in the context of the problem?
• Are the solutions reasonable?: Are the solutions reasonable and make sense in the context of the problem?

For our example problem, the solutions represent the dimensions of the garden. We can see that the solutions are x = 20 and y = 5, which means the garden should be 20 meters long and 5 meters wide.

Step 5: Check the Solution

Finally, check the solution to make sure it’s correct. Use the following steps to check the solution:

• Plug the solution back into the equation: Plug the solution back into the original equation to make sure it’s true.
• Check the units: Check the units of the solution to make sure they’re correct.
• Check the reasonableness: Check the reasonableness of the solution to make sure it makes sense in the context of the problem.

For our example problem, we can plug the solution back into the equation to check:

A = xy A = (20)(5) A = 100

The solution checks out, and we can be confident that the garden should be 20 meters long and 5 meters wide to maximize its area.

👍 Note: Always check your solution to make sure it's correct and reasonable. This will help you avoid errors and ensure that your solution is accurate.

As we’ve seen, solving quadratic word problems can be challenging, but breaking them down into manageable steps can make all the difference. By following these 5 easy steps, you can solve quadratic word problems with confidence and accuracy.

Summary of key points:

• Read and understand the problem
• Translate the problem into a quadratic equation
• Solve the quadratic equation
• Interpret the solutions
• Check the solution

By following these steps, you’ll be well on your way to solving quadratic word problems with ease.

Related Terms:

• Quadratic Applications Worksheet with answers
• Quadratic area Word Problems worksheet
• Rectangle quadratic word problems