Angle Proofs Worksheet with Answers
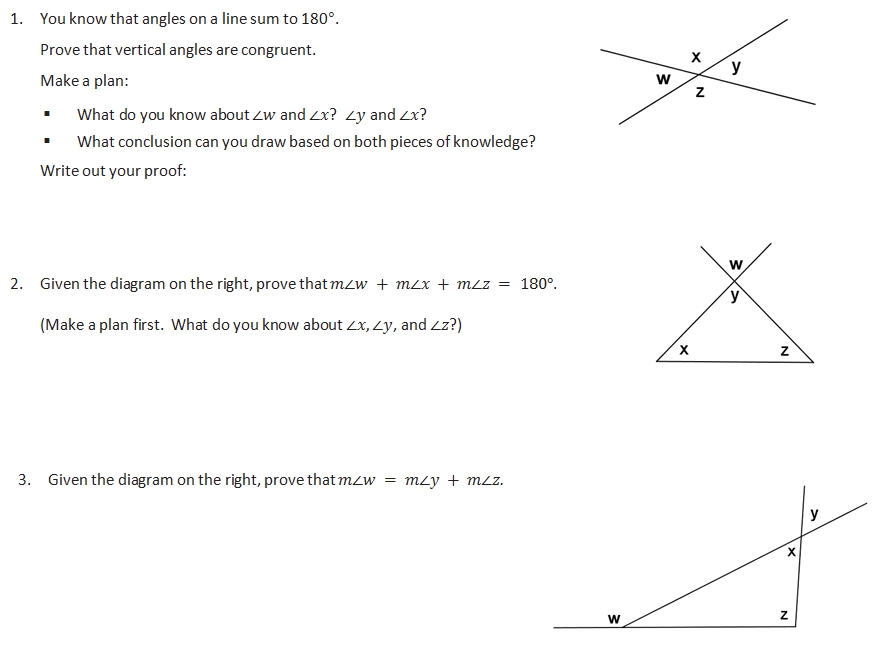
Understanding Angle Proofs: A Comprehensive Guide with Worksheet and Answers
In geometry, angle proofs are a crucial concept that helps students establish the validity of geometric statements. It involves a series of logical steps to prove that a particular statement is true. In this blog post, we will delve into the world of angle proofs, explore the different types, and provide a worksheet with answers to help students practice and reinforce their understanding.
What are Angle Proofs?
Angle proofs are a way of verifying the truth of a geometric statement by using a series of logical steps. It involves using previously proven statements, definitions, and postulates to prove a new statement. Angle proofs are used to establish the validity of various geometric concepts, such as the properties of angles, triangles, and other geometric figures.
Types of Angle Proofs
There are several types of angle proofs, including:
- Two-Column Proof: A two-column proof is a type of proof that involves two columns, one for statements and one for reasons. Each statement is accompanied by a reason that justifies it.
- Paragraph Proof: A paragraph proof is a type of proof that involves writing a series of statements in paragraph form. Each statement is justified by a reason that is written in the same paragraph.
- Flow Proof: A flow proof is a type of proof that involves using a flowchart to illustrate the steps of the proof.
Key Concepts in Angle Proofs
Some key concepts that are commonly used in angle proofs include:
- Postulates: Postulates are statements that are assumed to be true without proof. They are the foundation of geometry and are used to prove other statements.
- Theorems: Theorems are statements that have been proven to be true. They are used to prove other statements and are often used in conjunction with postulates.
- Definitions: Definitions are statements that define a particular term or concept. They are used to establish the meaning of a word or phrase and are often used in angle proofs.
- Properties of Angles: Properties of angles, such as the properties of complementary, supplementary, and corresponding angles, are often used in angle proofs.
Angle Proofs Worksheet
Here is a worksheet with 10 angle proofs for you to practice:
| Statement | Reason |
|---|---|
| 1. ∠A and ∠B are complementary. | Given |
| 2. ∠A + ∠B = 90°. | Definition of complementary angles |
| 3. ∠A = 30°. | Given |
| 4. ∠B = 60°. | Substitution property of equality |
| 5. ∠A and ∠B are supplementary. | Given |
| 6. ∠A + ∠B = 180°. | Definition of supplementary angles |
| 7. ∠A = 90°. | Given |
| 8. ∠B = 90°. | Substitution property of equality |
| 9. ∠A and ∠B are corresponding angles. | Given |
| 10. ∠A = ∠B. | Definition of corresponding angles |
Answers
Here are the answers to the worksheet:
- ∠A and ∠B are complementary because they add up to 90°.
- ∠A + ∠B = 90° because they are complementary.
- ∠A = 30° because it is given.
- ∠B = 60° because ∠A + ∠B = 90° and ∠A = 30°.
- ∠A and ∠B are supplementary because they add up to 180°.
- ∠A + ∠B = 180° because they are supplementary.
- ∠A = 90° because it is given.
- ∠B = 90° because ∠A + ∠B = 180° and ∠A = 90°.
- ∠A and ∠B are corresponding angles because they are on the same side of the transversal and are not adjacent.
- ∠A = ∠B because they are corresponding angles.
📝 Note: Make sure to read each statement carefully and use the given reasons to justify each statement.
By practicing these angle proofs, you will become more comfortable with the different types of proofs and be able to apply them to a variety of geometric concepts.
In conclusion, angle proofs are a fundamental concept in geometry that helps students establish the validity of geometric statements. By understanding the different types of proofs and key concepts, students can become proficient in writing and reading angle proofs.
What is an angle proof?
+An angle proof is a series of logical steps used to prove the validity of a geometric statement.
What are the different types of angle proofs?
+There are three main types of angle proofs: two-column proof, paragraph proof, and flow proof.
What is the purpose of an angle proof?
+The purpose of an angle proof is to establish the validity of a geometric statement by using a series of logical steps.