5 Easy Ways to Simplify Fractions
What are Fractions and Why Simplify Them?
Fractions are a way to represent part of a whole. They are written as two numbers, one on top of the other, with a line in between. The top number is called the numerator, and the bottom number is called the denominator. Fractions can be used to represent various things, such as a part of a pizza, a portion of a cake, or a section of a puzzle.
Simplifying fractions is essential to make them easier to work with and understand. Simplified fractions are more efficient and effective in various mathematical operations, such as addition, subtraction, multiplication, and division. In this blog post, we will discuss five easy ways to simplify fractions.
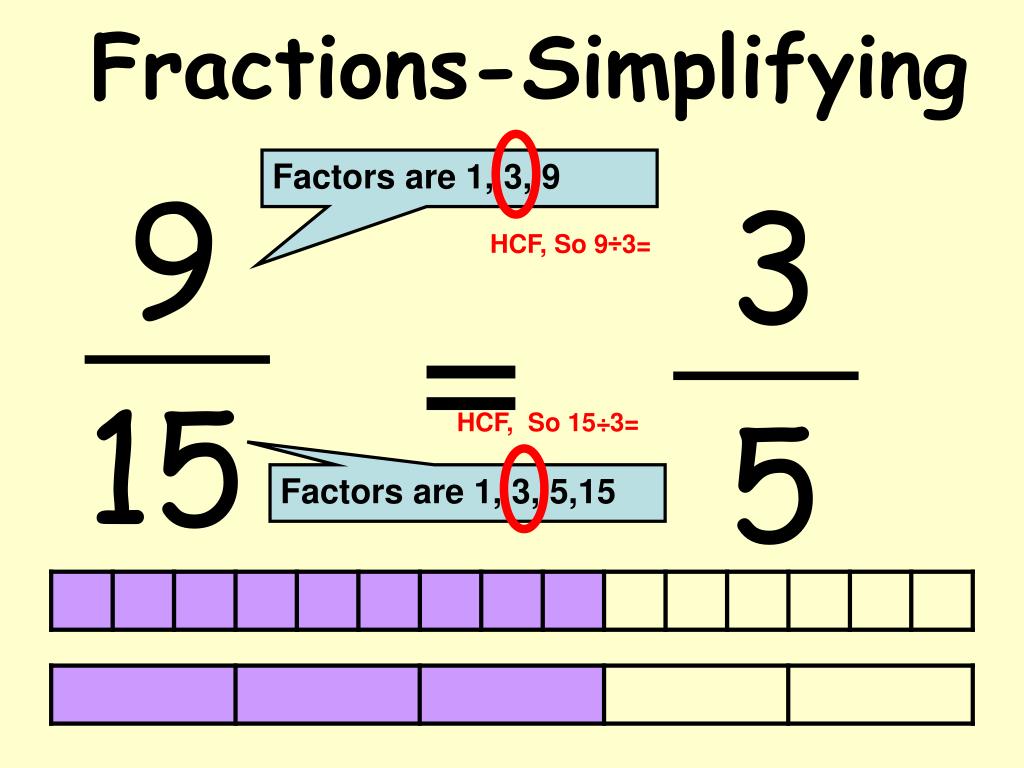
Method 1: Divide Both Numbers by the Greatest Common Divisor (GCD)
The greatest common divisor (GCD) is the largest number that divides both numbers without leaving a remainder. To simplify a fraction, find the GCD of the numerator and denominator, and then divide both numbers by the GCD.
For example, let’s simplify the fraction 12⁄16:
- Find the GCD of 12 and 16, which is 4.
- Divide both numbers by 4: 12 ÷ 4 = 3, 16 ÷ 4 = 4.
- The simplified fraction is 3⁄4.
🤔 Note: You can use online tools or calculators to find the GCD of two numbers.
Method 2: Cancel Out Common Factors
Another way to simplify fractions is to cancel out common factors between the numerator and denominator. A common factor is a number that divides both numbers without leaving a remainder.
For example, let’s simplify the fraction 6⁄8:
- Find the common factor between 6 and 8, which is 2.
- Cancel out the common factor: 6 ÷ 2 = 3, 8 ÷ 2 = 4.
- The simplified fraction is 3⁄4.
📝 Note: This method is also known as "canceling out" or "reducing" the fraction.
Method 3: Use Prime Factorization
Prime factorization is a way to break down a number into its prime factors. To simplify a fraction using prime factorization, find the prime factors of the numerator and denominator, and then cancel out any common factors.
For example, let’s simplify the fraction 9⁄12:
- Find the prime factors of 9: 3 × 3.
- Find the prime factors of 12: 2 × 2 × 3.
- Cancel out the common factor (3): 9 ÷ 3 = 3, 12 ÷ 3 = 4.
- The simplified fraction is 3⁄4.
Method 4: Use a Simplifying Fractions Chart
A simplifying fractions chart is a visual tool that shows the relationships between fractions. You can use a chart to find the simplified fraction by finding the equivalent fraction with the smallest numerator and denominator.
For example, let’s simplify the fraction 8⁄12 using a chart:
| Fraction | Simplified Fraction |
|---|---|
| 8⁄12 | 2⁄3 |
| 12⁄16 | 3⁄4 |
| 9⁄12 | 3⁄4 |
- Find the fraction 8⁄12 in the chart.
- The simplified fraction is 2⁄3.
Method 5: Use Online Tools or Calculators
There are many online tools and calculators that can simplify fractions for you. Simply enter the fraction and click the “simplify” button.
For example, let’s simplify the fraction 12⁄16 using an online calculator:
- Enter the fraction 12⁄16 in the calculator.
- Click the “simplify” button.
- The simplified fraction is 3⁄4.
💻 Note: Online tools and calculators can save time and effort, but it's essential to understand the underlying math concepts.
What is the purpose of simplifying fractions?
+Simplifying fractions makes them easier to work with and understand. It also helps in various mathematical operations, such as addition, subtraction, multiplication, and division.
How do I find the greatest common divisor (GCD) of two numbers?
+You can use online tools or calculators to find the GCD of two numbers. Alternatively, you can list the factors of each number and find the largest common factor.
What is prime factorization?
+Prime factorization is a way to break down a number into its prime factors. It involves finding the prime numbers that multiply together to give the original number.
In conclusion, simplifying fractions is an essential math concept that can be achieved using various methods. By understanding these methods, you can simplify fractions with ease and make math operations more efficient. Remember to practice regularly to become proficient in simplifying fractions.