5 Ways to Master Operations with Integers

Mastering Operations with Integers: A Comprehensive Guide
Integer operations are a fundamental concept in mathematics, and mastering them is crucial for problem-solving and critical thinking. In this article, we will explore five ways to master operations with integers, including addition, subtraction, multiplication, and division. By the end of this article, you will have a solid understanding of how to perform these operations with ease and confidence.
Understanding Integer Operations
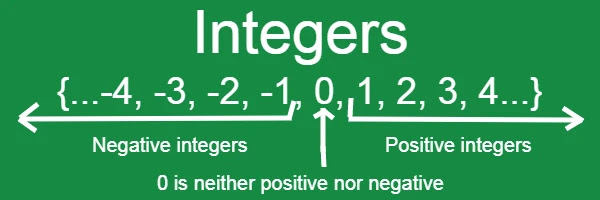
Before we dive into the five ways to master integer operations, let’s first understand what integers are and how they work. Integers are whole numbers that can be positive, negative, or zero. They are used to represent quantities that are countable, such as the number of apples in a basket or the number of students in a classroom.
Integer operations involve performing arithmetic operations on integers, such as addition, subtraction, multiplication, and division. These operations are used to solve problems and represent real-world situations, such as calculating the total cost of items, measuring the area of a room, or determining the number of items in a set.
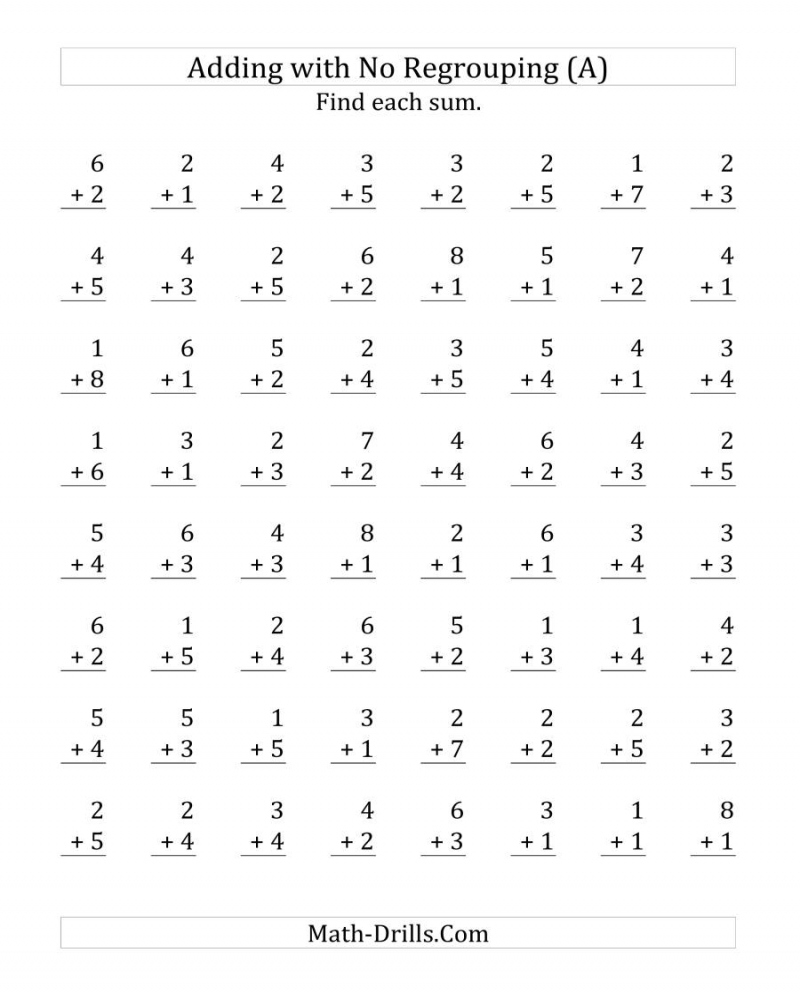
Way #1: Practice with Simple Integer Operations
One of the best ways to master integer operations is to practice with simple problems. Start by adding, subtracting, multiplying, and dividing small integers, such as 1, 2, 3, and so on. Use a worksheet or online tool to generate random problems and practice solving them.
For example, try solving the following problems:
- 2 + 3 =?
- 5 - 2 =?
- 4 × 6 =?
- 12 ÷ 4 =?
As you practice, pay attention to the rules of integer operations, such as the commutative property (e.g., 2 + 3 = 3 + 2) and the associative property (e.g., (2 + 3) + 4 = 2 + (3 + 4)).
Way #2: Use Visual Aids and Number Lines
Visual aids and number lines are powerful tools for understanding integer operations. A number line is a visual representation of integers on a line, with positive numbers to the right of zero and negative numbers to the left.
Use a number line to visualize integer operations, such as addition and subtraction. For example, to visualize the problem 2 + 3, start at 2 on the number line and move 3 units to the right. The answer is 5.
| Integer | Number Line Representation |
|---|---|
| 2 | • • |
| 3 | • • • |
| 2 + 3 | • • • • • |
Way #3: Use Real-World Examples and Word Problems
Using real-world examples and word problems is an effective way to master integer operations. Word problems involve using integers to represent real-world situations, such as calculating the cost of items or measuring the area of a room.
For example, consider the following word problem:
“Tom has 5 boxes of pencils to sell at the school store. Each box contains 12 pencils. How many pencils does Tom have in total?”
To solve this problem, multiply the number of boxes (5) by the number of pencils per box (12).
5 × 12 = 60
Tom has 60 pencils in total.
Way #4: Focus on Integer Properties and Rules
Understanding integer properties and rules is crucial for mastering integer operations. Some important properties and rules include:
- Commutative property: The order of integers does not change the result of an operation (e.g., 2 + 3 = 3 + 2).
- Associative property: The order in which integers are grouped does not change the result of an operation (e.g., (2 + 3) + 4 = 2 + (3 + 4)).
- Distributive property: The result of multiplying an integer by a sum or difference is equal to the sum or difference of the products (e.g., 2 × (3 + 4) = 2 × 3 + 2 × 4).
By understanding these properties and rules, you can simplify integer operations and solve problems more efficiently.
Way #5: Practice with Mixed Operations and Multi-Digit Numbers
Finally, practice with mixed operations and multi-digit numbers to master integer operations. Mixed operations involve combining multiple operations, such as addition and multiplication, to solve a problem.
For example, consider the following problem:
“4 × (2 + 3) - 5 =?”
To solve this problem, follow the order of operations (PEMDAS):
- Evaluate the expression inside the parentheses: 2 + 3 = 5
- Multiply 4 by the result: 4 × 5 = 20
- Subtract 5: 20 - 5 = 15
The answer is 15.
📝 Note: Practice regularly to master integer operations. Start with simple problems and gradually move on to more complex ones.
In conclusion, mastering integer operations requires practice, patience, and persistence. By following these five ways, you can develop a solid understanding of integer operations and improve your problem-solving skills. Remember to practice regularly, use visual aids and number lines, focus on integer properties and rules, and practice with mixed operations and multi-digit numbers.
What is the commutative property of integer operations?
+The commutative property states that the order of integers does not change the result of an operation (e.g., 2 + 3 = 3 + 2).
How do I visualize integer operations using a number line?
+Use a number line to visualize integer operations, such as addition and subtraction. For example, to visualize the problem 2 + 3, start at 2 on the number line and move 3 units to the right. The answer is 5.
What is the distributive property of integer operations?
+The distributive property states that the result of multiplying an integer by a sum or difference is equal to the sum or difference of the products (e.g., 2 × (3 + 4) = 2 × 3 + 2 × 4).