5 Ways to Solve Quadratic Equations Effortlessly

Unlocking the Secrets of Quadratic Equations

Quadratic equations have been a cornerstone of mathematics for centuries, and their applications can be seen in various fields, including physics, engineering, and computer science. These equations are of the form ax^2 + bx + c = 0, where a, b, and c are constants, and x is the variable. Despite their importance, many students and professionals struggle with solving quadratic equations. In this article, we will explore five ways to solve quadratic equations effortlessly, making it easier for you to tackle these equations with confidence.

Method 1: Factoring

Factoring is a popular method for solving quadratic equations, especially when the equation can be expressed as a product of two binomials. This method involves expressing the quadratic equation in the form (x + p)(x + q) = 0, where p and q are constants.

Example: Solve the quadratic equation x^2 + 5x + 6 = 0.

Solution: We can factor the equation as (x + 3)(x + 2) = 0.

This tells us that either (x + 3) = 0 or (x + 2) = 0. Solving for x, we get x = -3 or x = -2.

🤔 Note: Factoring is only possible when the quadratic equation can be expressed as a product of two binomials. If the equation cannot be factored, we need to use other methods.

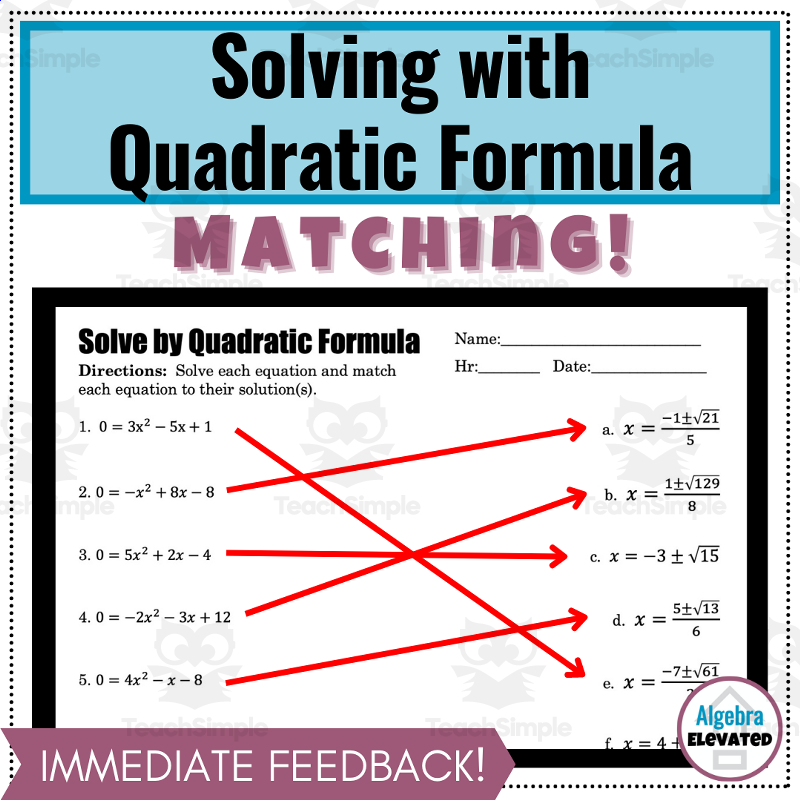
Method 2: Quadratic Formula

The quadratic formula is a powerful tool for solving quadratic equations of the form ax^2 + bx + c = 0. The formula is given by:

x = (-b ± √(b^2 - 4ac)) / 2a

Example: Solve the quadratic equation x^2 + 4x + 4 = 0.

Solution: Using the quadratic formula, we get:

x = (-4 ± √(4^2 - 4*1*4)) / 2*1 x = (-4 ± √(16 - 16)) / 2 x = (-4 ± √0) / 2 x = -4 / 2 x = -2

📝 Note: The quadratic formula can be used to solve any quadratic equation, but it's essential to check the discriminant (b^2 - 4ac) to determine the nature of the solutions.

Method 3: Graphing

Graphing is a visual method for solving quadratic equations. We can graph the quadratic function and find the x-intercepts, which represent the solutions to the equation.

Example: Solve the quadratic equation x^2 - 3x - 4 = 0.

Solution: Graphing the function f(x) = x^2 - 3x - 4, we get:

The x-intercepts are x = -1 and x = 4.

📊 Note: Graphing is a useful method for visualizing the solutions, but it may not provide exact values, especially for complex equations.

Method 4: Completing the Square

Completing the square is a method for solving quadratic equations by transforming the equation into a perfect square trinomial.

Example: Solve the quadratic equation x^2 + 6x + 8 = 0.

Solution: We can complete the square by adding and subtracting (b/2)^2 to the equation:

x^2 + 6x + 9 = 1 (x + 3)^2 = 1 x + 3 = ±1 x = -3 ± 1

👍 Note: Completing the square is a useful method for solving quadratic equations, especially when the coefficient of x^2 is not 1.

Method 5: Using Technology

In today’s digital age, we can use technology to solve quadratic equations. Graphing calculators, computer algebra systems, and online tools can help us solve quadratic equations quickly and accurately.

Example: Solve the quadratic equation x^2 - 2x - 6 = 0 using a graphing calculator.

Solution: Enter the equation into the calculator and press the solve button. The calculator will provide the solutions: x = -2 or x = 3.

📊 Note: Technology can be a powerful tool for solving quadratic equations, but it's essential to understand the underlying methods and concepts.

In conclusion, solving quadratic equations can be effortless with the right techniques and tools. By mastering these five methods, you’ll be able to tackle quadratic equations with confidence and accuracy. Remember to choose the method that best suits the equation and your personal preference.