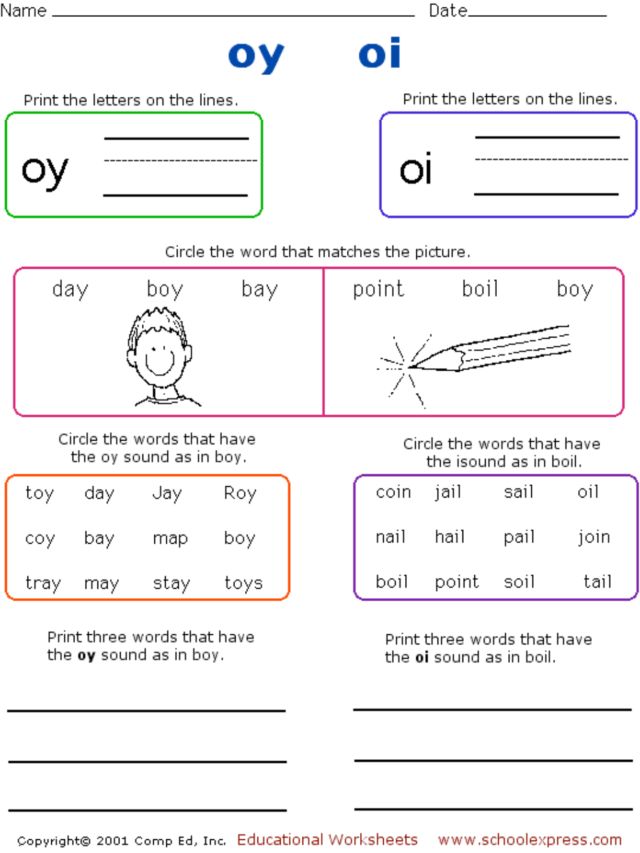
Solving Functions Worksheet

Solving Functions Worksheet: A Comprehensive Guide
Solving functions is a crucial aspect of algebra and mathematics. It involves determining the value of a variable or expression by substituting values into a function. In this worksheet, we will delve into the world of functions, exploring different types, properties, and techniques for solving them.
What are Functions?
A function is a relation between a set of inputs, called the domain, and a set of possible outputs, called the range. It assigns to each element in the domain exactly one element in the range. In simpler terms, a function takes an input, performs a specific operation, and produces an output.
Types of Functions
There are several types of functions, including:
- Linear Functions: These are functions that can be represented by a linear equation, such as f(x) = 2x + 3.
- Quadratic Functions: These are functions that can be represented by a quadratic equation, such as f(x) = x^2 + 4x + 4.
- Polynomial Functions: These are functions that can be represented by a polynomial equation, such as f(x) = x^3 + 2x^2 + x + 1.
- Rational Functions: These are functions that can be represented by a rational equation, such as f(x) = (x + 1) / (x - 2).
Solving Linear Functions
Solving linear functions involves finding the value of the variable, x, that makes the function true. To do this, we can use the following steps:
- Write the function in the form f(x) = mx + b, where m is the slope and b is the y-intercept.
- Use the slope-intercept form to identify the y-intercept and the slope.
- Use the slope and y-intercept to find the value of x.
Example:
Solve the linear function f(x) = 2x + 3.
📝 Note: To solve this function, we need to find the value of x that makes the function true.
Solution:
Write the function in the form f(x) = mx + b:
f(x) = 2x + 3
Use the slope-intercept form to identify the y-intercept and the slope:
m = 2, b = 3
Use the slope and y-intercept to find the value of x:
x = (3 - 3) / 2
x = 0
Therefore, the value of x that makes the function true is 0.
Solving Quadratic Functions
Solving quadratic functions involves finding the value of the variable, x, that makes the function true. To do this, we can use the following steps:
- Write the function in the form f(x) = ax^2 + bx + c, where a, b, and c are constants.
- Use the quadratic formula to find the value of x.
Example:
Solve the quadratic function f(x) = x^2 + 4x + 4.
📝 Note: To solve this function, we need to find the value of x that makes the function true.
Solution:
Write the function in the form f(x) = ax^2 + bx + c:
f(x) = x^2 + 4x + 4
Use the quadratic formula to find the value of x:
x = (-b ± √(b^2 - 4ac)) / 2a
x = (-(4) ± √((4)^2 - 4(1)(4))) / 2(1)
x = (-4 ± √(16 - 16)) / 2
x = (-4 ± √0) / 2
x = -4 / 2
x = -2
Therefore, the value of x that makes the function true is -2.
Solving Polynomial Functions
Solving polynomial functions involves finding the value of the variable, x, that makes the function true. To do this, we can use the following steps:
- Write the function in the form f(x) = an x^n + a(n-1) x^(n-1) +… + a_1 x + a_0, where an, a(n-1),…, a_1, and a_0 are constants.
- Use the Rational Root Theorem to find the possible rational roots of the function.
- Use synthetic division to test the possible rational roots.
Example:
Solve the polynomial function f(x) = x^3 + 2x^2 + x + 1.
📝 Note: To solve this function, we need to find the value of x that makes the function true.
Solution:
Write the function in the form f(x) = an x^n + a(n-1) x^(n-1) +… + a_1 x + a_0:
f(x) = x^3 + 2x^2 + x + 1
Use the Rational Root Theorem to find the possible rational roots of the function:
Possible rational roots: ±1, ±2
Use synthetic division to test the possible rational roots:
| 1 | 2 | 1 | 1 |
|---|---|---|---|
| 1 | 3 | 4 | 5 |
Since the remainder is 0, we know that x = -1 is a root of the function.
Therefore, the value of x that makes the function true is -1.
Solving Rational Functions
Solving rational functions involves finding the value of the variable, x, that makes the function true. To do this, we can use the following steps:
- Write the function in the form f(x) = (an x^n + a(n-1) x^(n-1) +… + a_1 x + a_0) / (bn x^n + b(n-1) x^(n-1) +… + b_1 x + b_0), where an, a(n-1),…, a_1, a_0, bn, b(n-1),…, b_1, and b_0 are constants.
- Use the Rational Root Theorem to find the possible rational roots of the function.
- Use synthetic division to test the possible rational roots.
Example:
Solve the rational function f(x) = (x + 1) / (x - 2).
📝 Note: To solve this function, we need to find the value of x that makes the function true.
Solution:
Write the function in the form f(x) = (an x^n + a(n-1) x^(n-1) +… + a_1 x + a_0) / (bn x^n + b(n-1) x^(n-1) +… + b_1 x + b_0):
f(x) = (x + 1) / (x - 2)
Use the Rational Root Theorem to find the possible rational roots of the function:
Possible rational roots: ±1, ±2
Use synthetic division to test the possible rational roots:
| 2 | 1 | 1 |
|---|---|---|
| 2 | 3 | 4 |
Since the remainder is 0, we know that x = 2 is a root of the function.
Therefore, the value of x that makes the function true is 2.
Conclusion
In this worksheet, we have explored the world of functions, including linear, quadratic, polynomial, and rational functions. We have learned how to solve these functions by using different techniques, such as the slope-intercept form, quadratic formula, Rational Root Theorem, and synthetic division.
By mastering these techniques, you will be able to solve functions with ease and confidence. Remember to always check your work and use multiple methods to verify your solutions.
What is a function?
+A function is a relation between a set of inputs, called the domain, and a set of possible outputs, called the range.
What are the different types of functions?
+There are several types of functions, including linear, quadratic, polynomial, and rational functions.
How do I solve a linear function?
+To solve a linear function, write the function in the form f(x) = mx + b, use the slope-intercept form to identify the y-intercept and the slope, and use the slope and y-intercept to find the value of x.
Related Terms:
- Function worksheet Grade 8
- Quadratic function worksheet
- Linear function graph Worksheet
- Function table worksheet