5 Easy Ways to Multiply Decimals by Powers of 10
Multiplying decimals by powers of 10 is a fundamental concept in mathematics, and it’s essential to master this skill for various mathematical operations. In this article, we will explore five easy ways to multiply decimals by powers of 10, along with practical examples and explanations.
Understanding Powers of 10
Before we dive into the methods, let’s quickly review what powers of 10 are. Powers of 10 are numbers that can be expressed as 10 raised to an integer power, such as 10^1, 10^2, 10^3, and so on. For example, 10^2 is equal to 100, and 10^3 is equal to 1000.
Method 1: Moving the Decimal Point
One of the simplest ways to multiply decimals by powers of 10 is to move the decimal point. When you multiply a decimal by a power of 10, you can simply move the decimal point to the right by the number of places indicated by the exponent.
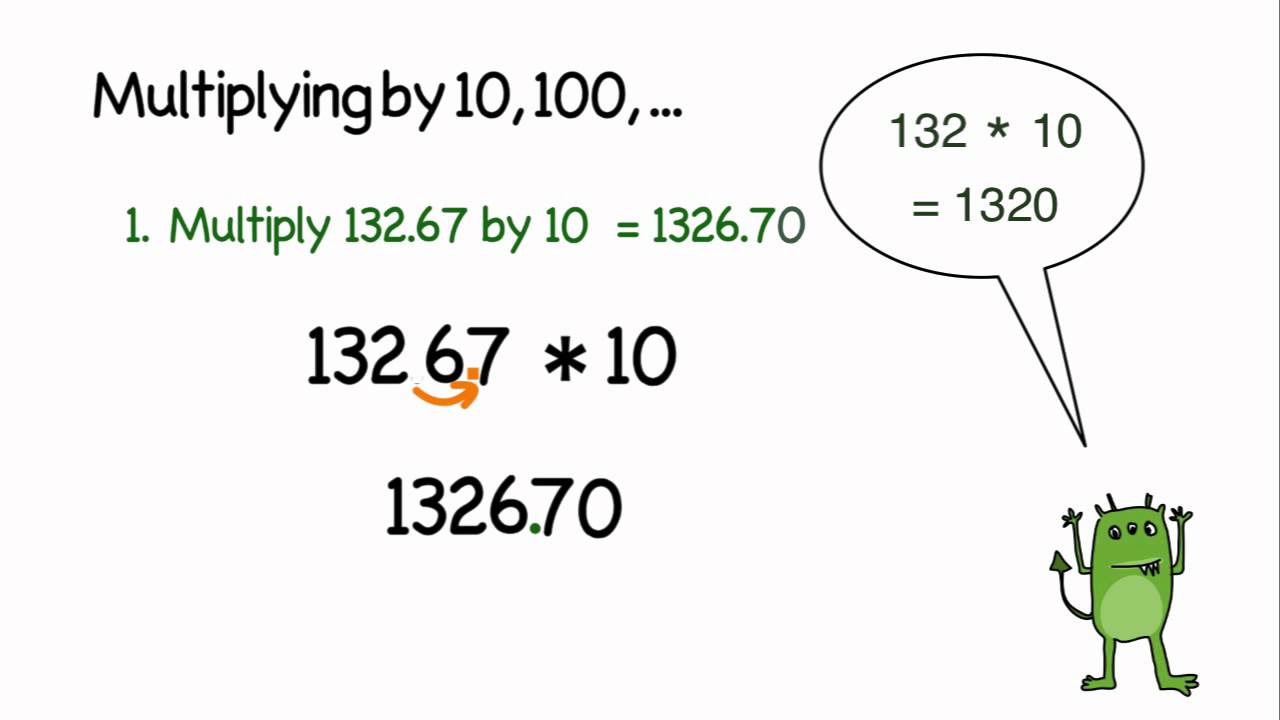
For example, let’s multiply 4.7 by 10^2 (100).
4.7 × 100 =?
To solve this, move the decimal point 2 places to the right:
4.7 → 47 → 470
So, 4.7 × 100 = 470.
Method 2: Adding Zeros
Another way to multiply decimals by powers of 10 is to add zeros to the right of the decimal number. The number of zeros you add is determined by the exponent.
Using the same example as above, let’s multiply 4.7 by 10^2 (100).
4.7 × 100 =?
To solve this, add 2 zeros to the right of 4.7:
4.7 → 4.70 → 470
So, 4.7 × 100 = 470.
📝 Note: When adding zeros, make sure to include the decimal point in the correct position.
Method 3: Using the Exponent as a Shortcut
This method involves using the exponent as a shortcut to determine the number of places to move the decimal point or add zeros.
For example, let’s multiply 2.5 by 10^3 (1000).
2.5 × 1000 =?
Since the exponent is 3, you can move the decimal point 3 places to the right or add 3 zeros to the right of 2.5.
2.5 → 2500
So, 2.5 × 1000 = 2500.
Method 4: Multiplying by 10
This method involves multiplying the decimal number by 10 repeatedly, depending on the exponent.
Using the same example as above, let’s multiply 2.5 by 10^3 (1000).
2.5 × 1000 =?
First, multiply 2.5 by 10:
2.5 × 10 = 25
Then, multiply 25 by 10 again:
25 × 10 = 250
Finally, multiply 250 by 10 one more time:
250 × 10 = 2500
So, 2.5 × 1000 = 2500.
Method 5: Using a Table
This method involves creating a table to help you visualize the multiplication process.
| Decimal Number | Power of 10 | Result |
|---|---|---|
| 4.7 | 10^1 | 47 |
| 4.7 | 10^2 | 470 |
| 4.7 | 10^3 | 4700 |
Using this table, you can easily multiply 4.7 by different powers of 10.
📝 Note: You can create a table for any decimal number and power of 10 to help you with multiplication.
In conclusion, multiplying decimals by powers of 10 is a straightforward process that can be achieved using various methods. Whether you prefer moving the decimal point, adding zeros, using the exponent as a shortcut, multiplying by 10, or creating a table, these methods will help you become more confident and proficient in your mathematical skills.
What is the difference between moving the decimal point and adding zeros?
+Both methods achieve the same result, but moving the decimal point is often faster and more efficient. Adding zeros is a more visual approach that can help you understand the concept better.
Can I use these methods for dividing decimals by powers of 10?
+No, these methods are specifically designed for multiplying decimals by powers of 10. For dividing decimals by powers of 10, you would need to use different methods, such as moving the decimal point to the left or subtracting zeros.
How can I practice multiplying decimals by powers of 10?
+You can practice multiplying decimals by powers of 10 using online resources, such as math worksheets or quizzes. You can also create your own practice problems using different decimal numbers and powers of 10.
Related Terms:
- teacher synergy llc
- Khan Academy
- IXL
- BrainPOP
- Udacity
- Duolingo