Geometry Constructions Worksheet Solutions for Students

Understanding Geometry Constructions

Geometry constructions involve creating geometric shapes using a compass and a straightedge. This technique is essential for students to understand and master, as it provides a solid foundation for more advanced mathematical concepts. In this article, we will delve into the world of geometry constructions, exploring the basics, types, and examples of constructions.

Basic Constructions

To begin with, it’s crucial to understand the basic constructions that form the building blocks of geometry. These include:

• Perpendicular Bisector: A line that passes through the midpoint of a given line segment and is perpendicular to it.
• Angle Bisector: A line that divides an angle into two equal parts.
• Line Segment: A part of a line with two endpoints.
• Circle: A set of points that are all equidistant from a central point called the center.

These basic constructions can be combined to create more complex shapes and designs.

Types of Constructions

There are several types of constructions, including:

• Line Constructions: Creating lines, line segments, and rays using a straightedge and compass.
• Circle Constructions: Drawing circles, arcs, and other curved shapes using a compass.
• Angle Constructions: Creating angles, angle bisectors, and perpendicular lines using a compass and straightedge.
• Shape Constructions: Building various shapes, such as triangles, quadrilaterals, and polygons, using a combination of line, circle, and angle constructions.

Step-by-Step Constructions

Let’s take a closer look at some step-by-step constructions:

Perpendicular Bisector Construction

• Step 1: Draw a line segment AB.
• Step 2: Place the compass point on A and draw an arc above and below the line segment.
• Step 3: Place the compass point on B and draw an arc above and below the line segment, intersecting the first arc.
• Step 4: Draw a line through the intersection points of the arcs.
• Step 5: Label the midpoint of the line segment as M.

Angle Bisector Construction

• Step 1: Draw an angle ∠ABC.
• Step 2: Place the compass point on B and draw an arc intersecting the sides of the angle.
• Step 3: Place the compass point on the intersection points of the arc and draw two arcs intersecting each other.
• Step 4: Draw a line through the intersection points of the arcs.
• Step 5: Label the angle bisector as BD.

Circle Construction

• Step 1: Draw a line segment AB.
• Step 2: Place the compass point on A and draw a circle with a radius of AB.
• Step 3: Label the center of the circle as O.

Triangle Construction

• Step 1: Draw a line segment AB.
• Step 2: Place the compass point on A and draw an arc above the line segment.
• Step 3: Place the compass point on B and draw an arc above the line segment, intersecting the first arc.
• Step 4: Draw a line through the intersection points of the arcs.
• Step 5: Label the triangle as ABC.

📝 Note: Always label your constructions clearly and accurately.

Geometry Constructions Worksheet Solutions

Here are some sample worksheet solutions:

Problem 1

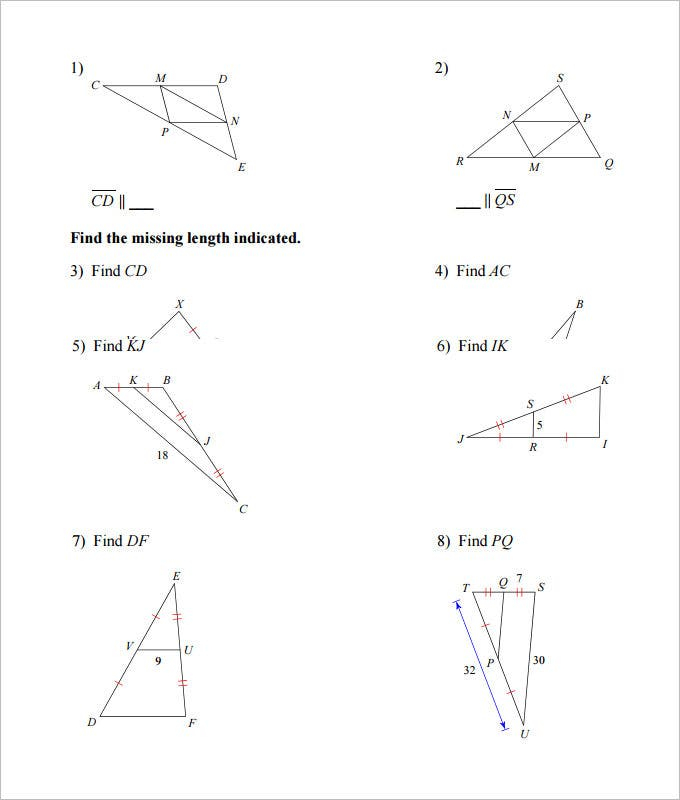
Construct a perpendicular bisector of line segment AB.

Solution

1. Draw line segment AB.
2. Place the compass point on A and draw an arc above and below the line segment.
3. Place the compass point on B and draw an arc above and below the line segment, intersecting the first arc.
4. Draw a line through the intersection points of the arcs.
5. Label the midpoint of the line segment as M.

Problem 2

Construct an angle bisector of ∠ABC.

Solution

1. Draw angle ∠ABC.
2. Place the compass point on B and draw an arc intersecting the sides of the angle.
3. Place the compass point on the intersection points of the arc and draw two arcs intersecting each other.
4. Draw a line through the intersection points of the arcs.
5. Label the angle bisector as BD.

Conclusion

Geometry constructions are an essential part of mathematics, providing a solid foundation for more advanced concepts. By mastering the basic constructions and understanding the different types of constructions, students can develop problem-solving skills and spatial reasoning. With practice and patience, students can become proficient in geometry constructions, paving the way for success in mathematics and beyond.