Mastering Decimals with Worksheets
Understanding Decimals: A Comprehensive Guide
Decimals are a fundamental concept in mathematics, and mastering them is essential for problem-solving and critical thinking. Decimals are used to represent fractions and mixed numbers in a more straightforward and efficient way. In this article, we will explore the world of decimals, including their definition, types, and operations. We will also provide worksheets and exercises to help you practice and reinforce your understanding of decimals.
What are Decimals?
Decimals are a way of expressing fractions and mixed numbers using a point (.) to separate the whole number part from the fractional part. The decimal point is used to indicate the tenths, hundredths, thousandths, and so on. For example, the decimal 3.4 represents the fraction 3 4⁄10.
Decimals can be classified into two main types:
- Terminating decimals: These are decimals that have a finite number of digits after the decimal point. For example, 0.5, 0.25, and 0.125 are all terminating decimals.
- Non-terminating decimals: These are decimals that have an infinite number of digits after the decimal point. For example, π (pi) is an irrational number that is represented as a non-terminating decimal: 3.14159265358979323846…
Operations with Decimals
Decimals can be added, subtracted, multiplied, and divided just like whole numbers. However, there are some key differences to keep in mind:
- Adding and subtracting decimals: When adding or subtracting decimals, the decimal point must be aligned. For example:
- 2.5 + 1.8 = 4.3
- 4.2 - 2.1 = 2.1
- Multiplying decimals: When multiplying decimals, the decimal point is placed after the multiplication is performed. For example:
- 2.5 × 3.2 = 8.0
- Dividing decimals: When dividing decimals, the decimal point is placed after the division is performed. For example:
- 4.2 ÷ 2.1 = 2.0
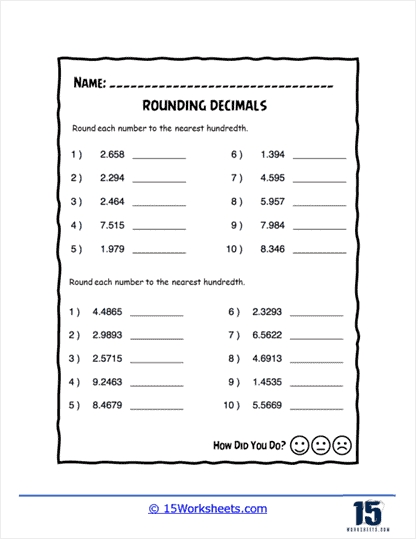
Worksheets and Exercises
Now that we have covered the basics of decimals, it’s time to practice! Here are some worksheets and exercises to help you reinforce your understanding of decimals:
Worksheet 1: Adding and Subtracting Decimals
| Problem | Solution |
|---|---|
| 2.5 + 1.8 = | 4.3 |
| 4.2 - 2.1 = | 2.1 |
| 3.4 + 2.9 = | 6.3 |
| 5.6 - 3.2 = | 2.4 |
Worksheet 2: Multiplying Decimals
| Problem | Solution |
|---|---|
| 2.5 × 3.2 = | 8.0 |
| 4.2 × 2.1 = | 8.82 |
| 3.4 × 2.9 = | 9.86 |
| 5.6 × 3.2 = | 17.92 |
Worksheet 3: Dividing Decimals
| Problem | Solution |
|---|---|
| 4.2 ÷ 2.1 = | 2.0 |
| 6.3 ÷ 3.2 = | 1.97 |
| 8.82 ÷ 2.1 = | 4.2 |
| 17.92 ÷ 3.2 = | 5.6 |
🤔 Note: Make sure to line up the decimal points when adding or subtracting decimals, and use the correct placement of the decimal point when multiplying or dividing decimals.
Tips and Tricks
Here are some additional tips and tricks to help you master decimals:
- Use a decimal grid: A decimal grid is a helpful tool for visualizing decimals. You can use a decimal grid to represent decimals as fractions or mixed numbers.
- Estimate and approximate: Estimating and approximating decimals can be helpful when performing calculations. For example, if you need to add 2.5 and 1.8, you can estimate the answer to be around 4.
- Use real-world examples: Decimals are used in many real-world applications, such as finance, science, and engineering. Using real-world examples can help make decimals more meaningful and interesting.
What is the difference between a terminating decimal and a non-terminating decimal?
+A terminating decimal has a finite number of digits after the decimal point, while a non-terminating decimal has an infinite number of digits after the decimal point.
How do I add and subtract decimals?
+To add and subtract decimals, align the decimal points and perform the calculation as you would with whole numbers.
What is the purpose of a decimal grid?
+A decimal grid is a helpful tool for visualizing decimals and representing them as fractions or mixed numbers.
Mastering decimals takes time and practice, but with the right tools and techniques, you can become proficient in no time. Remember to practice regularly and use real-world examples to make decimals more meaningful and interesting.