5 Ways to Master Quadratic Function Graphing
Understanding Quadratic Functions
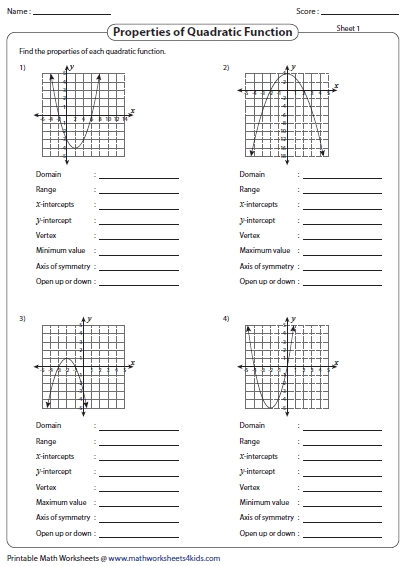
Quadratic functions are polynomial functions of degree two, which means the highest power of the variable is two. They are commonly expressed in the form of f(x) = ax^2 + bx + c, where ‘a’, ‘b’, and ‘c’ are constants, and ‘x’ is the variable. Graphing quadratic functions is a crucial concept in algebra and is used to visualize the relationship between the variables.
Method 1: Graphing Quadratic Functions Using the Standard Form
The standard form of a quadratic function is f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. This form makes it easy to identify the vertex, axis of symmetry, and the direction of the parabola.
- Identify the vertex (h, k) from the equation.
- Plot the vertex on the coordinate plane.
- Determine the axis of symmetry, which is a vertical line passing through the vertex.
- Plot additional points on either side of the axis of symmetry to determine the direction of the parabola.
📝 Note: The axis of symmetry is a vertical line that divides the parabola into two equal halves.
Method 2: Graphing Quadratic Functions Using the Vertex Form
The vertex form of a quadratic function is f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. This form is useful for graphing quadratic functions that are in the form of a perfect square trinomial.
- Identify the vertex (h, k) from the equation.
- Plot the vertex on the coordinate plane.
- Determine the axis of symmetry, which is a vertical line passing through the vertex.
- Plot additional points on either side of the axis of symmetry to determine the direction of the parabola.
Method 3: Graphing Quadratic Functions Using the Intercepts Form
The intercepts form of a quadratic function is f(x) = a(x - p)(x - q), where p and q are the x-intercepts. This form is useful for graphing quadratic functions that have two distinct x-intercepts.
- Identify the x-intercepts (p, q) from the equation.
- Plot the x-intercepts on the coordinate plane.
- Determine the axis of symmetry, which is a vertical line passing through the midpoint of the x-intercepts.
- Plot additional points on either side of the axis of symmetry to determine the direction of the parabola.
📝 Note: The x-intercepts are the points where the graph touches the x-axis.
Method 4: Graphing Quadratic Functions Using a Table of Values
Graphing quadratic functions using a table of values involves creating a table of x and y values to plot on the coordinate plane.
- Create a table of x and y values by substituting x values into the equation.
- Plot the points on the coordinate plane.
- Draw a smooth curve through the points to create the graph.
📝 Note: This method is useful for graphing quadratic functions that do not have a simple factored form.
Method 5: Graphing Quadratic Functions Using Technology
Graphing quadratic functions using technology involves using a graphing calculator or computer software to graph the function.
- Enter the equation into the graphing calculator or computer software.
- Adjust the window settings to view the entire graph.
- Use the graph to identify key features such as the vertex, axis of symmetry, and x-intercepts.
📝 Note: This method is useful for graphing quadratic functions quickly and accurately.
In conclusion, mastering quadratic function graphing involves understanding the different forms of quadratic functions and using various methods to graph them. By using these methods, you can become proficient in graphing quadratic functions and visualize the relationships between variables.
What is the standard form of a quadratic function?
+
The standard form of a quadratic function is f(x) = ax^2 + bx + c, where ‘a’, ‘b’, and ‘c’ are constants, and ‘x’ is the variable.
What is the vertex form of a quadratic function?
+
The vertex form of a quadratic function is f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola.
What is the axis of symmetry of a quadratic function?
+
The axis of symmetry is a vertical line that divides the parabola into two equal halves.