5 Ways to Master Multiplying Fractions
Unlocking the Secrets of Multiplying Fractions
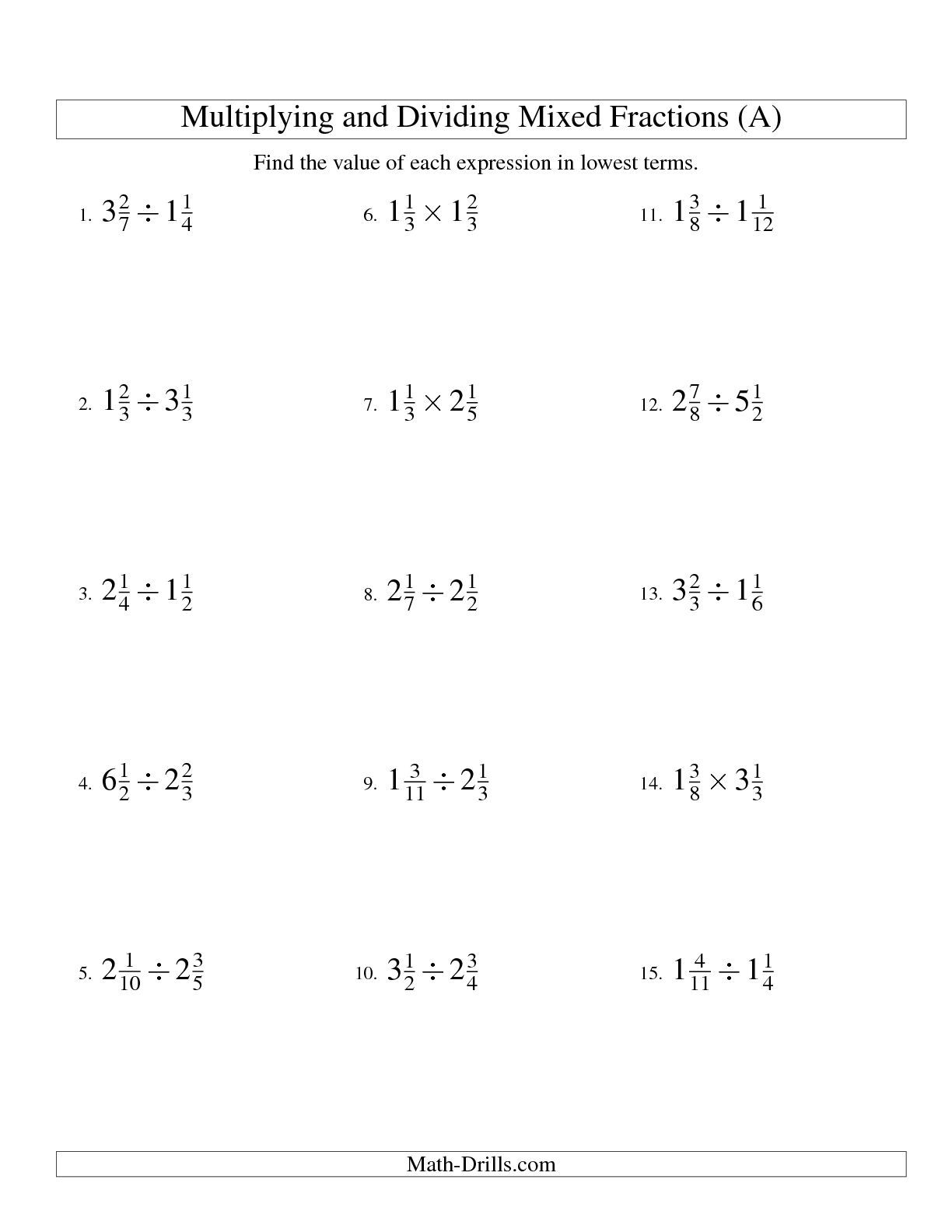
Multiplying fractions can be a daunting task for many students, but with the right approach, it can become a breeze. In this article, we will explore five ways to master multiplying fractions, making it easier for you to solve math problems with confidence.
Understanding the Basics
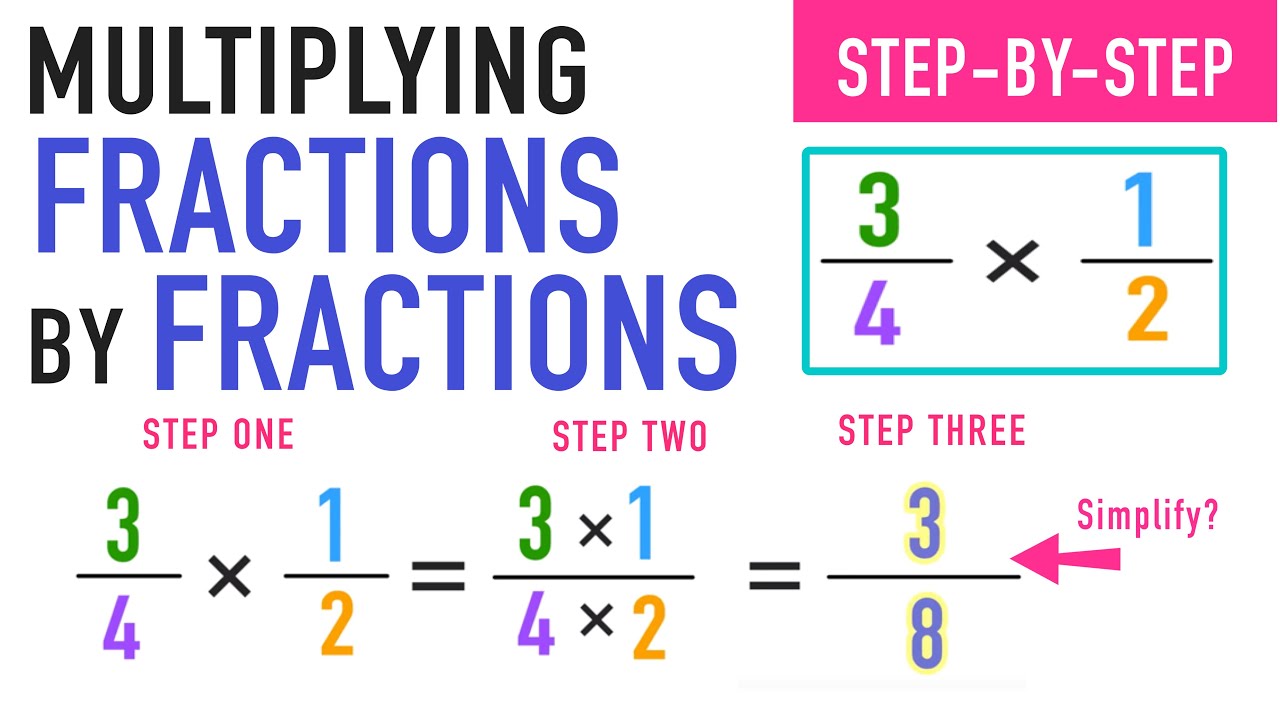
Before we dive into the five ways to master multiplying fractions, let’s quickly review the basics. To multiply fractions, you simply multiply the numerators (the numbers on top) and multiply the denominators (the numbers on the bottom). Then, you simplify the resulting fraction, if possible.
Example:
Multiply 1⁄2 and 3⁄4
Numerator: 1 × 3 = 3 Denominator: 2 × 4 = 8
Result: 3⁄8
Method 1: Using Visual Aids
Visual aids can be a great way to help you understand the concept of multiplying fractions. You can use diagrams or graphs to represent the fractions and see how they interact with each other.
Example:
Suppose you want to multiply 1⁄2 and 3⁄4. You can draw a diagram with two circles, one representing 1⁄2 and the other representing 3⁄4. Then, you can shade in the corresponding areas to see the resulting fraction.
| Fraction | Diagram |
|---|---|
| 1⁄2 |  |
| 3⁄4 |  |
| 3⁄8 |  |
Method 2: Using Real-World Examples
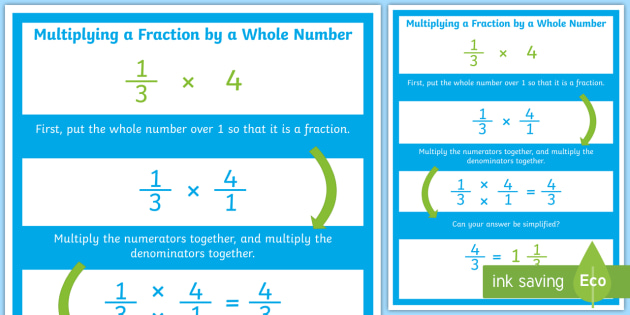
Using real-world examples can help make multiplying fractions more relatable and interesting. For instance, suppose you’re baking a cake and you need to multiply 1⁄4 cup of flour by 3⁄4 cup of sugar.
Example:
Multiply 1⁄4 cup and 3⁄4 cup
Numerator: 1 × 3 = 3 Denominator: 4 × 4 = 16
Result: 3⁄16 cup
Method 3: Breaking Down Complex Fractions
Sometimes, you may encounter complex fractions that are difficult to multiply. Breaking them down into simpler fractions can make it easier to work with.
Example:
Suppose you want to multiply 2 3⁄4 and 1 1⁄2. You can break them down into simpler fractions like this:
2 3⁄4 = 11⁄4 1 1⁄2 = 3⁄2
Then, you can multiply the numerators and denominators:
Numerator: 11 × 3 = 33 Denominator: 4 × 2 = 8
Result: 33⁄8
Method 4: Using Multiplication Charts
Multiplication charts can be a useful tool for multiplying fractions. You can create a chart with the numerators and denominators and use it to find the product.
Example:
| × | 1⁄2 | 3⁄4 |
|---|---|---|
| 1⁄2 | 1⁄4 | 3⁄8 |
| 3⁄4 | 3⁄8 | 9⁄16 |
Method 5: Practicing with Online Resources
Finally, practicing with online resources can help you master multiplying fractions. There are many websites and apps that offer interactive exercises and games to help you improve your skills.
Example:
You can try websites like Khan Academy or Mathway, which offer interactive exercises and games to help you practice multiplying fractions.
📝 Note: Practice makes perfect. The more you practice multiplying fractions, the more comfortable you'll become with the concept.
In conclusion, mastering multiplying fractions requires practice, patience, and persistence. By using visual aids, real-world examples, breaking down complex fractions, multiplication charts, and practicing with online resources, you can become a pro at multiplying fractions in no time.
What is the simplest way to multiply fractions?
+The simplest way to multiply fractions is to multiply the numerators and multiply the denominators, then simplify the resulting fraction.
Can I use visual aids to help me multiply fractions?
+Yes, visual aids like diagrams and graphs can be a great way to help you understand the concept of multiplying fractions.
How can I practice multiplying fractions online?
+You can practice multiplying fractions online by using websites like Khan Academy or Mathway, which offer interactive exercises and games.