Adding Subtracting Multiplying and Dividing Fractions Made Easy
Understanding Fractions
Fractions are a way to represent part of a whole. They consist of two parts: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into. For example, in the fraction 3⁄4, the numerator is 3 and the denominator is 4.
Adding Fractions
Adding fractions is a straightforward process, but it requires a common denominator. Here are the steps:
- Find a common denominator: The denominator is the number that appears below the line in a fraction. To add fractions, you need to have the same denominator for both fractions. If the denominators are different, you need to find the least common multiple (LCM) of the two denominators. The LCM is the smallest number that both denominators can divide into evenly.
- Convert to equivalent fractions: Once you have found the common denominator, convert both fractions to equivalent fractions with the common denominator.
- Add the numerators: Add the numerators (the numbers above the line) together, keeping the common denominator the same.
Example:
Add 1⁄4 and 1⁄6.
- Find a common denominator: The LCM of 4 and 6 is 12.
- Convert to equivalent fractions: 1⁄4 = 3⁄12 and 1⁄6 = 2⁄12.
- Add the numerators: 3 + 2 = 5, so the answer is 5⁄12.
📝 Note: When adding fractions, it's essential to have a common denominator to ensure accuracy.
Subtracting Fractions
Subtracting fractions is similar to adding fractions, but you subtract the numerators instead of adding them. Here are the steps:
- Find a common denominator: Just like with adding fractions, find the least common multiple (LCM) of the two denominators.
- Convert to equivalent fractions: Convert both fractions to equivalent fractions with the common denominator.
- Subtract the numerators: Subtract the numerators (the numbers above the line) from each other, keeping the common denominator the same.
Example:
Subtract 1⁄4 from 1⁄6.
- Find a common denominator: The LCM of 4 and 6 is 12.
- Convert to equivalent fractions: 1⁄4 = 3⁄12 and 1⁄6 = 2⁄12.
- Subtract the numerators: 2 - 3 = -1, so the answer is -1⁄12.
📝 Note: When subtracting fractions, be careful with negative signs, as they can affect the result.
Multiplying Fractions
Multiplying fractions is a bit different from adding and subtracting fractions. Here are the steps:
- Multiply the numerators: Multiply the numerators (the numbers above the line) together.
- Multiply the denominators: Multiply the denominators (the numbers below the line) together.
- Simplify the result: Simplify the resulting fraction, if possible.
Example:
Multiply 1⁄2 and 3⁄4.
- Multiply the numerators: 1 x 3 = 3.
- Multiply the denominators: 2 x 4 = 8.
- Simplify the result: The answer is 3⁄8.
📝 Note: When multiplying fractions, you don't need a common denominator, making it a simpler process than adding or subtracting fractions.
Dividing Fractions
Dividing fractions is the inverse of multiplying fractions. Here are the steps:
- Invert the second fraction: Flip the second fraction, so the numerator becomes the denominator and vice versa.
- Multiply the fractions: Multiply the fractions as you normally would.
- Simplify the result: Simplify the resulting fraction, if possible.
Example:
Divide 1⁄2 by 3⁄4.
- Invert the second fraction: Flip the second fraction, so it becomes 4⁄3.
- Multiply the fractions: Multiply 1⁄2 and 4⁄3.
- Simplify the result: The answer is 4⁄6, which simplifies to 2⁄3.
📝 Note: When dividing fractions, remember to invert the second fraction and then multiply.
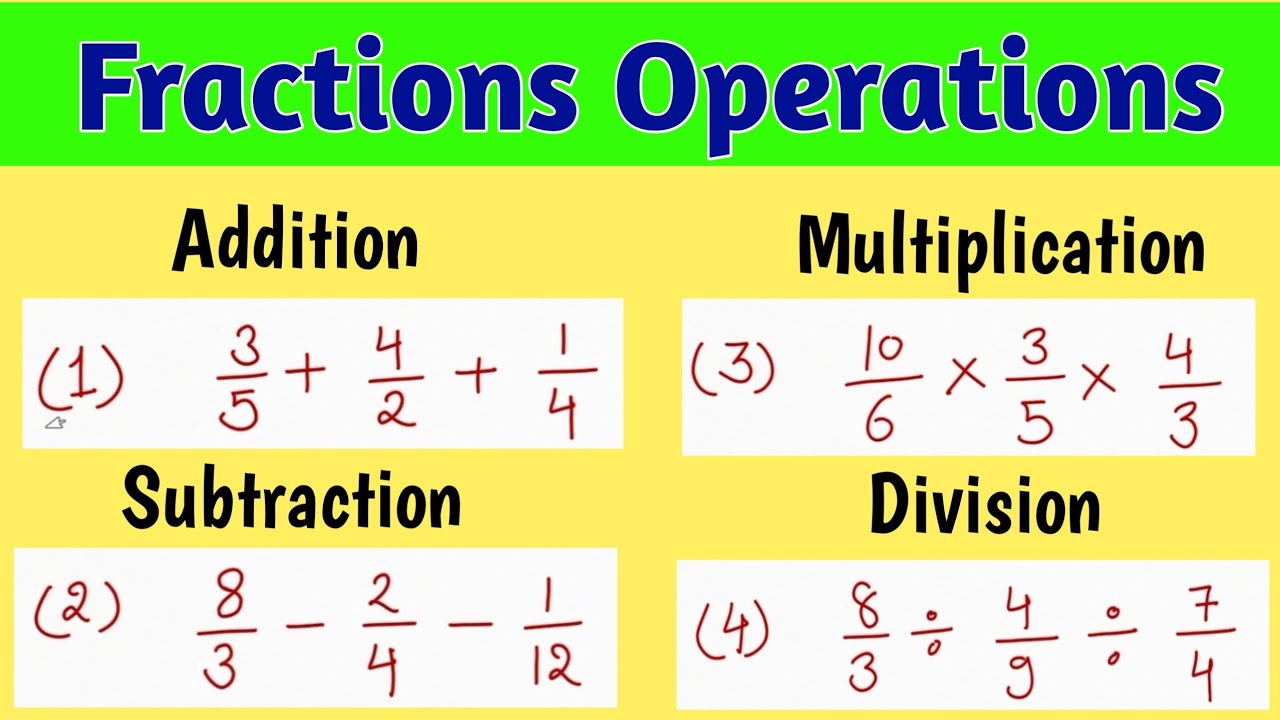
| Operation | Steps |
|---|---|
| Addition | Find a common denominator, convert to equivalent fractions, add numerators. |
| Subtraction | Find a common denominator, convert to equivalent fractions, subtract numerators. |
| Multiplication | Multiply numerators, multiply denominators, simplify result. |
| Division | Invert second fraction, multiply fractions, simplify result. |
Fractions can seem daunting, but with practice and patience, you’ll become a pro at adding, subtracting, multiplying, and dividing them.
In conclusion, fractions are an essential part of mathematics, and mastering the operations of adding, subtracting, multiplying, and dividing fractions will help you in various mathematical and real-world applications.
What is the least common multiple (LCM)?
+The LCM is the smallest number that both denominators can divide into evenly.
Why do I need to invert the second fraction when dividing fractions?
+Inverting the second fraction allows you to multiply instead of divide, making the process easier.
What is the difference between equivalent fractions and simplified fractions?
+Equivalent fractions have the same value, but may have different numerators and denominators. Simplified fractions are equivalent fractions with the smallest possible numerator and denominator.