Combining Like Terms Made Easy

Mastering the Art of Combining Like Terms

Combining like terms is a fundamental concept in algebra that can seem daunting at first, but with practice and the right strategies, it can become second nature. In this article, we will break down the concept of combining like terms, provide examples, and offer tips to help you master this essential skill.

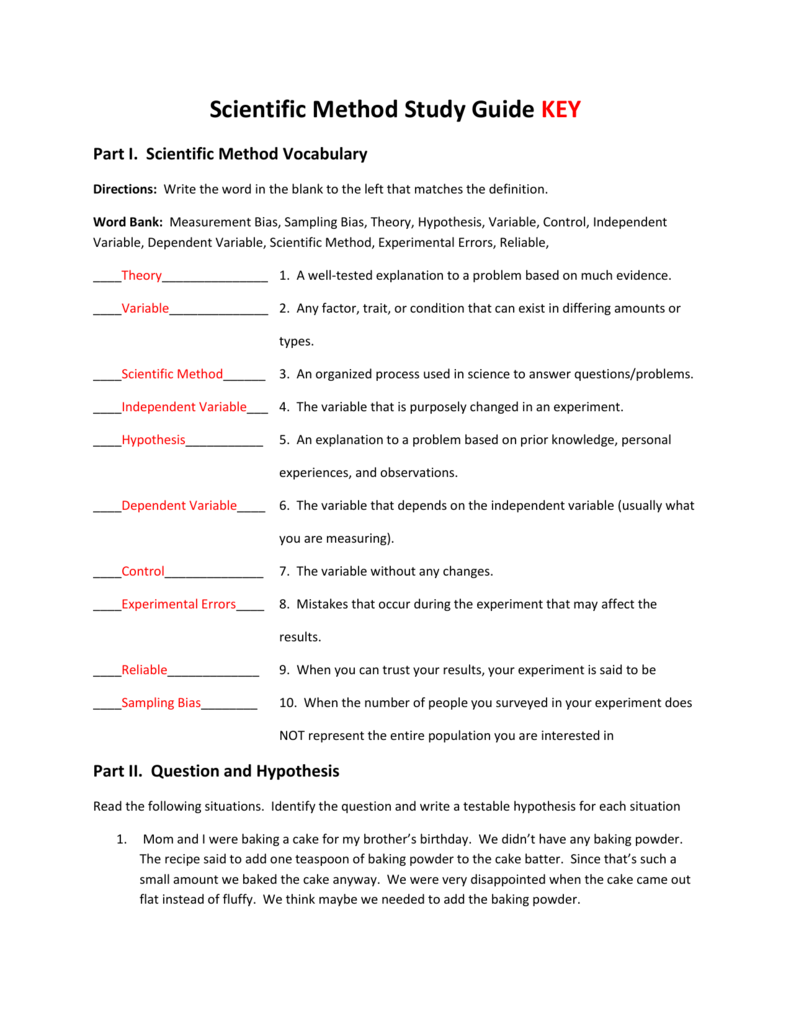
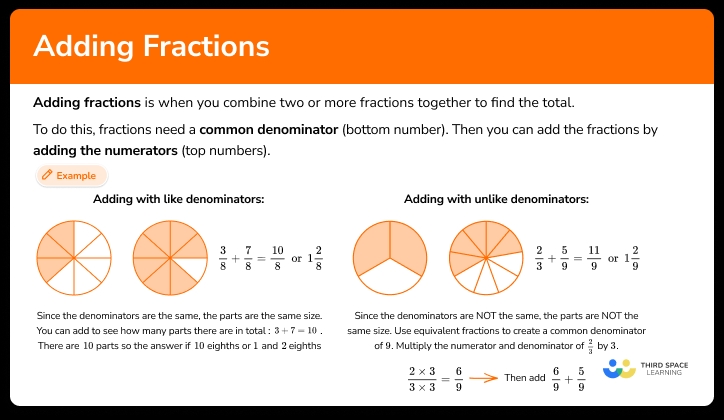
What are Like Terms?

Like terms are terms that have the same variable(s) raised to the same power. For example, 2x and 3x are like terms because they both have the variable x raised to the power of 1. On the other hand, 2x and 3y are not like terms because they have different variables.

Why is Combining Like Terms Important?

Combining like terms is essential in algebra because it helps to simplify expressions and equations. By combining like terms, you can:

• Simplify complex expressions
• Make it easier to solve equations
• Identify patterns and relationships between variables

How to Combine Like Terms

Combining like terms is a straightforward process. Here are the steps:

1. Identify the like terms in the expression or equation.
2. Add or subtract the coefficients of the like terms.
3. Keep the same variable(s) and exponent(s).

Let’s consider an example:

Suppose we have the expression 2x + 3x + 4. To combine the like terms, we follow the steps above:

• Identify the like terms: 2x and 3x are like terms because they both have the variable x raised to the power of 1.
• Add the coefficients: 2 + 3 = 5
• Keep the same variable(s) and exponent(s): x

So, the simplified expression is 5x + 4.

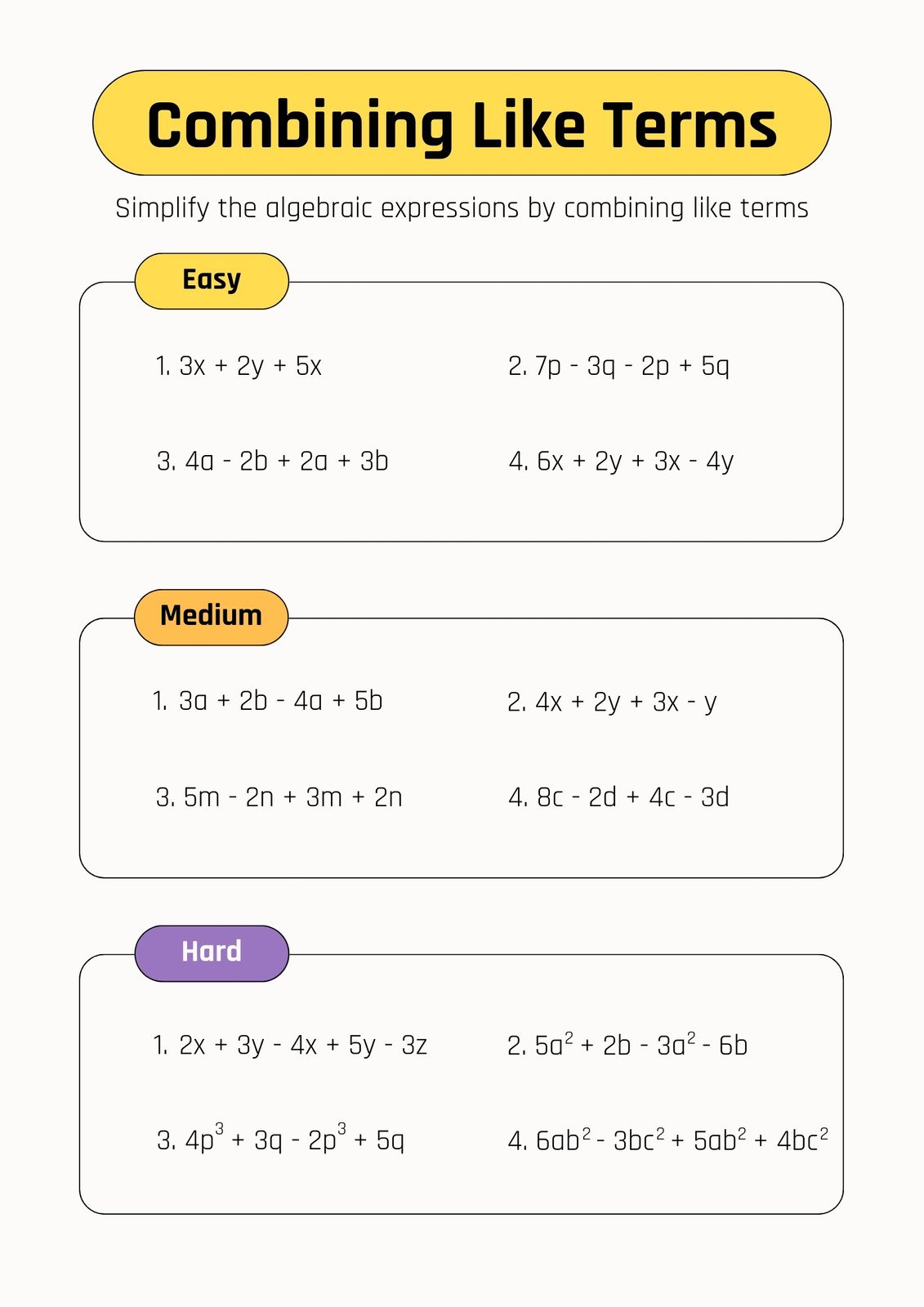
Examples and Practice

Here are some more examples to help you practice combining like terms:

• 2x + 4x - 3x =?
• 3y - 2y + 5y =?
• 2x^2 + 3x^2 - x^2 =?

Solutions:

• 2x + 4x - 3x = 3x
• 3y - 2y + 5y = 6y
• 2x^2 + 3x^2 - x^2 = 4x^2

📝 Note: When combining like terms, make sure to keep the same variable(s) and exponent(s). If the variables or exponents are different, you cannot combine the terms.

Tips and Tricks

Here are some tips to help you master the art of combining like terms:

• Identify like terms by looking for terms with the same variable(s) and exponent(s).
• Use the commutative property to rearrange the terms and make it easier to combine like terms.
• Use the distributive property to expand expressions and combine like terms.
• Practice, practice, practice! The more you practice combining like terms, the more comfortable you will become.

Common Mistakes to Avoid

Here are some common mistakes to avoid when combining like terms:

• Combining unlike terms: Make sure the terms have the same variable(s) and exponent(s).
• Forgetting to keep the same variable(s) and exponent(s).
• Not adding or subtracting the coefficients correctly.

🚨 Note: Combining like terms is a fundamental concept in algebra. Make sure to practice regularly to avoid mistakes and build a strong foundation in algebra.

Real-World Applications

Combining like terms has many real-world applications, including:

• Physics: Combining like terms is used to simplify complex equations in physics, such as the equation for force: F = ma.
• Engineering: Combining like terms is used to simplify complex equations in engineering, such as the equation for voltage: V = IR.
• Computer Science: Combining like terms is used in computer science to simplify complex algorithms and equations.

Conclusion

Combining like terms is a fundamental concept in algebra that can seem daunting at first, but with practice and the right strategies, it can become second nature. By mastering the art of combining like terms, you can simplify complex expressions and equations, identify patterns and relationships between variables, and solve problems more efficiently. Remember to practice regularly and avoid common mistakes to build a strong foundation in algebra.