5 Easy Ways to Add Fractions
Adding fractions can seem daunting, especially when dealing with different denominators. However, with a few simple steps and strategies, you can easily add fractions and become a pro in no time.
What are Fractions?
Before we dive into adding fractions, let’s quickly review what fractions are. Fractions are a way to represent part of a whole. They consist of a numerator (the top number) and a denominator (the bottom number). For example, in the fraction 1⁄2, 1 is the numerator and 2 is the denominator.
Why Do We Need to Add Fractions?
Adding fractions is a fundamental math concept that is used in various real-life situations, such as:
- Cooking and measuring ingredients
- Building and construction
- Science and engineering
- Finance and economics
5 Easy Ways to Add Fractions
Now, let’s move on to the 5 easy ways to add fractions. Follow these steps and examples to become a pro at adding fractions.
Method 1: Adding Fractions with the Same Denominator
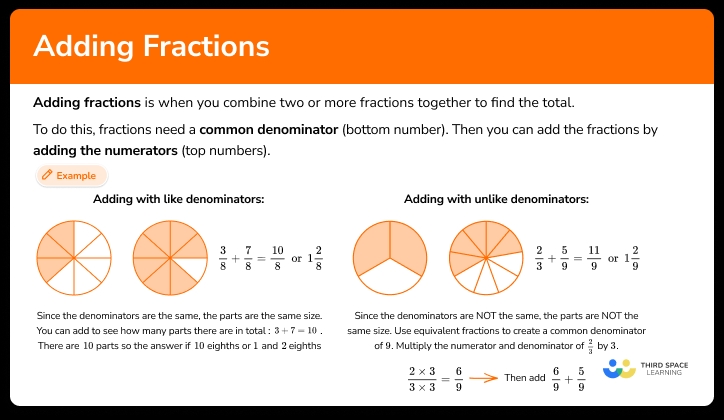
If two fractions have the same denominator, you can simply add the numerators and keep the same denominator.
Example: 1⁄4 + 2⁄4 =?
- Add the numerators: 1 + 2 = 3
- Keep the same denominator: 4
- Answer: 3⁄4
🤔 Note: When adding fractions with the same denominator, make sure to keep the same denominator.
Method 2: Adding Fractions with Different Denominators
If two fractions have different denominators, you need to find the least common multiple (LCM) of the two denominators. The LCM is the smallest number that both denominators can divide into evenly.
Example: 1⁄4 + 1⁄6 =?
- Find the LCM of 4 and 6: 12
- Convert both fractions to have a denominator of 12: 3⁄12 + 2⁄12
- Add the numerators: 3 + 2 = 5
- Keep the same denominator: 12
- Answer: 5⁄12
Method 3: Using Visual Aids
Visual aids like fraction strips or circle graphs can help you add fractions by providing a visual representation of the fractions.
Example: Use fraction strips to add 1⁄2 + 1⁄4
- Cut out fraction strips for 1⁄2 and 1⁄4
- Place the fraction strips side by side
- Count the total number of strips: 3⁄4
Method 4: Using Number Lines
Number lines can also help you add fractions by providing a visual representation of the fractions on a number line.
Example: Use a number line to add 3⁄4 + 1⁄4
- Draw a number line with markings for 1⁄4
- Place a marker at 3⁄4
- Move the marker 1⁄4 to the right: 1
- Answer: 1
Method 5: Using Real-World Examples
Using real-world examples can help make adding fractions more meaningful and fun.
Example: Tom has 1⁄2 of a pizza left over from lunch. His friend, Alex, gives him 1⁄4 of a pizza. How much pizza does Tom have now?
- Add the fractions: 1⁄2 + 1⁄4 = 3⁄4
- Answer: Tom has 3⁄4 of a pizza now
👍 Note: Using real-world examples can help make adding fractions more meaningful and fun.
Conclusion
Adding fractions may seem daunting at first, but with practice and the right strategies, you can become a pro in no time. Remember to use visual aids, number lines, and real-world examples to help make adding fractions more meaningful and fun. With these 5 easy ways to add fractions, you’ll be able to tackle any fraction problem that comes your way.
What is the easiest way to add fractions?
+The easiest way to add fractions is to use Method 1: Adding Fractions with the Same Denominator. If two fractions have the same denominator, you can simply add the numerators and keep the same denominator.
What is the least common multiple (LCM) and why is it important?
+The least common multiple (LCM) is the smallest number that both denominators can divide into evenly. It is important because it helps you find a common denominator when adding fractions with different denominators.
Can I use visual aids to add fractions?
+Yes, you can use visual aids like fraction strips or circle graphs to add fractions. Visual aids can provide a visual representation of the fractions and make it easier to add them.