Solve Complex Numbers with 5 Essential Algebra 2 Tips

Mastering Complex Numbers with 5 Essential Algebra 2 Tips
Complex numbers are a fundamental concept in Algebra 2, and understanding how to work with them is crucial for success in mathematics and science. In this article, we will explore 5 essential tips to help you solve complex numbers with confidence.
Tip 1: Understand the Basics of Complex Numbers
A complex number is a number that can be expressed in the form a + bi, where a and b are real numbers, and i is the imaginary unit, which satisfies the equation i^2 = -1. The real part of the complex number is a, and the imaginary part is bi.
For example, the complex number 3 + 4i has a real part of 3 and an imaginary part of 4i.
Tip 2: Learn How to Add and Subtract Complex Numbers
To add or subtract complex numbers, you simply add or subtract the real parts and the imaginary parts separately.
For example, to add (2 + 3i) + (4 + 5i), you would add the real parts: 2 + 4 = 6, and add the imaginary parts: 3i + 5i = 8i. The result is 6 + 8i.
Similarly, to subtract (2 + 3i) - (4 + 5i), you would subtract the real parts: 2 - 4 = -2, and subtract the imaginary parts: 3i - 5i = -2i. The result is -2 - 2i.
Tip 3: Master the Art of Multiplying Complex Numbers
To multiply complex numbers, you need to follow the distributive property (also known as FOIL).
For example, to multiply (2 + 3i) × (4 + 5i), you would use the distributive property:
(2 + 3i) × (4 + 5i) = 2(4) + 2(5i) + 3i(4) + 3i(5i) = 8 + 10i + 12i + 15i^2
Since i^2 = -1, we can simplify the expression:
= 8 + 10i + 12i - 15 = -7 + 22i
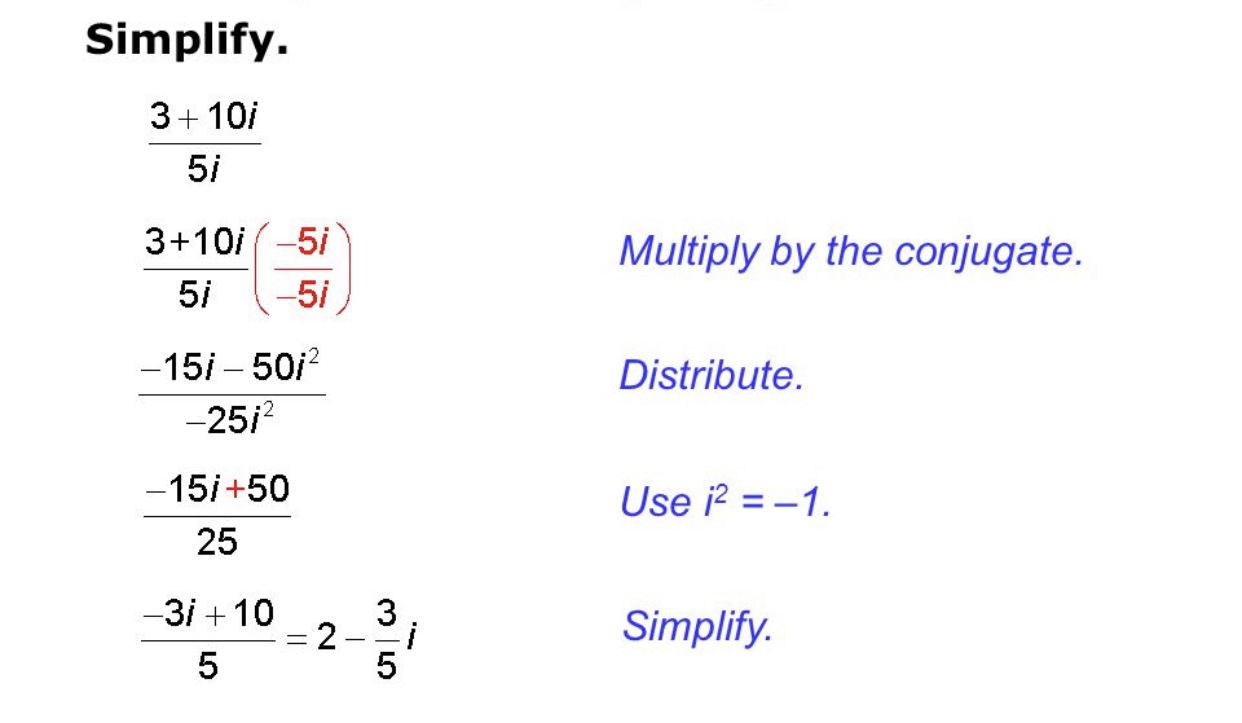
Tip 4: Learn How to Divide Complex Numbers
To divide complex numbers, you need to multiply the numerator and denominator by the conjugate of the denominator.
For example, to divide (2 + 3i) ÷ (4 + 5i), you would multiply the numerator and denominator by the conjugate of the denominator, which is 4 - 5i.
(2 + 3i) ÷ (4 + 5i) = (2 + 3i) × (4 - 5i) / (4 + 5i) × (4 - 5i) = (8 - 10i + 12i - 15i^2) / (16 - 25i^2) = (8 + 2i + 15) / (16 + 25) = (23 + 2i) / 41
Tip 5: Practice, Practice, Practice!
The key to mastering complex numbers is practice. Make sure to practice adding, subtracting, multiplying, and dividing complex numbers until you feel confident and comfortable.
Also, try to apply complex numbers to real-world problems, such as electrical engineering, physics, and computer science.
💡 Note: Practice is key to mastering complex numbers. Try to solve as many problems as you can to become more confident and proficient.
Common Complex Number Formulas
Here are some common complex number formulas to keep in mind:
| Formula | Description |
|---|---|
| (a + bi) + (c + di) = (a + c) + (b + d)i | Addition of complex numbers |
| (a + bi) - (c + di) = (a - c) + (b - d)i | Subtraction of complex numbers |
| (a + bi) × (c + di) = (ac - bd) + (ad + bc)i | Multiplication of complex numbers |
| (a + bi) ÷ (c + di) = (ac + bd) / (c^2 + d^2) + (bc - ad) / (c^2 + d^2)i | Division of complex numbers |
In conclusion, mastering complex numbers is a fundamental skill in Algebra 2, and with these 5 essential tips, you can become proficient in solving complex number problems. Remember to practice regularly and apply complex numbers to real-world problems to become more confident and proficient.
What is a complex number?
+A complex number is a number that can be expressed in the form a + bi, where a and b are real numbers, and i is the imaginary unit, which satisfies the equation i^2 = -1.
How do I add complex numbers?
+To add complex numbers, you simply add the real parts and the imaginary parts separately. For example, (2 + 3i) + (4 + 5i) = (2 + 4) + (3 + 5)i = 6 + 8i.
How do I multiply complex numbers?
+To multiply complex numbers, you need to follow the distributive property (also known as FOIL). For example, (2 + 3i) × (4 + 5i) = 2(4) + 2(5i) + 3i(4) + 3i(5i) = 8 + 10i + 12i + 15i^2.
Related Terms:
- Imaginary Numbers Worksheet pdf
- Dividing complex numbers worksheet pdf
- Simplify Imaginary Numbers Worksheet