Algebra 2 Piecewise Functions Worksheet Answers
Algebra 2 Piecewise Functions can be a bit tricky, but don’t worry, we’ve got you covered! Here’s a comprehensive guide to help you understand and solve piecewise functions.
What are Piecewise Functions?
Piecewise functions, also known as split-definition functions, are functions that have different definitions for different parts of their domain. In other words, the function’s rule changes depending on the input value.
Types of Piecewise Functions
There are two main types of piecewise functions:
- Continuous Piecewise Functions: These functions have different definitions for different parts of their domain, but the function’s value is the same at the boundary points.
- Discontinuous Piecewise Functions: These functions have different definitions for different parts of their domain, and the function’s value is not the same at the boundary points.
How to Solve Piecewise Functions
To solve piecewise functions, you need to follow these steps:
- Identify the Domain: Identify the different parts of the domain for which the function has different definitions.
- Evaluate the Function: Evaluate the function for each part of the domain separately.
- Combine the Results: Combine the results from each part of the domain to get the final answer.
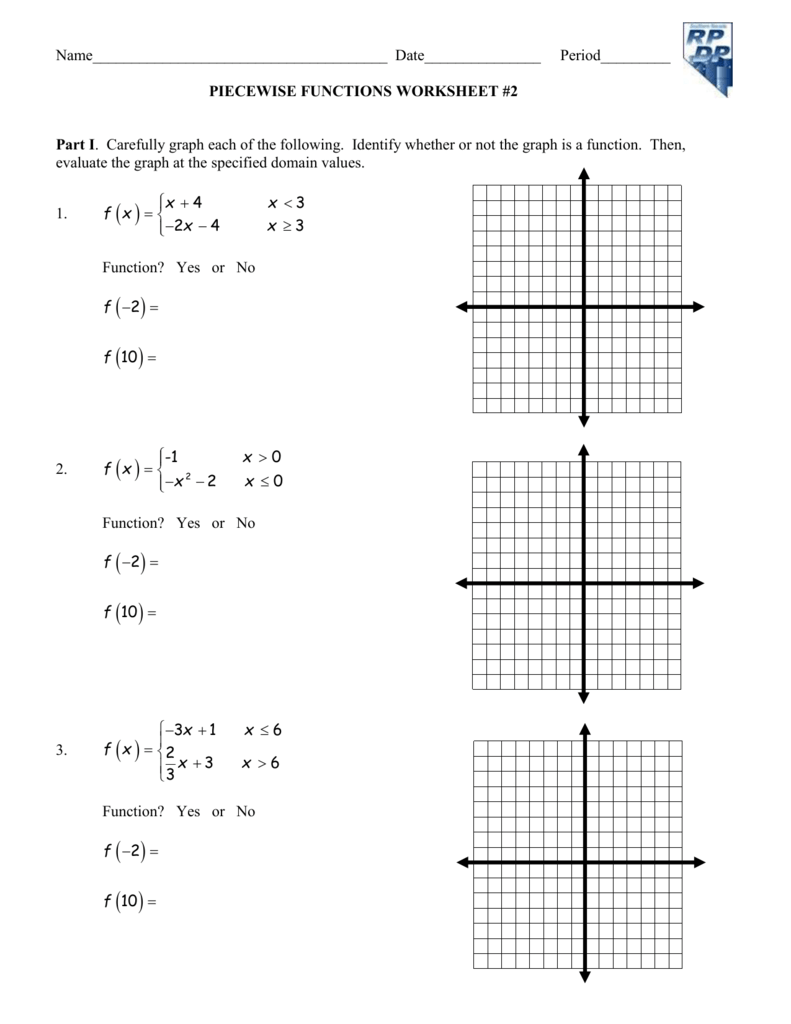
Example 1: Continuous Piecewise Function
Suppose we have a piecewise function defined as:
f(x) = {x^2, x >= 0 {2x, x < 0}
To evaluate this function at x = 2, we need to first identify which part of the domain x = 2 belongs to. Since x = 2 is greater than or equal to 0, we use the first definition of the function, f(x) = x^2.
f(2) = 2^2 = 4
So, the value of the function at x = 2 is 4.
Example 2: Discontinuous Piecewise Function
Suppose we have a piecewise function defined as:
g(x) = {x + 1, x < 2 {3x, x >= 2}
To evaluate this function at x = 2, we need to first identify which part of the domain x = 2 belongs to. Since x = 2 is greater than or equal to 2, we use the second definition of the function, g(x) = 3x.
g(2) = 3(2) = 6
However, if we evaluate the function at x = 2 using the first definition, we get:
g(2) = 2 + 1 = 3
Since the function has different values at x = 2 depending on which definition we use, the function is discontinuous at x = 2.
Worksheet Answers
Here are the answers to some common piecewise function problems:
| Problem | Answer |
|---|---|
| f(x) = {x^2, x >= 0; 2x, x < 0}, f(2) | 4 |
| g(x) = {x + 1, x < 2; 3x, x >= 2}, g(2) | 6 (using the second definition) |
| h(x) = {x^3, x > 0; -x, x <= 0}, h(-1) | 1 |
| k(x) = {2x, x >= 0; x^2, x < 0}, k(3) | 6 |
👍 Note: Make sure to identify the correct part of the domain before evaluating the function.
As you can see, piecewise functions can be a bit tricky, but with practice and patience, you can master them!
Now, it’s time to try some exercises on your own. Remember to follow the steps outlined above and to always identify the correct part of the domain before evaluating the function.
What is the main difference between continuous and discontinuous piecewise functions?
+Continuous piecewise functions have the same value at the boundary points, while discontinuous piecewise functions have different values at the boundary points.
How do you evaluate a piecewise function at a given input value?
+First, identify which part of the domain the input value belongs to. Then, use the corresponding definition of the function to evaluate the function at that input value.
Can a piecewise function have more than two definitions?
+Yes, a piecewise function can have more than two definitions. In fact, it can have as many definitions as needed to cover all parts of the domain.
Related Terms:
- Piecewise Functions Worksheet pdf