Slope Intercept Form Practice Worksheet Answers

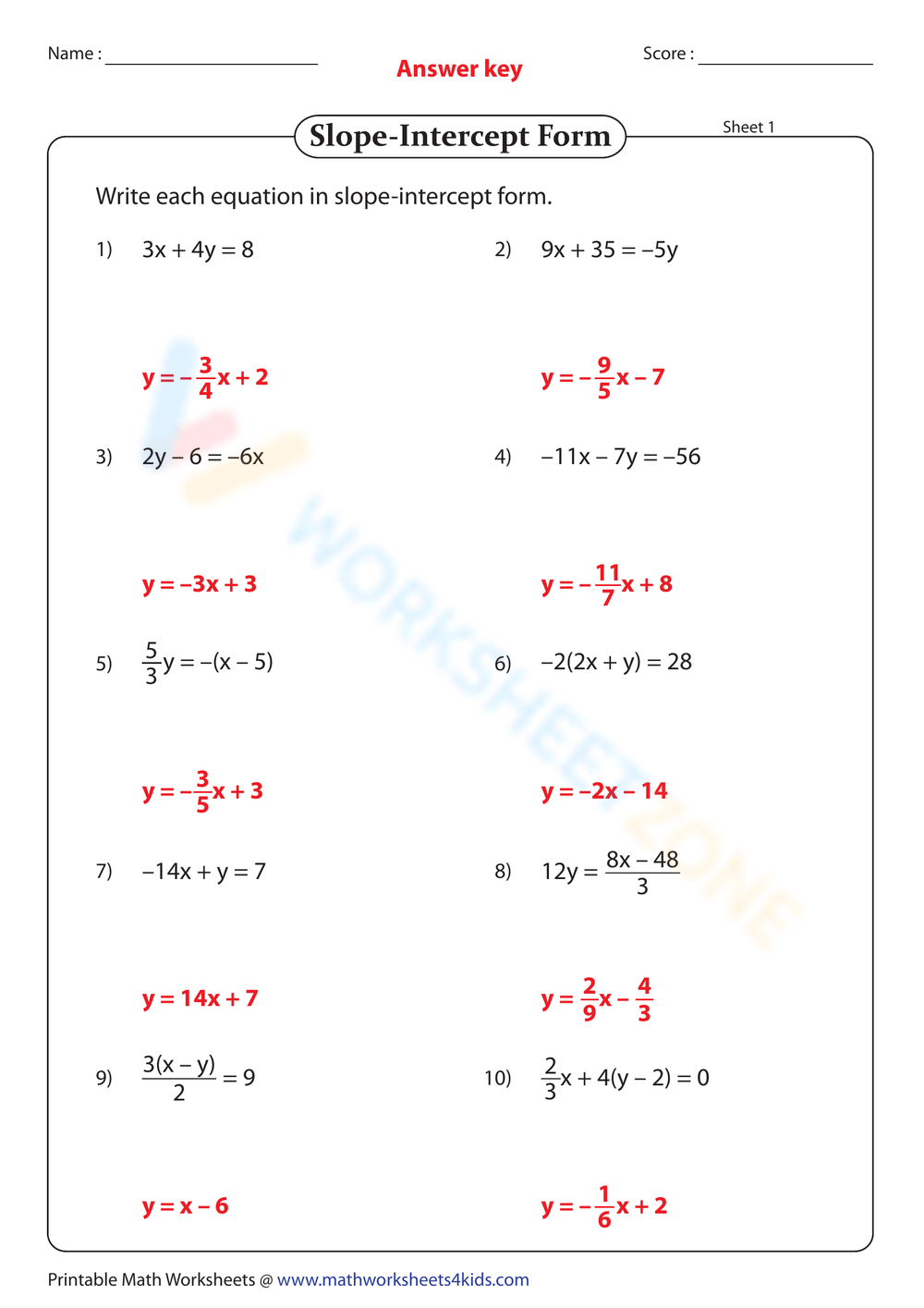
Mastering Slope Intercept Form: A Comprehensive Guide

Slope intercept form is a fundamental concept in algebra and mathematics, representing a linear equation in the form y = mx + b, where m is the slope and b is the y-intercept. Understanding and working with slope intercept form is essential for solving problems in various fields, including physics, engineering, and economics. In this article, we will delve into the world of slope intercept form, providing you with a practice worksheet and answers to help you master this concept.

What is Slope Intercept Form?

Slope intercept form is a way to represent a linear equation in the form y = mx + b, where:

• m is the slope of the line, which represents the rate of change of the line
• b is the y-intercept, which is the point where the line crosses the y-axis

For example, the equation y = 2x + 3 is in slope intercept form, where m = 2 and b = 3.

How to Convert to Slope Intercept Form

To convert an equation to slope intercept form, you need to isolate the y variable. Here are the steps:

1. Move all terms involving x to the left side of the equation.
2. Move all constant terms to the right side of the equation.
3. Simplify the equation to get it in the form y = mx + b.

For example, convert the equation 2x + 3y = 5 to slope intercept form:

1. Move all terms involving x to the left side: 2x = 5 - 3y
2. Move all constant terms to the right side: 2x - 5 = -3y
3. Simplify the equation: y = (²⁄₃)x - ⁵⁄₃

Slope Intercept Form Practice Worksheet

Here are 10 practice problems to help you master slope intercept form:

1. Convert the equation 3x + 2y = 7 to slope intercept form.
2. Find the equation of the line that passes through the point (2,3) with a slope of 4.
3. Convert the equation x - 2y = 3 to slope intercept form.
4. Find the equation of the line that passes through the points (1,2) and (3,4).
5. Convert the equation 2x + 5y = 11 to slope intercept form.
6. Find the equation of the line that has a slope of 2 and passes through the point (4,5).
7. Convert the equation x - 3y = 2 to slope intercept form.
8. Find the equation of the line that passes through the points (2,1) and (4,3).
9. Convert the equation 4x + 2y = 9 to slope intercept form.
10. Find the equation of the line that has a slope of 3 and passes through the point (2,1).

Slope Intercept Form Practice Worksheet Answers

Here are the answers to the practice problems:

1. y = (-³⁄₂)x + ⁷⁄₂
2. y = 4x - 5
3. y = (¹⁄₂)x - ³⁄₂
4. y = x + 1
5. y = (-²⁄₅)x + ¹¹⁄₅
6. y = 2x - 3
7. y = (¹⁄₃)x - ²⁄₃
8. y = x - 1
9. y = (-2)x + ⁹⁄₂
10. y = 3x - 5

📝 Note: Make sure to check your work and simplify your answers to get the correct slope intercept form.

Conclusion

Mastering slope intercept form is essential for solving problems in various fields. With practice and patience, you can become proficient in converting equations to slope intercept form and finding the equations of lines. Remember to check your work and simplify your answers to get the correct slope intercept form. With this guide and practice worksheet, you are well on your way to becoming a pro at slope intercept form.