Mastering Inverse Functions with Worksheet 7.4 Solutions

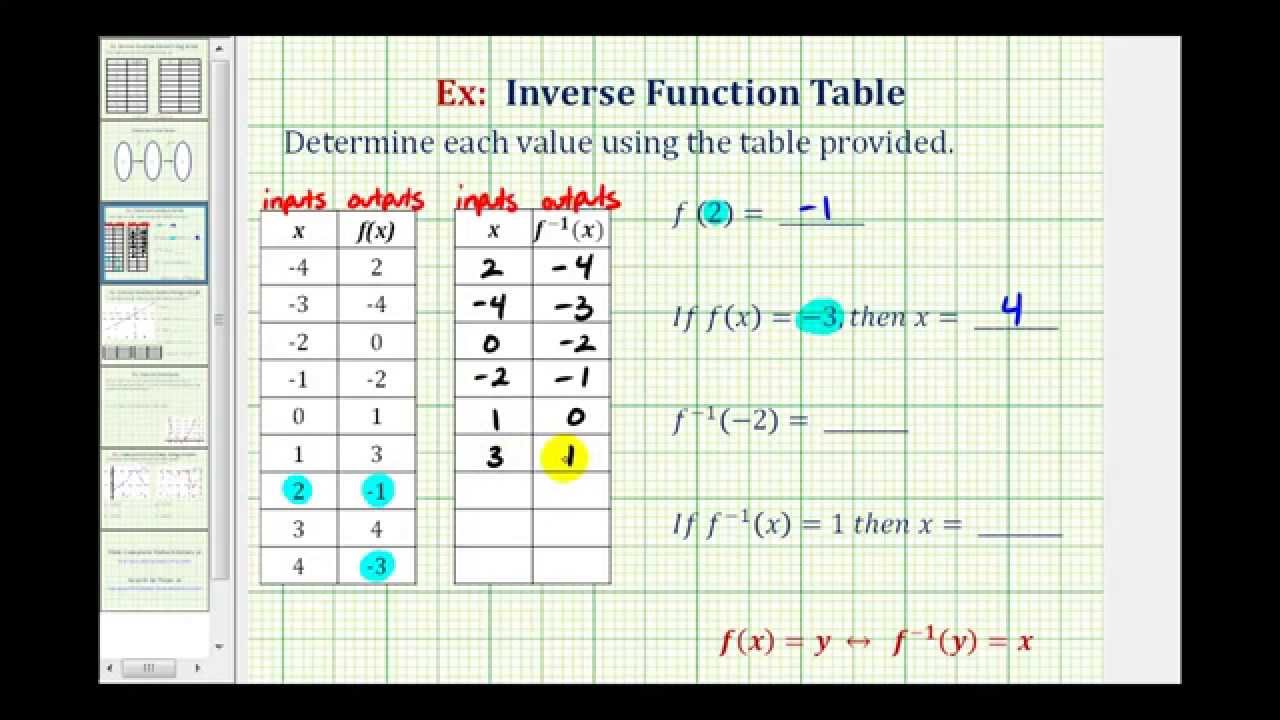
Understanding Inverse Functions

Inverse functions are a fundamental concept in mathematics, and they play a crucial role in various mathematical operations, including solving equations and graphing functions. In this blog post, we will delve into the world of inverse functions, exploring their definition, properties, and applications. We will also provide a comprehensive guide on how to solve inverse function problems using Worksheet 7.4 solutions.

What are Inverse Functions?

Inverse functions are functions that reverse the operation of the original function. In other words, if a function f(x) takes an input x and produces an output y, then the inverse function f^(-1)(x) takes the input y and produces the output x. The notation f^(-1) is read as “f inverse” and is used to denote the inverse function.

Properties of Inverse Functions

Inverse functions have several important properties that make them useful in various mathematical operations:

• Domain and Range: The domain of the inverse function is the range of the original function, and the range of the inverse function is the domain of the original function.
• One-to-One Correspondence: Inverse functions establish a one-to-one correspondence between the inputs and outputs of the original function.
• Reversibility: Inverse functions can be used to reverse the operation of the original function.

How to Find the Inverse of a Function

Finding the inverse of a function involves several steps:

1. Step 1: Write the function as y = f(x).
2. Step 2: Swap the x and y variables to get x = f(y).
3. Step 3: Solve the resulting equation for y.

For example, consider the function f(x) = 2x + 1. To find the inverse of this function, we follow the steps above:

1. Step 1: Write the function as y = 2x + 1.
2. Step 2: Swap the x and y variables to get x = 2y + 1.
3. Step 3: Solve the resulting equation for y:

x = 2y + 1

Subtract 1 from both sides:

x - 1 = 2y

Divide both sides by 2:

(x - 1)/2 = y

So, the inverse of the function f(x) = 2x + 1 is f^(-1)(x) = (x - 1)/2.

Solving Inverse Function Problems using Worksheet 7.4 Solutions

Worksheet 7.4 provides a comprehensive set of problems to help you practice solving inverse function problems. Here are some examples of how to solve these problems using the steps outlined above:

Problem 1: Find the inverse of the function f(x) = 3x - 2.

Solution:

1. Step 1: Write the function as y = 3x - 2.
2. Step 2: Swap the x and y variables to get x = 3y - 2.
3. Step 3: Solve the resulting equation for y:

x = 3y - 2

Add 2 to both sides:

x + 2 = 3y

Divide both sides by 3:

(x + 2)/3 = y

So, the inverse of the function f(x) = 3x - 2 is f^(-1)(x) = (x + 2)/3.

Problem 2: Find the inverse of the function f(x) = x^2 + 1.

Solution:

1. Step 1: Write the function as y = x^2 + 1.
2. Step 2: Swap the x and y variables to get x = y^2 + 1.
3. Step 3: Solve the resulting equation for y:

x = y^2 + 1

Subtract 1 from both sides:

x - 1 = y^2

Take the square root of both sides:

±√(x - 1) = y

So, the inverse of the function f(x) = x^2 + 1 is f^(-1)(x) = ±√(x - 1).

Important Notes

• When solving inverse function problems, make sure to check your work by plugging the inverse function back into the original function to ensure that it produces the original output.

In conclusion, inverse functions are an essential concept in mathematics, and mastering them can help you solve a wide range of mathematical problems. By following the steps outlined above and practicing with Worksheet 7.4 solutions, you can become proficient in finding the inverses of functions and applying them to real-world problems.

Related Terms:

• Inverse function pdf
• 10.3 Practice - Inverse Functions
• Inverse functions Precalculus Worksheet