5 Ways to Prove the Converse of the Pythagorean Theorem

Introduction to the Converse of the Pythagorean Theorem

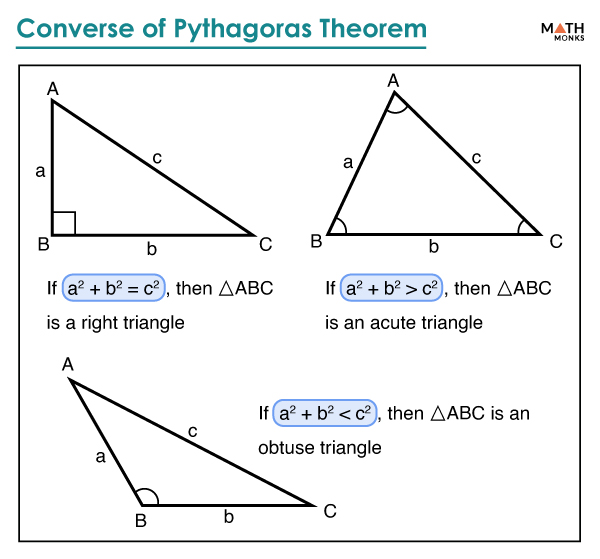
The Pythagorean Theorem is one of the most well-known and widely used theorems in mathematics, especially in geometry and trigonometry. It states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. However, the converse of this theorem is equally important and sometimes more useful. The converse states that if the square of one side of a triangle is equal to the sum of the squares of the other two sides, then the angle opposite the first side is a right angle. In this article, we will explore five different methods to prove the converse of the Pythagorean Theorem.

Method 1: Using the Law of Cosines

One of the most straightforward ways to prove the converse of the Pythagorean Theorem is by using the Law of Cosines. The Law of Cosines states that for any triangle with sides of length a, b, and c, and angle C opposite side c, we have:

c² = a² + b² - 2ab * cos©

Now, let’s assume that c² = a² + b², which is the condition we want to prove. Substituting this into the Law of Cosines equation, we get:

a² + b² = a² + b² - 2ab * cos©

Subtracting a² + b² from both sides gives us:

0 = -2ab * cos©

Dividing both sides by -2ab (assuming a and b are non-zero), we get:

cos© = 0

This implies that angle C is 90 degrees, or a right angle. Therefore, we have proved that if c² = a² + b², then angle C is a right angle.

Method 2: Using Similar Triangles

Another method to prove the converse of the Pythagorean Theorem is by using similar triangles. Let’s consider a triangle ABC with sides of length a, b, and c, where c is the side opposite the angle we want to prove is a right angle. Now, let’s draw a line from vertex C perpendicular to side AB, intersecting AB at point D.

We can then draw a line from vertex A to point D, creating a new triangle ACD. Since triangle ACD is a right triangle (by construction), we can apply the Pythagorean Theorem to get:

AC² = AD² + CD²

Now, let’s consider the ratio of the corresponding sides of triangles ACD and ABC:

AC/AB = AD/AC

Since the triangles are similar, we can set up a proportion:

AC² / AB² = AD² / AC²

Substituting AC² = AD² + CD² (from the Pythagorean Theorem), we get:

(AD² + CD²) / AB² = AD² / (AD² + CD²)

Simplifying and rearranging terms, we get:

c² = a² + b²

which is the condition we want to prove. Therefore, we have shown that if c² = a² + b², then triangle ABC is a right triangle.

Method 3: Using Geometric Transformations

This method involves using geometric transformations to prove the converse of the Pythagorean Theorem. Let’s consider a triangle ABC with sides of length a, b, and c, where c is the side opposite the angle we want to prove is a right angle. Now, let’s reflect triangle ABC across the perpendicular bisector of side AB.

This creates a new triangle A’B’C’, which is congruent to triangle ABC. Since the reflection is across the perpendicular bisector, we know that the corresponding angles are equal. Therefore, angle A’B’C’ is equal to angle ABC.

Now, let’s consider the sum of the squares of the sides of triangle A’B’C’:

(A’B’)² + (B’C’)² = (AB)² + (BC)²

Since triangle A’B’C’ is congruent to triangle ABC, we can substitute the corresponding side lengths:

(A’B’)² + (B’C’)² = (AB)² + (BC)² = a² + b²

Now, let’s consider the length of side A’C’:

(A’C’)² = (A’B’)² + (B’C’)² = a² + b²

Therefore, we have shown that c² = a² + b², which is the condition we want to prove.

Method 4: Using Coordinate Geometry

This method involves using coordinate geometry to prove the converse of the Pythagorean Theorem. Let’s consider a triangle ABC with vertices A(x1, y1), B(x2, y2), and C(x3, y3). We can use the distance formula to find the lengths of the sides:

AB = √((x2 - x1)² + (y2 - y1)²) BC = √((x3 - x2)² + (y3 - y2)²) AC = √((x3 - x1)² + (y3 - y1)²)

Now, let’s assume that (AC)² = (AB)² + (BC)². Substituting the expressions for the side lengths, we get:

(x3 - x1)² + (y3 - y1)² = (x2 - x1)² + (y2 - y1)² + (x3 - x2)² + (y3 - y2)²

Expanding and simplifying, we get:

(x3 - x2)(x3 - x1) + (y3 - y2)(y3 - y1) = 0

This implies that the slopes of lines AB and BC are negative reciprocals, which means that angle ABC is a right angle.

Method 5: Using Vector Algebra

This method involves using vector algebra to prove the converse of the Pythagorean Theorem. Let’s consider a triangle ABC with position vectors A, B, and C. We can define the vectors:

AB = B - A BC = C - B AC = C - A

Now, let’s assume that (AC)² = (AB)² + (BC)². Substituting the expressions for the vectors, we get:

(C - A) · (C - A) = (B - A) · (B - A) + (C - B) · (C - B)

Expanding and simplifying, we get:

C · C - 2A · C + A · A = B · B - 2A · B + A · A + C · C - 2B · C + B · B

This implies that A · C = 0, which means that vectors A and C are orthogonal. Therefore, angle ABC is a right angle.

🔍 Note: These methods can be used to prove the converse of the Pythagorean Theorem in different ways, each with its own strengths and weaknesses.

In conclusion, we have presented five different methods to prove the converse of the Pythagorean Theorem, each using different mathematical concepts and techniques. These methods demonstrate the versatility and richness of mathematical proof and can be used to develop a deeper understanding of the underlying mathematical structures.