5 Ways to Master Sine and Cosine Graphing

Understanding the Basics of Sine and Cosine Graphs

Sine and cosine graphs are fundamental concepts in trigonometry, and mastering them can be a game-changer for students and professionals alike. These graphs are crucial in understanding various real-world phenomena, from the motion of waves to the rotation of objects. In this article, we will explore five ways to master sine and cosine graphing, making it easier for you to tackle even the most complex problems.

1. Understanding the Periodic Nature of Sine and Cosine Functions

The sine and cosine functions are periodic, meaning they repeat their values at regular intervals. The period of both functions is 2π, which means that their graphs repeat every 2π units. This property is essential in graphing sine and cosine functions, as it allows you to identify the repeating patterns in their graphs.

To master this concept, try the following:

• Identify the period of the function: 2π
• Determine the amplitude (maximum value) and the range of the function
• Use the periodic nature to sketch the graph over multiple periods

2. Visualizing the Graphs of Sine and Cosine Functions

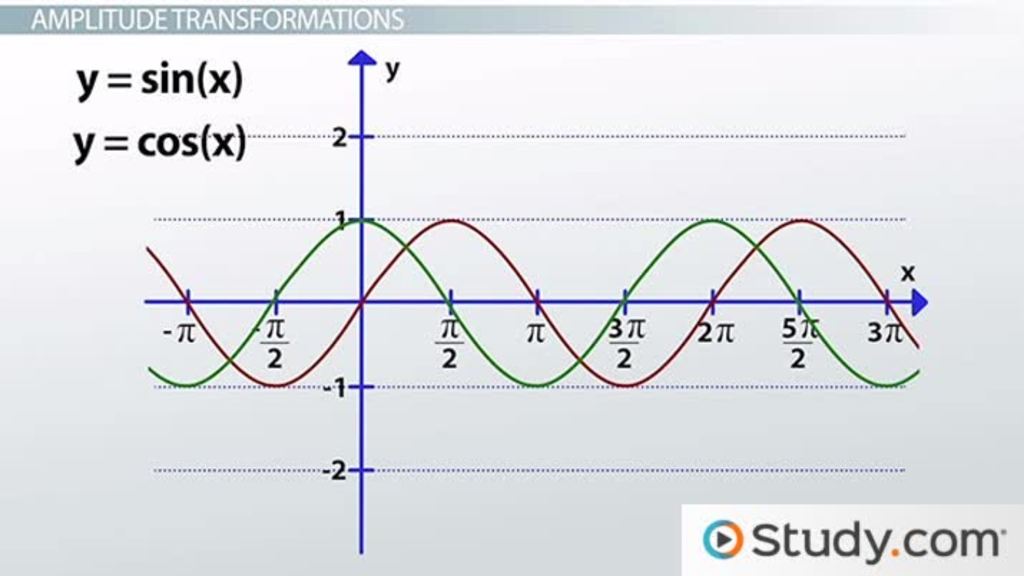
Visualizing the graphs of sine and cosine functions can be a challenge, but there are a few tricks to help you. One way to visualize the graph of a sine function is to start at the origin (0,0) and move to the right. As you move, the graph will oscillate between the maximum and minimum values.

To visualize the cosine function, you can use a similar approach, but start at the point (0,1) instead.

Here are some key points to keep in mind when visualizing the graphs:

• The sine function starts at the origin (0,0) and moves to the right, oscillating between the maximum and minimum values.
• The cosine function starts at the point (0,1) and moves to the right, oscillating between the maximum and minimum values.
• The graphs of both functions are symmetric about the x-axis.

📝 Note: Use a graphing calculator or software to visualize the graphs and explore their properties.

3. Identifying Key Features of Sine and Cosine Graphs

Sine and cosine graphs have several key features that you need to be familiar with. These include:

• Amplitude: The maximum value of the function, which determines the height of the graph.
• Period: The distance over which the graph repeats.
• Phase shift: The horizontal shift of the graph, which determines the starting point of the oscillation.
• Vertical shift: The vertical shift of the graph, which determines the position of the graph on the y-axis.

To master this concept, try the following:

• Identify the amplitude, period, phase shift, and vertical shift of the function
• Use these features to sketch the graph
• Experiment with different values of these features to see how they affect the graph

4. Graphing Sine and Cosine Functions with Transformations

Graphing sine and cosine functions with transformations is a crucial skill in trigonometry. Transformations can be used to shift, stretch, or compress the graph of a function.

Here are some common transformations you need to know:

• Horizontal shift: Shift the graph to the left or right by adding or subtracting a constant from the input.
• Vertical shift: Shift the graph up or down by adding or subtracting a constant from the output.
• Stretch or compression: Stretch or compress the graph horizontally or vertically by multiplying or dividing the input or output by a constant.

To master this concept, try the following:

• Apply different transformations to the graph of a sine or cosine function
• Use a graphing calculator or software to visualize the effects of these transformations
• Practice graphing functions with multiple transformations

5. Using Technology to Enhance Your Understanding

Technology can be a powerful tool in mastering sine and cosine graphing. Graphing calculators and software can help you visualize the graphs and explore their properties.

Here are some ways to use technology to enhance your understanding:

• Graphing calculators: Use a graphing calculator to visualize the graphs of sine and cosine functions.
• Software: Use software such as Desmos or GeoGebra to explore the properties of sine and cosine graphs.
• Interactive simulations: Use interactive simulations to explore the effects of transformations on the graph of a sine or cosine function.

By following these five ways to master sine and cosine graphing, you will be well on your way to becoming proficient in trigonometry. Remember to practice regularly and use technology to enhance your understanding.

As you continue to explore the world of trigonometry, keep in mind that mastering sine and cosine graphing is a skill that takes time and practice. With persistence and dedication, you can overcome any challenges and achieve your goals.