4 Types of Slopes to Master
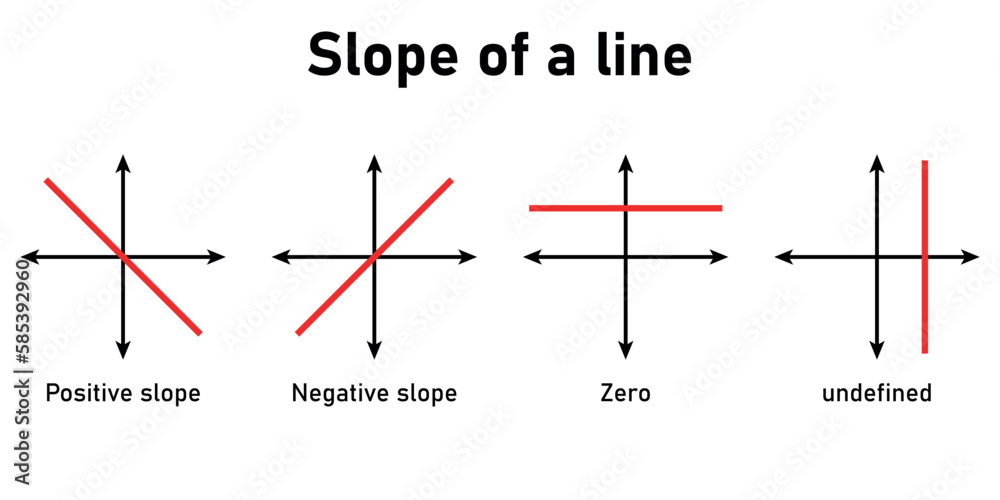
Understanding Slopes in Mathematics
In mathematics, a slope is a measure of how much a line rises (or falls) vertically over a given horizontal distance. It’s a fundamental concept in algebra, geometry, and calculus, and is used to describe the steepness of a line. Mastering different types of slopes is essential for problem-solving and graphing linear equations. In this article, we will explore four types of slopes that you need to know.
1. Positive Slope
A positive slope indicates that the line rises from left to right. The slope is calculated by dividing the vertical change (rise) by the horizontal change (run). For example, if a line rises 3 units vertically and moves 4 units horizontally, the slope would be 3⁄4 or 0.75. A positive slope is denoted by a positive number.
🔍 Note: A positive slope means that the line has an upward direction, but it does not necessarily mean that the line is steep.
2. Negative Slope
A negative slope indicates that the line falls from left to right. The slope is calculated in the same way as a positive slope, but the result will be a negative number. For example, if a line falls 2 units vertically and moves 5 units horizontally, the slope would be -2⁄5 or -0.4. A negative slope is denoted by a negative number.
3. Zero Slope
A zero slope indicates that the line is horizontal and does not rise or fall. In this case, the vertical change is zero, and the slope is calculated as 0/any number, which equals zero. A zero slope is denoted by a horizontal line.
| Horizontal Change (run) | Vertical Change (rise) | Slope |
|---|---|---|
| 4 | 0 | 0/4 = 0 |
4. Undefined Slope
An undefined slope, also known as an infinite slope, occurs when the horizontal change is zero, and the vertical change is not zero. This type of slope is denoted by a vertical line. In this case, the slope is not defined, as the ratio of vertical change to horizontal change is not a finite number.
🔍 Note: An undefined slope does not mean that the line has no slope, but rather that the slope is not defined in the classical sense.
In conclusion, mastering different types of slopes is essential for working with linear equations and graphing lines. By understanding positive, negative, zero, and undefined slopes, you will be able to analyze and solve a wide range of problems in mathematics.
What is the difference between a positive and negative slope?
+A positive slope indicates that the line rises from left to right, while a negative slope indicates that the line falls from left to right.
Can a line have a zero slope?
+Yes, a line can have a zero slope, which means that the line is horizontal and does not rise or fall.
What is an undefined slope?
+An undefined slope occurs when the horizontal change is zero, and the vertical change is not zero, resulting in a vertical line.
Related Terms:
- Slope worksheet
- Types of slope Notes PDF
- Slope Review worksheet
- Slope triangle worksheet
- Find the slope answer Key
- Rise over run Worksheet PDF