5 Easy Ways to Master Rectangle Perimeter and Area
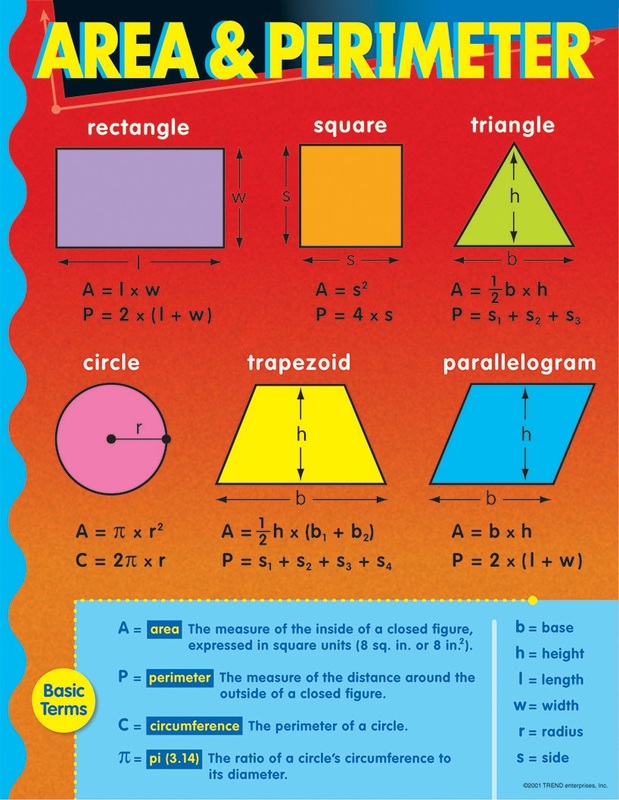
Understanding Rectangle Perimeter and Area
Rectangle perimeter and area are two fundamental concepts in geometry that are used to calculate the distance around and the size of a rectangle, respectively. Mastering these concepts is essential for problem-solving in mathematics, science, and real-life applications. In this article, we will explore 5 easy ways to master rectangle perimeter and area.
Way 1: Learn the Formulas
The first step to mastering rectangle perimeter and area is to learn the formulas. The formula for the perimeter of a rectangle is:
Perimeter = 2(l + w)
where l is the length and w is the width of the rectangle.
The formula for the area of a rectangle is:
Area = l × w
where l is the length and w is the width of the rectangle.
📝 Note: Make sure to memorize these formulas, as they will be used frequently in calculations.
Way 2: Practice with Simple Problems
Practice makes perfect! Start with simple problems to build your confidence and understanding of rectangle perimeter and area. For example:
- Find the perimeter of a rectangle with a length of 6 cm and a width of 4 cm.
- Find the area of a rectangle with a length of 8 cm and a width of 5 cm.
Solve these problems using the formulas, and then check your answers to ensure you are correct.
Way 3: Use Visual Aids
Visual aids such as diagrams and graphs can help you understand and visualize the concepts of rectangle perimeter and area. Draw a rectangle and label its length and width. Then, use the formulas to calculate the perimeter and area.
For example:
| Length (l) | Width (w) | Perimeter | Area |
|---|---|---|---|
| 6 cm | 4 cm | 2(6 + 4) = 20 cm | 6 × 4 = 24 cm² |
| Length (l) | Width (w) | Perimeter | Area |
|---|---|---|---|
| 6 cm | 4 cm | 2(6 + 4) = 20 cm | 6 × 4 = 24 cm² |
Way 4: Apply to Real-Life Scenarios
Apply your knowledge of rectangle perimeter and area to real-life scenarios to make the concepts more meaningful and interesting. For example:
- A room has a rectangular floor with a length of 12 meters and a width of 8 meters. What is the perimeter of the floor? What is the area of the floor?
- A picture frame has a rectangular shape with a length of 20 cm and a width of 15 cm. What is the perimeter of the frame? What is the area of the frame?
🏠 Note: Use everyday objects and situations to practice and apply your knowledge of rectangle perimeter and area.
Way 5: Play Games and Activities
Make learning fun by playing games and activities that involve rectangle perimeter and area. For example:
- Create a rectangle puzzle where students have to find the perimeter and area of a rectangle given its length and width.
- Play a game of “Rectangle Bingo” where students have to calculate the perimeter and area of a rectangle to mark the correct answer on their bingo card.
By incorporating games and activities into your learning, you can make the concepts of rectangle perimeter and area more engaging and enjoyable.
In conclusion, mastering rectangle perimeter and area requires practice, patience, and persistence. By following these 5 easy ways, you can build your confidence and understanding of these fundamental concepts in geometry. Remember to apply your knowledge to real-life scenarios, use visual aids, and make learning fun by playing games and activities.
What is the formula for the perimeter of a rectangle?
+The formula for the perimeter of a rectangle is Perimeter = 2(l + w), where l is the length and w is the width of the rectangle.
What is the formula for the area of a rectangle?
+The formula for the area of a rectangle is Area = l × w, where l is the length and w is the width of the rectangle.
How can I practice and apply my knowledge of rectangle perimeter and area?
+You can practice and apply your knowledge of rectangle perimeter and area by solving simple problems, using visual aids, applying to real-life scenarios, and playing games and activities.