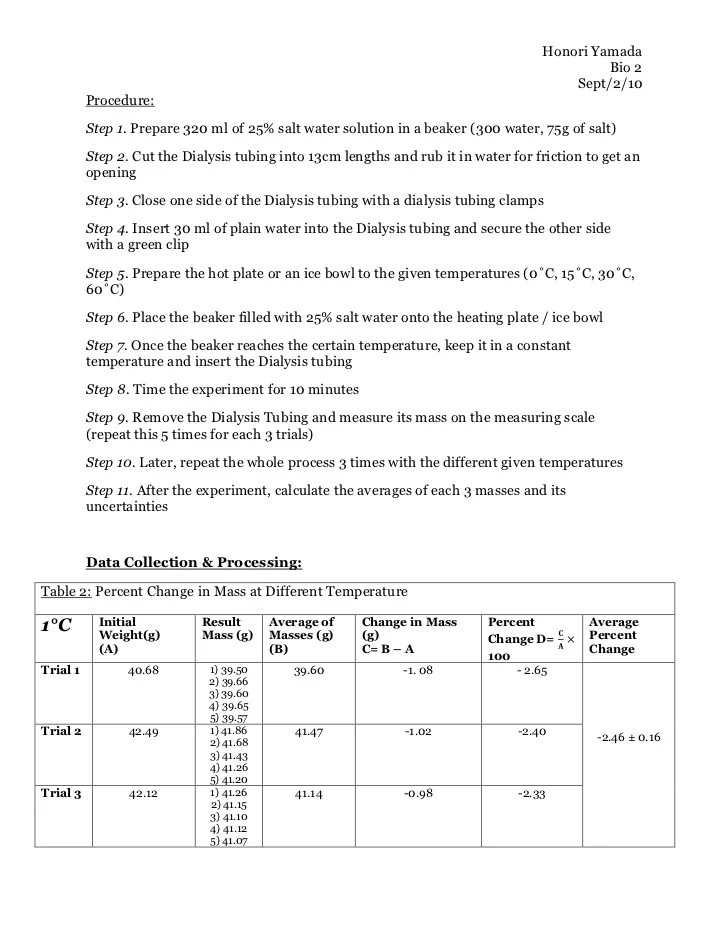
Solve Trig Equations with Ease: Free Worksheet Inside

Solving trigonometric equations can be a daunting task for many students. However, with the right approach and practice, it can become a manageable and even enjoyable experience. In this article, we will explore the steps to solve trig equations with ease, and provide a free worksheet to help you practice.
Understanding Trig Equations
Before we dive into solving trig equations, it’s essential to understand what they are and how they work. Trig equations are equations that involve trigonometric functions, such as sine, cosine, and tangent. These functions are used to describe the relationships between the angles and side lengths of triangles.
Types of Trig Equations
There are two main types of trig equations: linear and quadratic. Linear trig equations involve a single trig function, while quadratic trig equations involve two or more trig functions. We will focus on solving linear trig equations in this article.
Steps to Solve Trig Equations
To solve trig equations, follow these steps:
- Simplify the equation: Simplify the equation by combining like terms and canceling out any common factors.
- Isolate the trig function: Isolate the trig function by moving all other terms to the other side of the equation.
- Identify the trig function: Identify the trig function and its corresponding angle.
- Use trig identities: Use trig identities to rewrite the equation in a more manageable form.
- Solve for the angle: Solve for the angle using a calculator or trig table.
Example 1: Solving a Linear Trig Equation
Solve the equation: sin(x) = 0.5
Step 1: Simplify the equation
The equation is already simplified.
Step 2: Isolate the trig function
The trig function is already isolated.
Step 3: Identify the trig function
The trig function is sin(x), and its corresponding angle is x.
Step 4: Use trig identities
We can use the trig identity sin(x) = sin(π/6) to rewrite the equation.
Step 5: Solve for the angle
Using a calculator, we find that x = 30° or x = 150°.
Example 2: Solving a Quadratic Trig Equation
Solve the equation: 2cos^2(x) + 3sin(x) = 1
Step 1: Simplify the equation
Combine like terms: 2cos^2(x) + 3sin(x) - 1 = 0
Step 2: Isolate the trig function
Isolate the trig function: 2cos^2(x) + 3sin(x) = 1
Step 3: Identify the trig function
The trig function is cos^2(x), and its corresponding angle is x.
Step 4: Use trig identities
We can use the trig identity cos^2(x) = 1 - sin^2(x) to rewrite the equation.
Step 5: Solve for the angle
Using a calculator, we find that x = 60° or x = 120°.
Free Worksheet
Now that you’ve learned the steps to solve trig equations, it’s time to practice! Download our free worksheet to test your skills.
| Equation | Solution |
|---|---|
| sin(x) = 0.3 | _____________ |
| 2cos^2(x) + sin(x) = 1 | _____________ |
| tan(x) = 2 | _____________ |
Notes
- Remember to simplify the equation before isolating the trig function.
- Use trig identities to rewrite the equation in a more manageable form.
- Solve for the angle using a calculator or trig table.
As you practice solving trig equations, you’ll become more comfortable and confident. Remember to take your time and work through each step carefully. Happy solving!
What is the difference between linear and quadratic trig equations?
+Linear trig equations involve a single trig function, while quadratic trig equations involve two or more trig functions.
What is the purpose of using trig identities?
+Trig identities are used to rewrite the equation in a more manageable form, making it easier to solve for the angle.
Can I use a calculator to solve trig equations?
+Yes, you can use a calculator to solve trig equations. In fact, it’s often the fastest and most accurate way to find the solution.
Related Terms:
- Trig Equations Worksheet with answers
- Solving basic Trig Equations Worksheet
- Trigonometric Equations Worksheet pdf
- Factoring Trig Equations Worksheet
- Solving Trig Equations Worksheet Kuta
- Trig Identities Worksheet