Mastering Probability Concepts: Theoretical and Experimental Worksheet
Understanding Probability Concepts
Probability is a measure of the likelihood of an event occurring. It is a fundamental concept in mathematics and statistics, and it has numerous applications in various fields, including science, engineering, economics, and finance. In this article, we will explore the theoretical and experimental aspects of probability, highlighting key concepts, formulas, and examples.
Theoretical Probability
Theoretical probability, also known as classical probability, is based on the number of favorable outcomes divided by the total number of possible outcomes. This approach assumes that each outcome is equally likely to occur.
Formula: P(A) = Number of favorable outcomes / Total number of possible outcomes
Example: A fair six-sided die is rolled. What is the probability of getting a 4?
- Number of favorable outcomes: 1 (getting a 4)
- Total number of possible outcomes: 6 (1, 2, 3, 4, 5, 6)
- P(A) = 1⁄6 ≈ 0.17
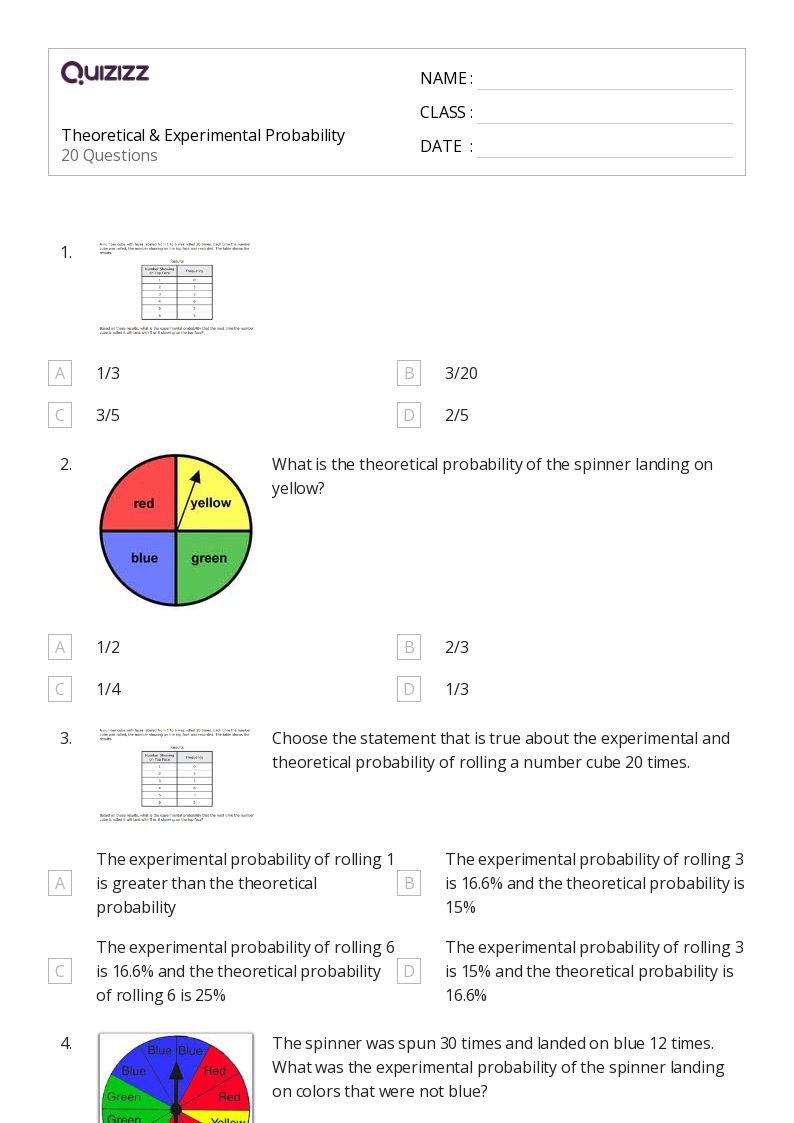
Experimental Probability
Experimental probability, also known as empirical probability, is based on the results of repeated trials or experiments. This approach estimates the probability of an event by analyzing the data collected from the experiments.
Formula: P(A) = Number of times the event occurs / Total number of trials
Example: A coin is flipped 10 times, and the results are: H, T, H, H, T, T, H, T, H, T. What is the probability of getting heads?
- Number of times the event occurs: 5 (getting heads)
- Total number of trials: 10
- P(A) = 5⁄10 = 0.5
Key Concepts and Formulas
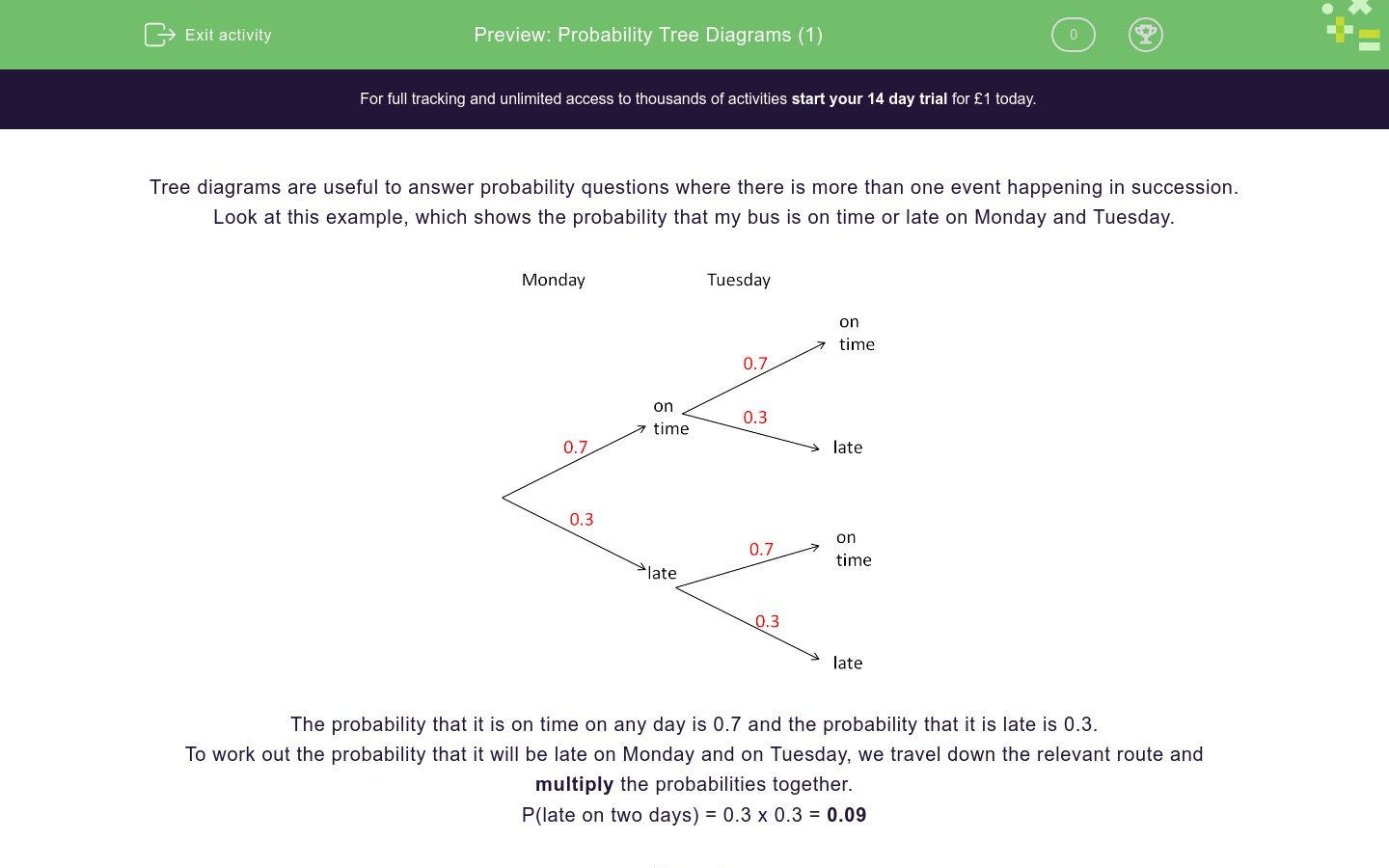
- Independent Events: Events that do not affect each other’s probability. Formula: P(A ∩ B) = P(A) × P(B)
- Dependent Events: Events that affect each other’s probability. Formula: P(A ∩ B) = P(A) × P(B|A)
- Mutually Exclusive Events: Events that cannot occur simultaneously. Formula: P(A ∪ B) = P(A) + P(B)
- Conditional Probability: The probability of an event occurring given that another event has occurred. Formula: P(A|B) = P(A ∩ B) / P(B)
Worksheets and Exercises
To practice and reinforce your understanding of probability concepts, try the following exercises:
- A fair deck of 52 cards is shuffled, and a card is drawn. What is the probability of getting a heart?
- A die is rolled twice. What is the probability of getting a sum of 7?
- A coin is flipped three times. What is the probability of getting two heads and one tail?
- A bag contains 5 red marbles, 3 blue marbles, and 2 green marbles. What is the probability of drawing a red marble?
Solutions:
- P(A) = 13⁄52 = 0.25
- P(A) = 6⁄36 = 0.17
- P(A) = 3⁄8 = 0.38
- P(A) = 5⁄10 = 0.5
💡 Note: The probability values may vary depending on the specific experiment or trial.
Real-World Applications
Probability has numerous applications in various fields, including:
- Insurance: Actuaries use probability to calculate premiums and policy payouts.
- Finance: Investors use probability to estimate the likelihood of stock prices and returns.
- Engineering: Engineers use probability to design and optimize systems, such as traffic flow and queueing systems.
- Medicine: Researchers use probability to estimate the effectiveness of treatments and medications.
Common Probability Mistakes
- Gambler’s Fallacy: Believing that a random event is more likely to happen because it has not happened recently.
- Hot Hand Fallacy: Believing that a random event is more likely to happen because it has happened recently.
- Overconfidence: Overestimating the accuracy of probability estimates.
📝 Note: Avoid these common mistakes by understanding the underlying probability concepts and using data to inform your decisions.
Conclusion
Probability is a fundamental concept in mathematics and statistics, with numerous applications in various fields. By understanding theoretical and experimental probability, key concepts, and formulas, you can make informed decisions and analyze data effectively. Remember to practice and apply probability concepts to real-world scenarios to reinforce your understanding.
What is the difference between theoretical and experimental probability?
+Theoretical probability is based on the number of favorable outcomes divided by the total number of possible outcomes, while experimental probability is based on the results of repeated trials or experiments.
What is the formula for conditional probability?
+P(A|B) = P(A ∩ B) / P(B)
What is the gambler’s fallacy?
+The gambler’s fallacy is the mistaken belief that a random event is more likely to happen because it has not happened recently.
Related Terms:
- Experimental probability Worksheet answers