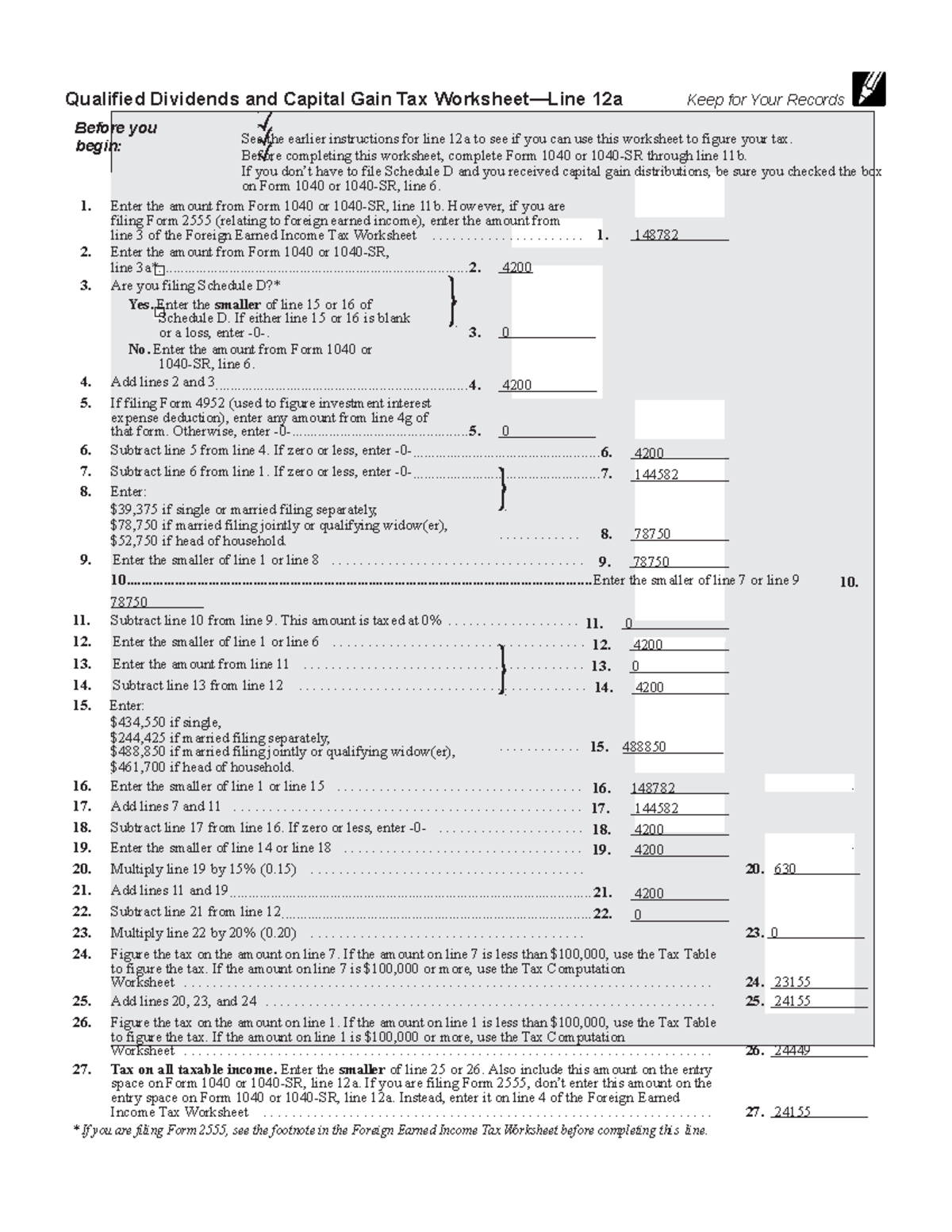
Solve Linear Equations with Ease: Systems Worksheet

Understanding Systems of Linear Equations

Systems of linear equations are a fundamental concept in algebra, and they have numerous applications in various fields, including physics, engineering, and economics. In this article, we will explore the basics of systems of linear equations and provide a comprehensive guide on how to solve them.

What is a System of Linear Equations?

A system of linear equations is a set of two or more linear equations that contain two or more variables. The equations are said to be linear because the highest power of the variables is 1. For example:

2x + 3y = 7 x - 2y = -3

In this example, we have two linear equations with two variables, x and y.

Types of Systems of Linear Equations

There are three types of systems of linear equations:

• Consistent and Independent: This type of system has a unique solution, and the lines intersect at a single point.
• Consistent and Dependent: This type of system has infinitely many solutions, and the lines are identical.
• Inconsistent: This type of system has no solution, and the lines are parallel and never intersect.

Methods for Solving Systems of Linear Equations

There are several methods for solving systems of linear equations, including:

• Substitution Method: This method involves solving one equation for one variable and substituting that expression into the other equation.
• Elimination Method: This method involves adding or subtracting the equations to eliminate one variable.
• Graphical Method: This method involves graphing the equations on a coordinate plane and finding the point of intersection.

Solving Systems of Linear Equations using the Substitution Method

The substitution method involves solving one equation for one variable and substituting that expression into the other equation.

Example:

Solve the system:

x + 2y = 4 3x - 2y = 5

Solution:

Solve the first equation for x:

x = 4 - 2y

Substitute this expression into the second equation:

3(4 - 2y) - 2y = 5

Expand and simplify:

12 - 6y - 2y = 5

Combine like terms:

-8y = -7

Divide by -8:

y = ⁷⁄₈

Now substitute this value back into one of the original equations to find x:

x + 2(⁷⁄₈) = 4

Simplify:

x = ³⁄₂

Therefore, the solution to the system is x = ³⁄₂ and y = ⁷⁄₈.

📝 Note: When using the substitution method, make sure to substitute the expression into the other equation correctly, and simplify carefully to avoid errors.

Solving Systems of Linear Equations using the Elimination Method

The elimination method involves adding or subtracting the equations to eliminate one variable.

Example:

Solve the system:

2x + 3y = 7 x - 2y = -3

Solution:

Add the two equations to eliminate x:

(2x + 3y) + (x - 2y) = 7 + (-3)

Combine like terms:

3x + y = 4

Now solve for x:

x = 4 - y

Substitute this expression into one of the original equations to find y:

2(4 - y) + 3y = 7

Expand and simplify:

8 - 2y + 3y = 7

Combine like terms:

y = 1

Now substitute this value back into one of the original equations to find x:

2x + 3(1) = 7

Simplify:

x = 2

Therefore, the solution to the system is x = 2 and y = 1.

📝 Note: When using the elimination method, make sure to add or subtract the equations correctly, and simplify carefully to avoid errors.

Practice Worksheet

Here is a practice worksheet with 5 systems of linear equations for you to solve:

1. 2x + 3y = 7 x - 2y = -3

2. x + 2y = 4 3x - 2y = 5

3. 2x + 4y = 8 x - 3y = -2

4. x + y = 3 2x - 2y = 0

5. 3x + 2y = 5 x - 4y = -3

📝 Note: Use the substitution or elimination method to solve each system. Make sure to show your work and simplify carefully to avoid errors.

Systems of linear equations are a fundamental concept in algebra, and they have numerous applications in various fields. By understanding the different types of systems and the methods for solving them, you can become proficient in solving systems of linear equations with ease.