Mastering Fractions: Adding and Subtracting with Unlike Denominators

Understanding Fractions with Unlike Denominators

Fractions are a fundamental concept in mathematics, and being able to add and subtract them is a crucial skill. However, things can get tricky when dealing with fractions that have unlike denominators. In this article, we will explore the concept of adding and subtracting fractions with unlike denominators and provide a step-by-step guide on how to master this skill.

The Concept of Unlike Denominators

Unlike denominators refer to fractions that have different denominators. For example, ¹⁄₄ and ¹⁄₆ are fractions with unlike denominators because their denominators are not the same. When adding or subtracting fractions with unlike denominators, we need to find a common denominator that both fractions can share.

Why Finding a Common Denominator is Important

Finding a common denominator is essential when adding or subtracting fractions with unlike denominators. This is because the denominators of the fractions need to be the same in order to perform the operation. If the denominators are not the same, we cannot simply add or subtract the numerators.

📝 Note: The common denominator is also known as the least common multiple (LCM) of the two denominators.

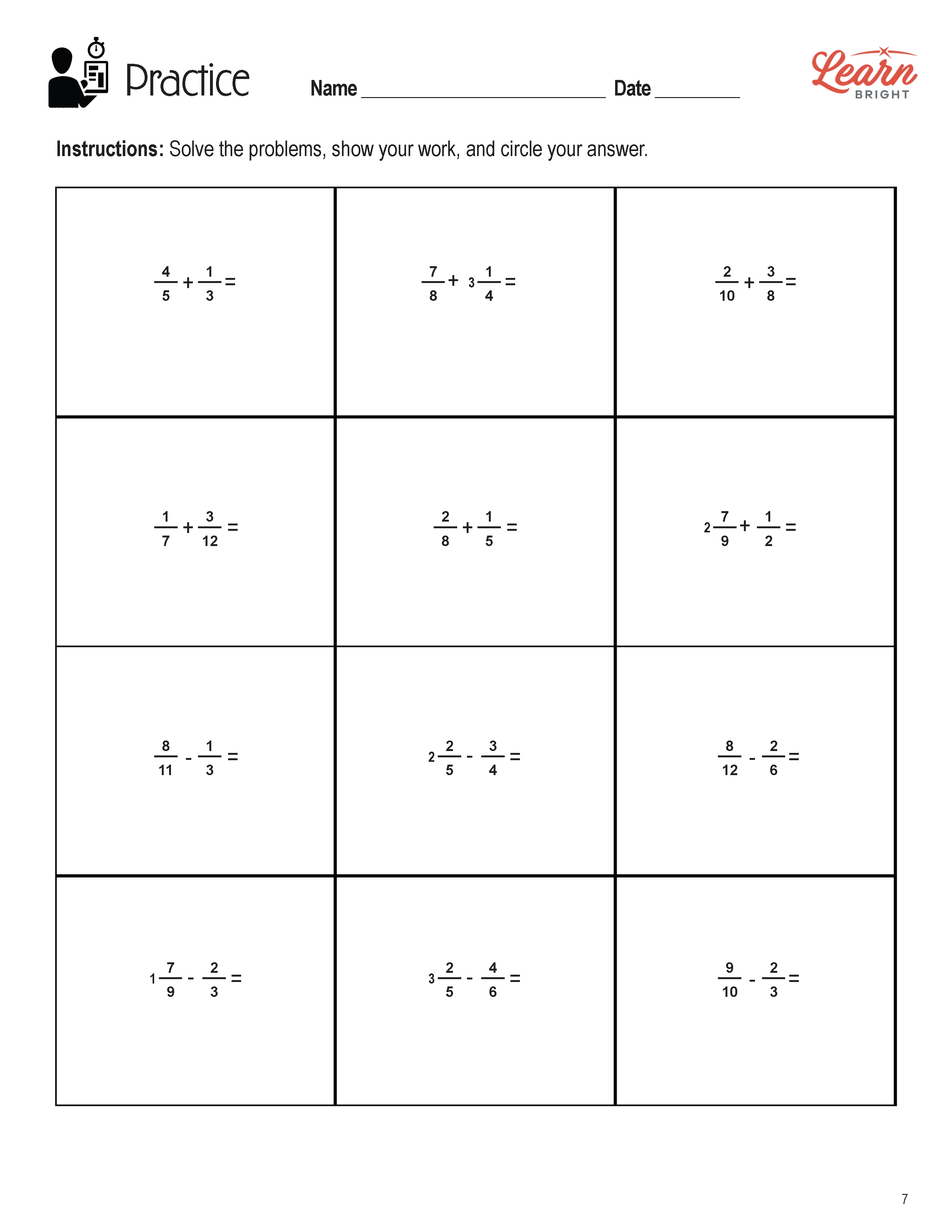
Step-by-Step Guide to Adding Fractions with Unlike Denominators

Here is a step-by-step guide on how to add fractions with unlike denominators:

1. Identify the fractions: Identify the two fractions that you want to add, for example, ¹⁄₄ and ¹⁄₆.
2. Find the common denominator: Find the least common multiple (LCM) of the two denominators, which is the smallest number that both denominators can divide into evenly. In this case, the LCM of 4 and 6 is 12.
3. Convert the fractions: Convert both fractions to have the common denominator. To do this, multiply the numerator and denominator of each fraction by the necessary multiplier.
   • For ¹⁄₄, multiply the numerator and denominator by 3 to get ³⁄₁₂.
   • For ¹⁄₆, multiply the numerator and denominator by 2 to get ²⁄₁₂.
4. Add the fractions: Now that both fractions have the same denominator, you can add them by adding the numerators.
   • ³⁄₁₂ + ²⁄₁₂ = ⁵⁄₁₂

Step-by-Step Guide to Subtracting Fractions with Unlike Denominators

Here is a step-by-step guide on how to subtract fractions with unlike denominators:

1. Identify the fractions: Identify the two fractions that you want to subtract, for example, ²⁄₃ and ³⁄₄.
2. Find the common denominator: Find the least common multiple (LCM) of the two denominators, which is the smallest number that both denominators can divide into evenly. In this case, the LCM of 3 and 4 is 12.
3. Convert the fractions: Convert both fractions to have the common denominator. To do this, multiply the numerator and denominator of each fraction by the necessary multiplier.
   • For ²⁄₃, multiply the numerator and denominator by 4 to get ⁸⁄₁₂.
   • For ³⁄₄, multiply the numerator and denominator by 3 to get ⁹⁄₁₂.
4. Subtract the fractions: Now that both fractions have the same denominator, you can subtract them by subtracting the numerators.
   • ⁸⁄₁₂ - ⁹⁄₁₂ = -¹⁄₁₂

Using Real-World Examples to Illustrate the Concept

Here are a few real-world examples to illustrate the concept of adding and subtracting fractions with unlike denominators:

• Cooking: A recipe calls for ¹⁄₄ cup of flour and ¹⁄₆ cup of sugar. If you want to add these two ingredients together, you need to find a common denominator, which is 12. You can then convert both fractions to have a denominator of 12 and add them together.
• Building: A carpenter needs to cut two pieces of wood, one that is ²⁄₃ of a foot long and another that is ³⁄₄ of a foot long. If the carpenter wants to know the total length of wood needed, they need to subtract the two fractions. To do this, they need to find a common denominator, which is 12.

Conclusion

Adding and subtracting fractions with unlike denominators is a crucial skill in mathematics. By following the step-by-step guides outlined in this article, you can master this skill and become proficient in working with fractions. Remember to always find the common denominator and convert both fractions to have the same denominator before performing the operation.