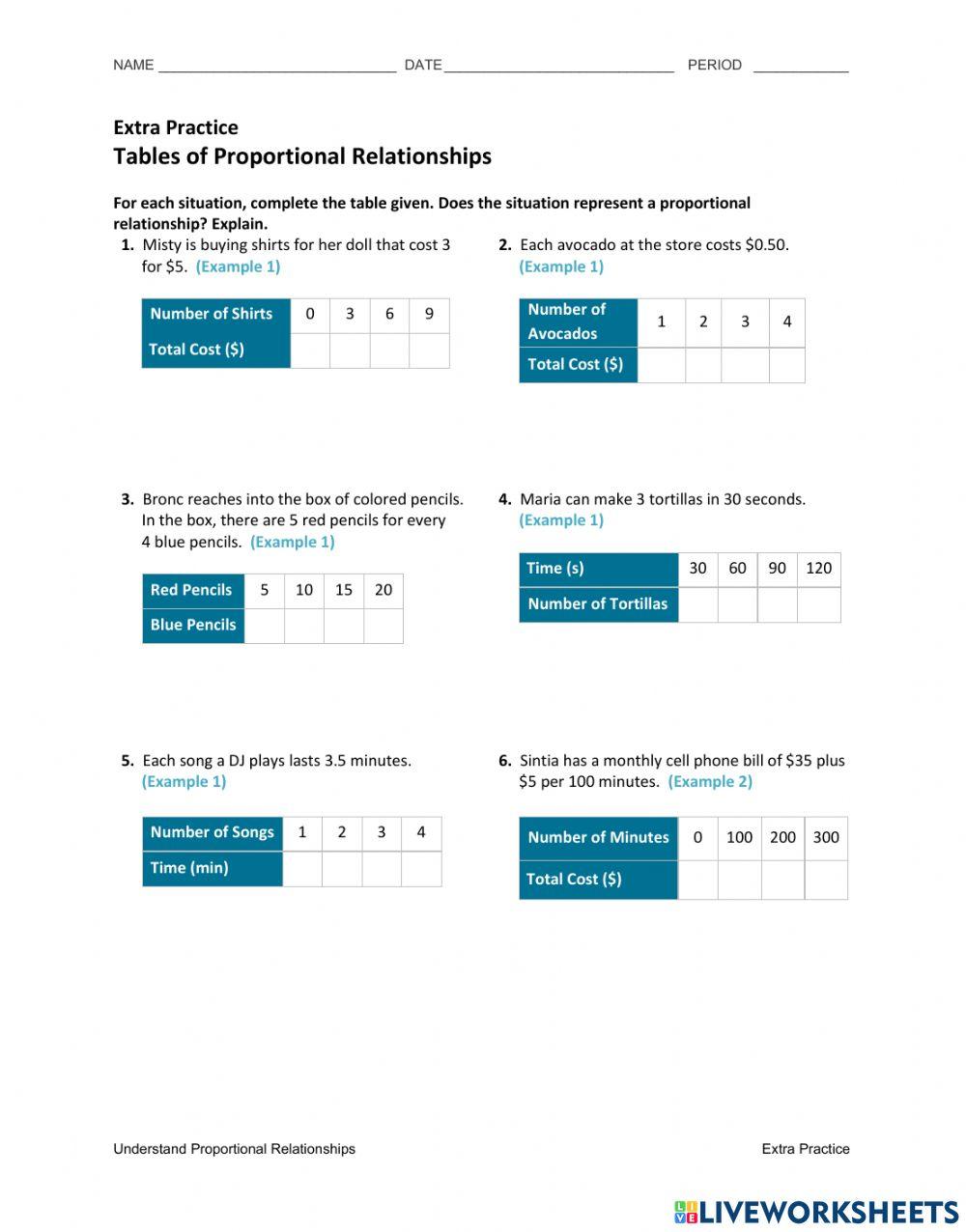
Convert to Slope Intercept Form Easily with This Worksheet
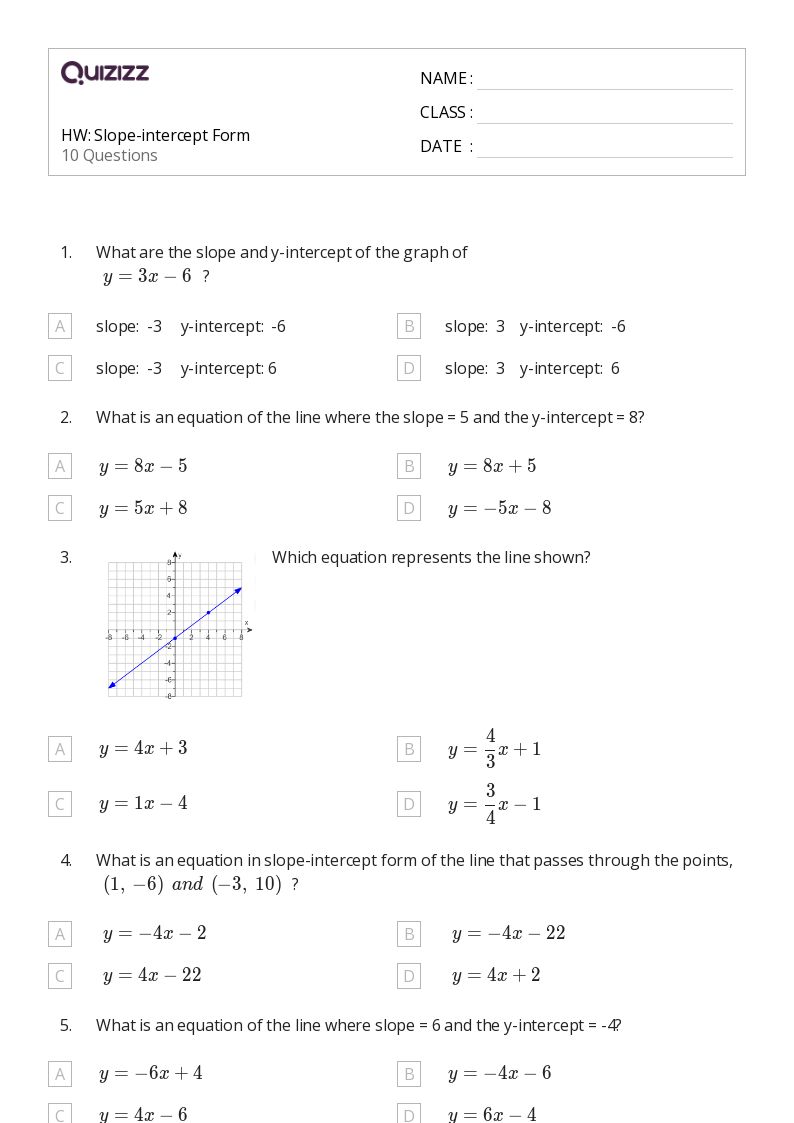
Understanding Slope-Intercept Form
In mathematics, particularly in algebra and geometry, slope-intercept form is a way to express the equation of a linear line. It is one of the most commonly used forms of linear equations. The slope-intercept form is denoted as y = mx + b, where m represents the slope of the line, and b is the y-intercept.
📝 Note: The y-intercept is the point at which the line crosses the y-axis.
Why Convert to Slope-Intercept Form?
Converting to slope-intercept form is useful because it allows us to easily identify the slope and y-intercept of a line. This information can be used to graph the line, write equations of lines, and solve systems of equations.
How to Convert to Slope-Intercept Form
Converting to slope-intercept form involves rearranging the equation to isolate y. Here are the steps to follow:
- Write the equation in its original form.
- Add or subtract the same value to both sides of the equation to isolate the term with y.
- Divide both sides of the equation by the coefficient of y to solve for y.
Let’s use an example to illustrate this process:
Example 1: Convert the equation 2x + 3y = 7 to slope-intercept form.

| Step | Equation |
|---|---|
| Original form | 2x + 3y = 7 |
| Add -2x to both sides | 3y = -2x + 7 |
| Divide by 3 | y = (-2⁄3)x + 7⁄3 |
Therefore, the equation 2x + 3y = 7 in slope-intercept form is y = (-2⁄3)x + 7⁄3.
Example 2: Convert the equation x - 2y = -3 to slope-intercept form.
| Step | Equation |
|---|---|
| Original form | x - 2y = -3 |
| Add 2y to both sides | x = 2y - 3 |
| Subtract x from both sides | 2y = x + 3 |
| Divide by 2 | y = (1⁄2)x + 3⁄2 |
Therefore, the equation x - 2y = -3 in slope-intercept form is y = (1⁄2)x + 3⁄2.
Common Mistakes to Avoid
When converting to slope-intercept form, be careful to avoid the following common mistakes:
- Incorrect signs: Make sure to distribute the negative signs correctly when adding or subtracting values to both sides of the equation.
- Incorrect coefficients: Double-check that you are dividing both sides of the equation by the correct coefficient of y.
- Omitting steps: Make sure to complete all the necessary steps to isolate y.
Practice with This Worksheet
Practice converting equations to slope-intercept form with the following worksheet:
| Equation | Slope-Intercept Form |
|---|---|
| x + 4y = 9 | |
| 2x - 3y = -2 | |
| 3x + 2y = 7 | |
| x - 5y = -1 | |
| 4x + y = 10 |
Fill in the answers to the worksheet to practice converting equations to slope-intercept form.
What is the purpose of converting to slope-intercept form?
+Converting to slope-intercept form allows us to easily identify the slope and y-intercept of a line, which is useful for graphing lines, writing equations of lines, and solving systems of equations.
How do I convert an equation to slope-intercept form?
+To convert an equation to slope-intercept form, follow these steps: (1) Write the equation in its original form, (2) Add or subtract the same value to both sides of the equation to isolate the term with y, and (3) Divide both sides of the equation by the coefficient of y to solve for y.
What are some common mistakes to avoid when converting to slope-intercept form?
+Some common mistakes to avoid when converting to slope-intercept form include incorrect signs, incorrect coefficients, and omitting steps. Make sure to distribute negative signs correctly, divide by the correct coefficient of y, and complete all necessary steps to isolate y.
In summary, converting to slope-intercept form is an essential skill in mathematics that allows us to easily identify the slope and y-intercept of a line. By following the steps outlined in this post and practicing with the worksheet, you can master the skill of converting equations to slope-intercept form.
Related Terms:
- Slope intercept form Worksheet Kuta