5 Tips to Solve Two-Step Inequalities

Understanding Two-Step Inequalities
Inequalities are a fundamental concept in mathematics, and solving them is an essential skill for any math enthusiast. Two-step inequalities, in particular, can be a bit tricky, but with the right approach, you can master them. In this article, we will provide you with 5 valuable tips to help you solve two-step inequalities with ease.
Tip 1: Identify the Variable and the Inequality Sign
When dealing with two-step inequalities, it’s crucial to identify the variable and the inequality sign. The variable is the letter or symbol that represents the unknown value, and the inequality sign indicates the relationship between the variable and the constant term.
For example, consider the inequality 2x + 5 > 11. In this case, x is the variable, and > is the inequality sign.
📝 Note: Make sure to read the inequality sign carefully, as it can change the direction of the solution.
Tip 2: Isolate the Variable by Performing the First Operation
To solve a two-step inequality, you need to isolate the variable by performing the first operation. This usually involves adding, subtracting, multiplying, or dividing both sides of the inequality by a constant term.
For instance, let’s solve the inequality 2x + 5 > 11. To isolate the variable, we need to subtract 5 from both sides:
2x + 5 - 5 > 11 - 5
This simplifies to:
2x > 6
Tip 3: Perform the Second Operation to Isolate the Variable Completely
Once you’ve performed the first operation, you’ll need to perform the second operation to isolate the variable completely. This usually involves dividing or multiplying both sides of the inequality by a coefficient.
Using the same example, we need to divide both sides by 2 to isolate the variable:
2x / 2 > 6 / 2
This simplifies to:
x > 3
Tip 4: Consider the Direction of the Inequality Sign
When solving two-step inequalities, it’s essential to consider the direction of the inequality sign. If the inequality sign is > or ≥, you’ll need to perform the operations in the same direction. However, if the inequality sign is < or ≤, you’ll need to perform the operations in the opposite direction.
For example, consider the inequality x - 3 < 5. To solve this, we need to add 3 to both sides, but because the inequality sign is <, we’ll need to perform the operation in the opposite direction:
x - 3 + 3 < 5 + 3
This simplifies to:
x < 8
Tip 5: Check Your Solution by Plugging it Back into the Original Inequality
Finally, it’s crucial to check your solution by plugging it back into the original inequality. This ensures that your solution satisfies the original inequality.
Using the same example, we can plug x = 4 into the original inequality x - 3 < 5:
4 - 3 < 5
This simplifies to:
1 < 5
Since this is true, our solution x = 4 satisfies the original inequality.
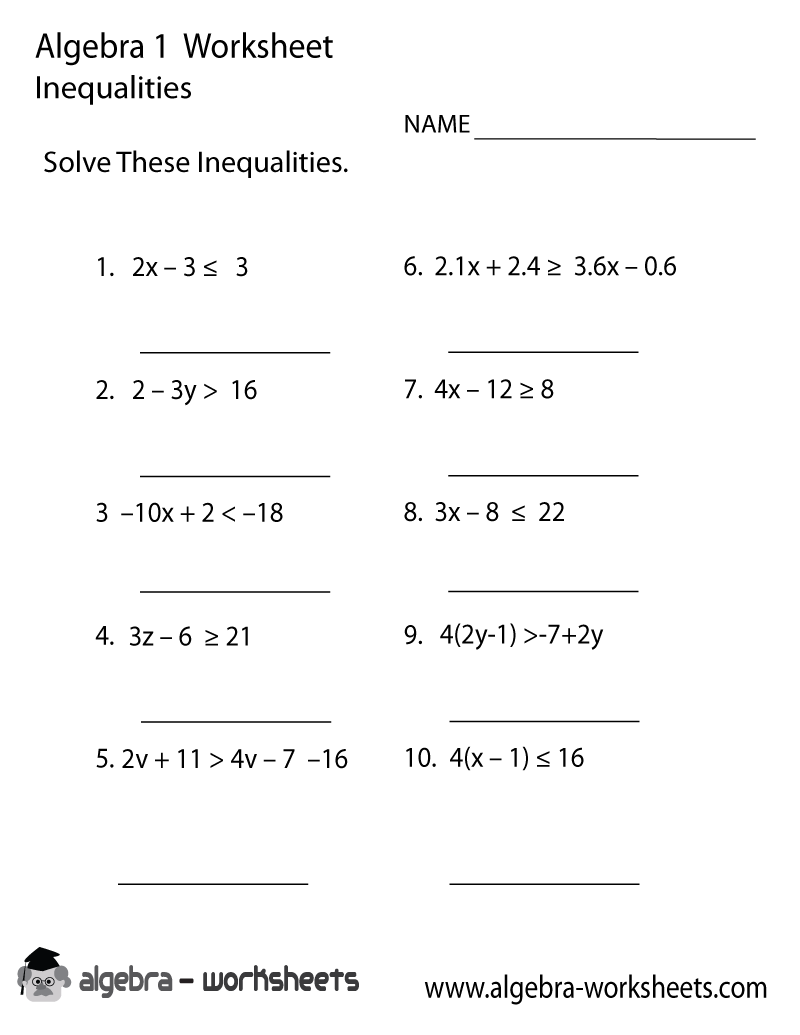
| Two-Step Inequality | Solution |
|---|---|
| 2x + 5 > 11 | x > 3 |
| x - 3 < 5 | x < 8 |
| 3x + 2 ≥ 14 | x ≥ 4 |
By following these 5 tips, you’ll be able to solve two-step inequalities with confidence. Remember to identify the variable and inequality sign, isolate the variable by performing the first operation, perform the second operation to isolate the variable completely, consider the direction of the inequality sign, and check your solution by plugging it back into the original inequality.
With practice and patience, you’ll become proficient in solving two-step inequalities and develop a solid foundation for more advanced math concepts.
What is the difference between a one-step and a two-step inequality?
+A one-step inequality requires only one operation to solve, whereas a two-step inequality requires two operations to isolate the variable.
How do I know which operation to perform first when solving a two-step inequality?
+You should perform the operation that isolates the variable term, usually by adding, subtracting, multiplying, or dividing both sides of the inequality by a constant term.
Can I solve two-step inequalities with fractions or decimals?
+Yes, you can solve two-step inequalities with fractions or decimals by following the same steps and operations as with whole numbers.
Related Terms:
- Multi step Inequalities Worksheet PDF
- two-step inequalities worksheet 7th grade
- Solving one-Step Inequalities Worksheet PDF
- Solving Inequalities Worksheet PDF