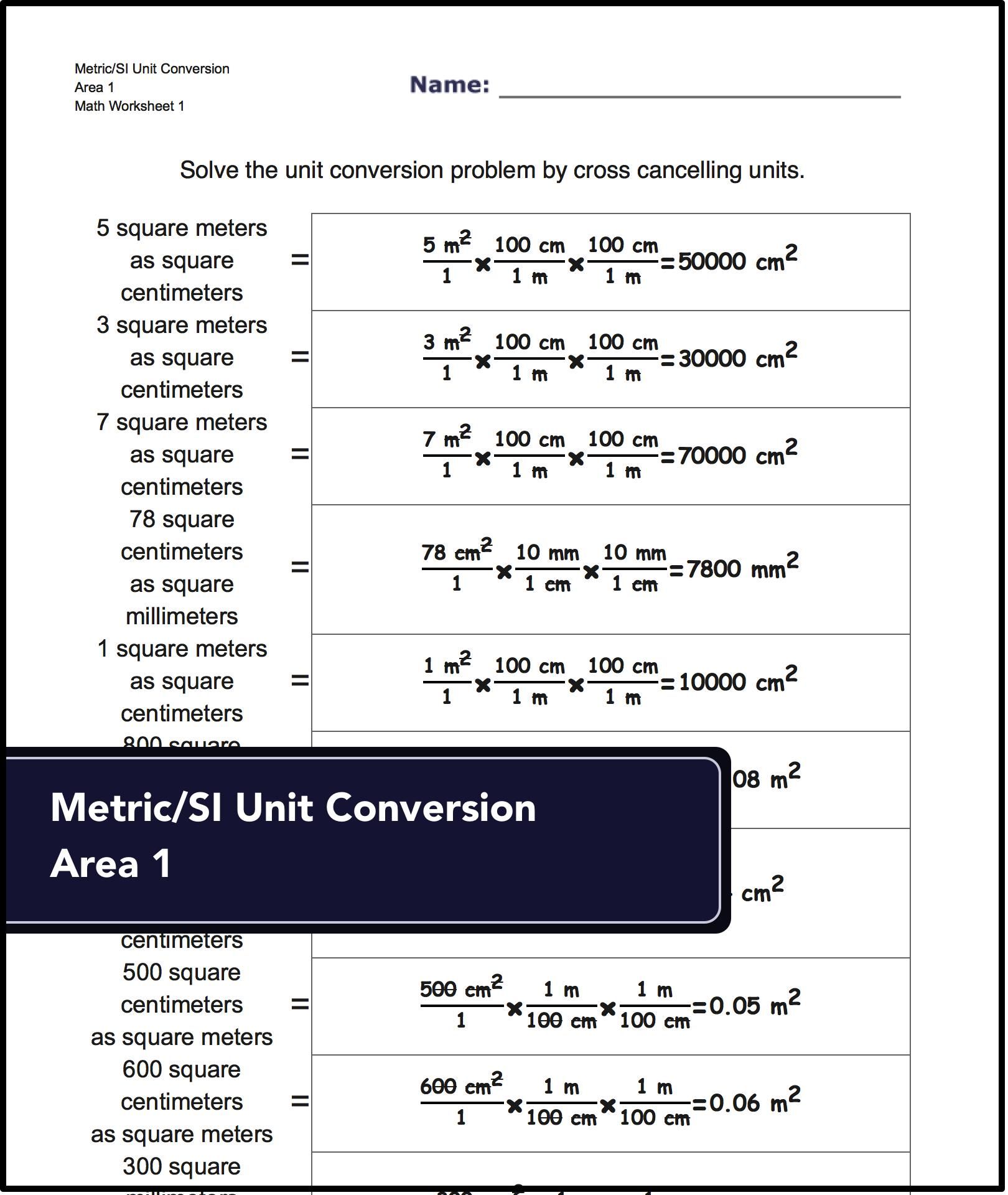
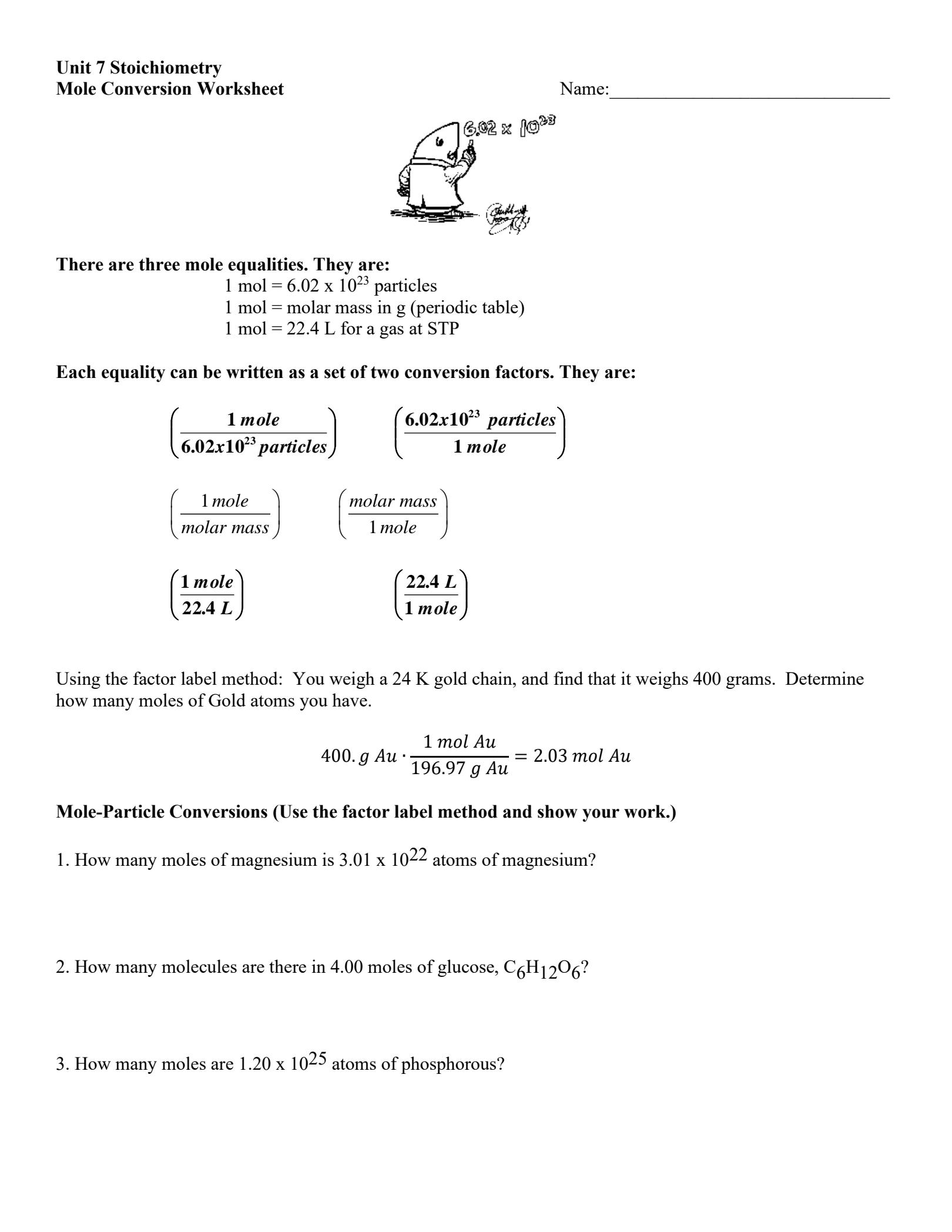
7 Essential Trigonometry Problems Solved
Understanding Trigonometry: A Comprehensive Guide
Trigonometry is a fundamental branch of mathematics that deals with the relationships between the sides and angles of triangles. It has numerous applications in various fields, including physics, engineering, and navigation. In this article, we will delve into seven essential trigonometry problems and provide step-by-step solutions to help you grasp the concepts better.
Problem 1: Finding the Length of a Side in a Right Triangle
Given a right triangle with an acute angle of 30° and a hypotenuse of 10 cm, find the length of the side opposite the angle.
Solution:
Using the sine function, we can write:
sin(30°) = opposite side / hypotenuse
We know that sin(30°) = 0.5, and the hypotenuse is 10 cm. Plugging in these values, we get:
0.5 = opposite side / 10
To find the length of the opposite side, we can multiply both sides by 10:
opposite side = 0.5 × 10 opposite side = 5 cm
Therefore, the length of the side opposite the 30° angle is 5 cm.
Problem 2: Finding the Angle in a Right Triangle
Given a right triangle with a side opposite the angle of 6 cm and a hypotenuse of 10 cm, find the angle.
Solution:
Using the sine function, we can write:
sin(angle) = opposite side / hypotenuse
We know that the opposite side is 6 cm, and the hypotenuse is 10 cm. Plugging in these values, we get:
sin(angle) = 6 / 10 sin(angle) = 0.6
To find the angle, we can take the inverse sine (sin^(-1)) of both sides:
angle = sin^(-1)(0.6)
Using a calculator, we find that the angle is approximately 36.87°.
Problem 3: Finding the Length of a Side in an Oblique Triangle
Given an oblique triangle with an angle of 60°, a side adjacent to the angle of 8 cm, and a side opposite the angle of 12 cm, find the length of the third side.
Solution:
Using the Law of Cosines, we can write:
c² = a² + b² - 2ab × cos©
where c is the length of the third side, a and b are the lengths of the other two sides, and C is the angle between them.
Plugging in the values, we get:
c² = 8² + 12² - 2(8)(12) × cos(60°) c² = 64 + 144 - 192 × 0.5 c² = 208 - 96 c² = 112
Taking the square root of both sides, we get:
c = √112 c ≈ 10.6 cm
Therefore, the length of the third side is approximately 10.6 cm.
Problem 4: Finding the Angle in an Oblique Triangle
Given an oblique triangle with sides of 8 cm, 12 cm, and 15 cm, find the angle opposite the 12 cm side.
Solution:
Using the Law of Cosines, we can write:
c² = a² + b² - 2ab × cos©
where c is the length of the side opposite the angle, a and b are the lengths of the other two sides, and C is the angle between them.
Plugging in the values, we get:
12² = 8² + 15² - 2(8)(15) × cos© 144 = 64 + 225 - 240 × cos© 144 = 289 - 240 × cos©
Simplifying and rearranging, we get:
240 × cos© = 289 - 144 240 × cos© = 145
Dividing both sides by 240, we get:
cos© = 145 / 240 cos© ≈ 0.6042
Taking the inverse cosine (cos^(-1)) of both sides, we get:
C = cos^(-1)(0.6042) C ≈ 52.51°
Therefore, the angle opposite the 12 cm side is approximately 52.51°.
Problem 5: Finding the Area of a Triangle
Given a triangle with a base of 10 cm and a height of 6 cm, find the area.
Solution:
Using the formula for the area of a triangle, we can write:
Area = (1⁄2) × base × height Area = (1⁄2) × 10 × 6 Area = 30 cm²
Therefore, the area of the triangle is 30 cm².
Problem 6: Finding the Volume of a Tetrahedron
Given a tetrahedron with a base area of 20 cm² and a height of 8 cm, find the volume.
Solution:
Using the formula for the volume of a tetrahedron, we can write:
Volume = (1⁄3) × base area × height Volume = (1⁄3) × 20 × 8 Volume = 53.33 cm³
Therefore, the volume of the tetrahedron is approximately 53.33 cm³.
Problem 7: Finding the Length of a Side in a Right Triangle using the Pythagorean Theorem
Given a right triangle with a hypotenuse of 10 cm and a side opposite the angle of 6 cm, find the length of the adjacent side.
Solution:
Using the Pythagorean Theorem, we can write:
a² + b² = c²
where a is the length of the adjacent side, b is the length of the opposite side, and c is the length of the hypotenuse.
Plugging in the values, we get:
a² + 6² = 10² a² + 36 = 100
Simplifying and rearranging, we get:
a² = 100 - 36 a² = 64
Taking the square root of both sides, we get:
a = √64 a = 8 cm
Therefore, the length of the adjacent side is 8 cm.
🔍 Note: The Pythagorean Theorem can only be used for right triangles.
In conclusion, these seven essential trigonometry problems demonstrate the importance of understanding the relationships between the sides and angles of triangles. By applying various formulas and theorems, such as the sine and cosine functions, the Law of Cosines, and the Pythagorean Theorem, we can solve a wide range of problems in trigonometry.
What is trigonometry?
+
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles.
What is the Law of Cosines?
+
The Law of Cosines is a formula that relates the lengths of the sides of a triangle to the cosine of one of its angles.
What is the Pythagorean Theorem?
+
The Pythagorean Theorem is a formula that relates the lengths of the sides of a right triangle.
Related Terms:
- Sin cos tan Worksheet Kuta
- Sine ratio Worksheet
- Sin cos tan worksheet corbettmaths
- Sin cos tan table
- Sin, cos tan triangle