Sequences of Transformations Worksheet
Understanding Sequences of Transformations
Transformations are a fundamental concept in geometry, and understanding how to apply them in sequence is crucial for solving complex problems. In this article, we will explore the concept of sequences of transformations, how to apply them, and provide a worksheet to help you practice.
What are Sequences of Transformations?
A sequence of transformations is a series of transformations applied to a figure in a specific order. The transformations can include translations, rotations, reflections, and dilations. Each transformation is applied to the resulting figure from the previous transformation.
Types of Transformations
There are four main types of transformations:
- Translation: A translation is a transformation that moves a figure from one location to another without changing its size or shape.
- Rotation: A rotation is a transformation that turns a figure around a fixed point, called the center of rotation.
- Reflection: A reflection is a transformation that flips a figure over a line, called the line of reflection.
- Dilation: A dilation is a transformation that changes the size of a figure.
Applying Sequences of Transformations
To apply a sequence of transformations, follow these steps:
- Identify the transformations to be applied and their order.
- Apply the first transformation to the original figure.
- Apply the second transformation to the resulting figure from the first transformation.
- Continue applying the remaining transformations in the specified order.
- The final figure is the result of the sequence of transformations.
Examples of Sequences of Transformations
Here are a few examples of sequences of transformations:
- Translation + Rotation: Translate a figure 5 units to the right, then rotate it 90 degrees counterclockwise.
- Reflection + Dilation: Reflect a figure over the x-axis, then dilate it by a scale factor of 2.
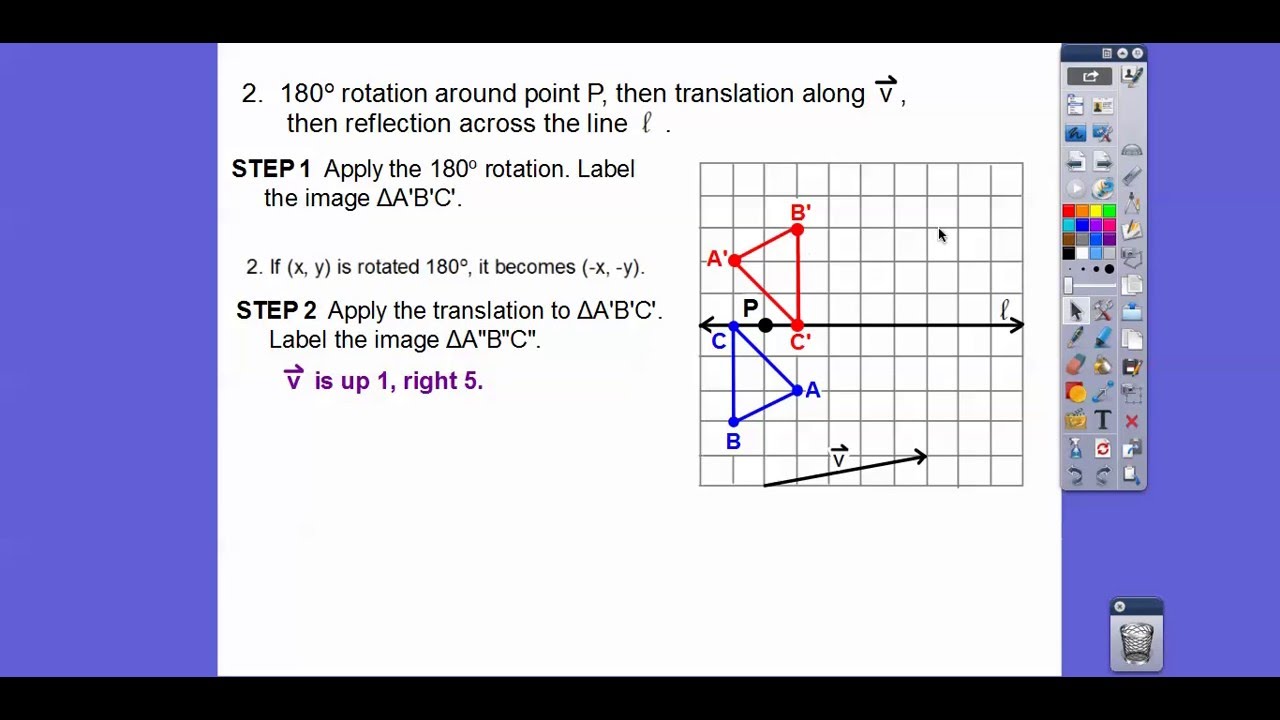
- Rotation + Translation: Rotate a figure 180 degrees clockwise, then translate it 3 units up.
Worksheet: Sequences of Transformations
Try the following exercises to practice applying sequences of transformations:
| Original Figure | Sequence of Transformations | Resulting Figure |
|---|---|---|
| Triangle ABC | Translate 2 units left, then rotate 120 degrees clockwise | |
| Quadrilateral DEFG | Reflect over the y-axis, then dilate by a scale factor of 1.5 | |
| Pentagon HIJKL | Rotate 90 degrees counterclockwise, then translate 4 units right |
📝 Note: Use graph paper to help you visualize the transformations and draw the resulting figures.
Conclusion
Sequences of transformations are an essential concept in geometry, and understanding how to apply them is crucial for solving complex problems. By practicing with the worksheet above, you can develop your skills and become more confident in applying sequences of transformations.
What is a sequence of transformations?
+A sequence of transformations is a series of transformations applied to a figure in a specific order.
What are the four main types of transformations?
+The four main types of transformations are translation, rotation, reflection, and dilation.
How do I apply a sequence of transformations?
+To apply a sequence of transformations, identify the transformations to be applied and their order, then apply each transformation in sequence to the resulting figure from the previous transformation.
Related Terms:
- Teacher synergy llc
- Khan Academy
- IXL
- BrainPOP
- Udacity
- Duolingo