Multiply with Ease: Scientific Notation Worksheet

Multiplying numbers in scientific notation can seem daunting at first, but with practice and the right techniques, it can become second nature. In this article, we will explore the basics of multiplying numbers in scientific notation and provide a comprehensive worksheet to help you master this essential math skill.

What is Scientific Notation?

Scientific notation is a way of expressing very large or very small numbers in a more manageable form. It consists of a coefficient (a number between 1 and 10) multiplied by a power of 10. For example, the number 456,000,000 can be written in scientific notation as 4.56 x 10^8.

How to Multiply Numbers in Scientific Notation

Multiplying numbers in scientific notation involves multiplying the coefficients and adding the exponents. Here are the steps:

1. Multiply the coefficients.
2. Add the exponents.

Let’s look at an example:

(2.5 x 10^4) x (3.2 x 10^2)

1. Multiply the coefficients: 2.5 x 3.2 = 8
2. Add the exponents: 10^4 x 10^2 = 10^(4+2) = 10^6

The answer is 8 x 10^6.

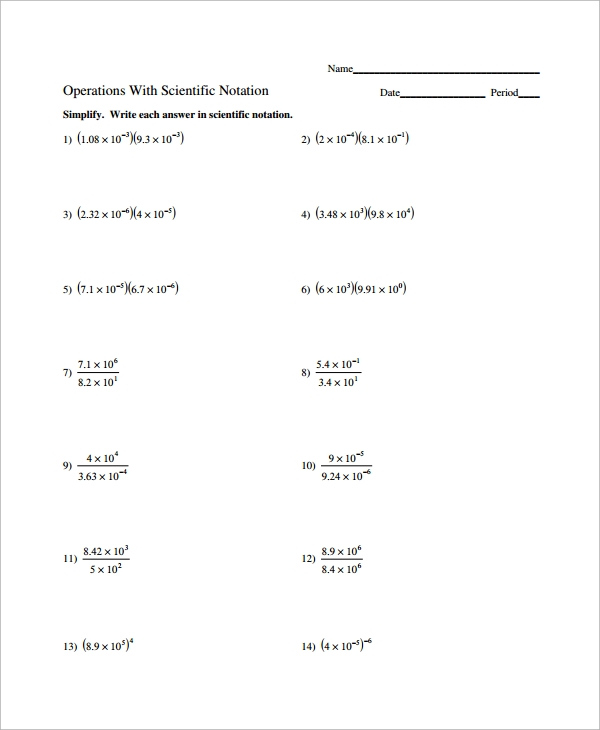
Worksheet: Multiplying Numbers in Scientific Notation

Here is a comprehensive worksheet to help you practice multiplying numbers in scientific notation:

Section 1: Simple Multiplication

1. (2 x 10^3) x (4 x 10^2) =
2. (5 x 10^2) x (2 x 10^4) =
3. (3 x 10^5) x (2 x 10^1) =
4. (4 x 10^1) x (3 x 10^3) =
5. (2 x 10^2) x (5 x 10^1) =

Section 2: Multiplication with Different Exponents

1. (2.5 x 10^4) x (3.2 x 10^2) =
2. (4.1 x 10^3) x (2.9 x 10^5) =
3. (1.8 x 10^2) x (3.1 x 10^4) =
4. (2.9 x 10^1) x (4.2 x 10^3) =
5. (3.5 x 10^5) x (2.1 x 10^2) =

Section 3: Multiplication with Zero Exponents

1. (2 x 10^0) x (4 x 10^2) =
2. (5 x 10^0) x (2 x 10^4) =
3. (3 x 10^0) x (2 x 10^1) =
4. (4 x 10^0) x (3 x 10^3) =
5. (2 x 10^0) x (5 x 10^1) =

Section 4: Word Problems

1. A car travels 2.5 x 10^4 miles in 3.2 x 10^2 days. How many miles does it travel per day?
2. A bakery sells 4.1 x 10^3 loaves of bread per day. If each loaf costs $2.9 x 10^1, how much money does the bakery make in a day?
3. A scientist measures the length of a cell to be 1.8 x 10^2 micrometers. If the cell is divided into 3.1 x 10^4 parts, what is the length of each part?

Answer Key

Section 1: Simple Multiplication

1. 8 x 10^5
2. 10 x 10^6
3. 6 x 10^6
4. 12 x 10^4
5. 10 x 10^3

Section 2: Multiplication with Different Exponents

1. 8 x 10^6
2. 12 x 10^8
3. 5.58 x 10^6
4. 12 x 10^4
5. 7.35 x 10^7

Section 3: Multiplication with Zero Exponents

1. 8 x 10^2
2. 10 x 10^4
3. 6 x 10^1
4. 12 x 10^3
5. 10 x 10^1

Section 4: Word Problems

1. 78.12 miles per day
2. $1.198 x 10^4 per day
3. 5.8 x 10^2 micrometers per part

Tips and Tricks

• Always multiply the coefficients first.
• Add the exponents carefully.
• Make sure to line up the decimal points when multiplying coefficients.
• Use a calculator to check your answers.

In Conclusion

Multiplying numbers in scientific notation is a valuable skill that can help you solve complex math problems with ease. With practice and patience, you can master this skill and become more confident in your math abilities. Remember to always follow the rules of multiplying coefficients and adding exponents, and don’t be afraid to use a calculator to check your answers.

Frequently Asked Questions

Related Terms:

• Scientific notation Multiplication Worksheet
• Division of Scientific Notation Worksheet