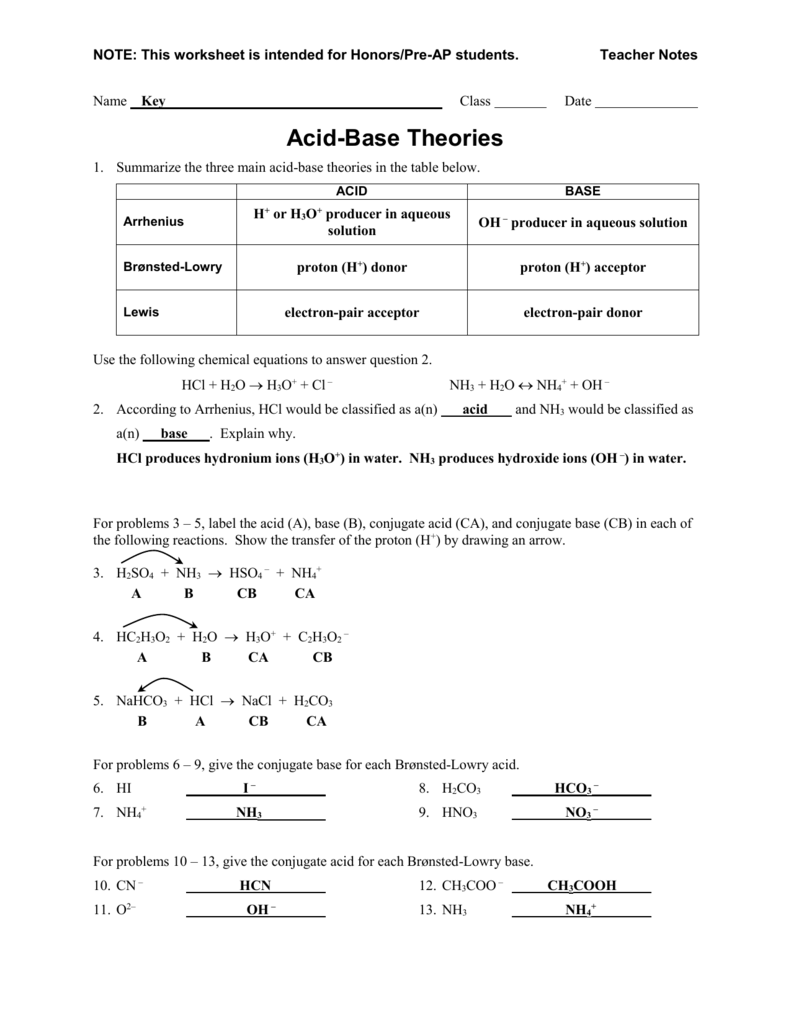
5 Ways to Master Quadrilateral Proofs
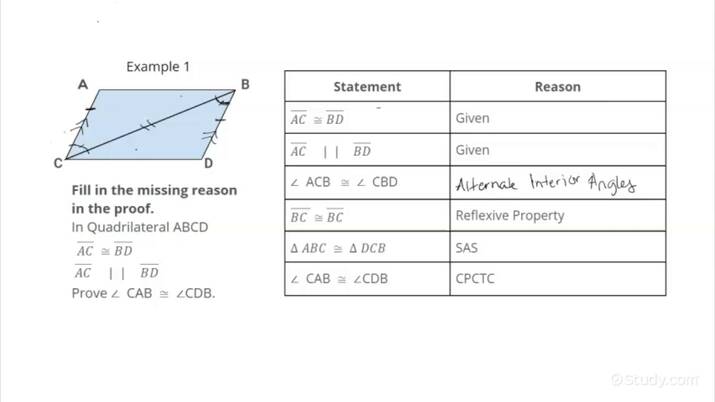
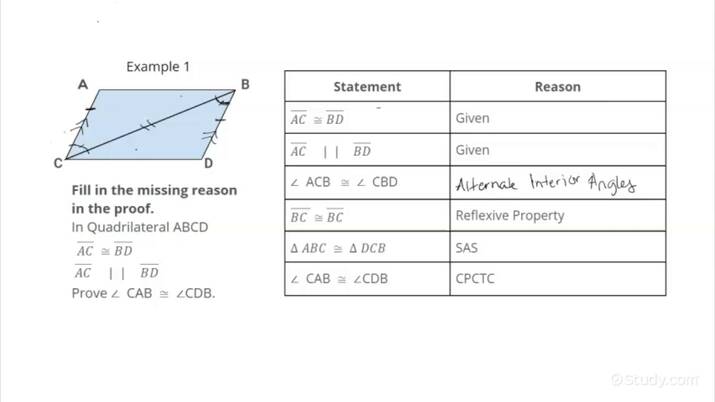
Understanding Quadrilateral Proofs
Quadrilateral proofs can be a daunting task for many students, but with a solid understanding of the basics and a few expert tips, anyone can master them. In this article, we’ll explore five ways to improve your skills and become a pro at quadrilateral proofs.
1. Know Your Quadrilateral Properties
Before diving into proofs, it’s essential to understand the properties of different types of quadrilaterals. Here are some key properties to remember:
- Parallelograms: Opposite sides are parallel and congruent, opposite angles are congruent, and consecutive angles are supplementary.
- Rectangles: All properties of parallelograms, plus all angles are right angles.
- Rhombuses: All sides are congruent, opposite angles are congruent, and consecutive angles are supplementary.
- Squares: All properties of rhombuses, plus all angles are right angles.
- Trapezoids: One pair of opposite sides is parallel, and the non-parallel sides are called legs.
- Isosceles Trapezoids: A trapezoid with congruent legs.
📝 Note: Make sure to review the properties of each type of quadrilateral before starting a proof.
2. Use the Given Information Wisely
When working on a proof, it’s crucial to carefully read and understand the given information. Identify what’s given and what needs to be proven. Use the given information to your advantage by:
- Drawing diagrams: Visualize the problem by drawing a diagram. This will help you understand the relationships between the different parts of the quadrilateral.
- Labeling parts: Label the vertices, sides, and angles to help you keep track of the different components.
- Using given measurements: Use any given measurements, such as side lengths or angle measures, to help you prove the required statements.
3. Choose the Right Theorems and Corollaries
Quadrilateral proofs often require the use of various theorems and corollaries. Familiarize yourself with the following:
- Parallelogram Theorem: If both pairs of opposite sides of a quadrilateral are congruent, then the quadrilateral is a parallelogram.
- Rectangle Theorem: If a quadrilateral has three right angles, then it is a rectangle.
- Rhombus Theorem: If all sides of a quadrilateral are congruent, then it is a rhombus.
- Trapezoid Theorem: If a quadrilateral has one pair of opposite sides that is parallel, then it is a trapezoid.
📚 Note: Make sure to understand the conditions and conclusions of each theorem and corollary.
4. Prove It Step by Step
Breaking down the proof into smaller, manageable steps is essential. Follow these steps:
- Start with the given information: Begin by restating the given information and identifying what needs to be proven.
- Use theorems and corollaries: Apply relevant theorems and corollaries to prove the required statements.
- Use logical reasoning: Use logical reasoning to connect the steps and arrive at the final conclusion.
- Check your work: Review your proof to ensure that it is logical and complete.
5. Practice, Practice, Practice
The key to mastering quadrilateral proofs is practice. Try solving different types of problems and proofs to become more comfortable with the material. Here are some tips to keep in mind:
- Start with simple proofs: Begin with basic proofs and gradually move on to more complex ones.
- Use online resources: Utilize online resources, such as video tutorials and practice problems, to supplement your learning.
- Join a study group: Collaborate with classmates or friends to work on proofs and learn from one another.
| Quadrilateral Type | Properties | Theorems and Corollaries |
|---|---|---|
| Parallelogram | Opposite sides are parallel and congruent, opposite angles are congruent | Parallelogram Theorem |
| Rectangle | All properties of parallelograms, plus all angles are right angles | Rectangle Theorem |
| Rhombus | All sides are congruent, opposite angles are congruent | Rhombus Theorem |
| Trapezoid | One pair of opposite sides is parallel | Trapezoid Theorem |
By following these five tips and practicing regularly, you’ll become proficient in quadrilateral proofs and be able to tackle even the most challenging problems with confidence.
In conclusion, mastering quadrilateral proofs requires a combination of understanding properties, using the right theorems and corollaries, and practicing regularly. With dedication and persistence, anyone can become a pro at quadrilateral proofs and excel in mathematics.
What is the difference between a parallelogram and a rectangle?
+A parallelogram has opposite sides that are parallel and congruent, while a rectangle is a type of parallelogram with all right angles.
How do I prove that a quadrilateral is a rhombus?
+To prove that a quadrilateral is a rhombus, you need to show that all sides are congruent and opposite angles are congruent.
What is the trapezoid theorem?
+The trapezoid theorem states that if a quadrilateral has one pair of opposite sides that is parallel, then it is a trapezoid.
Related Terms:
- Quadrilateral Proofs worksheet with answers
- Quadrilateral Proofs worksheet pdf
- Quadrilateral proofs practice
- Proofs involving quadrilaterals
- Parallelogram proofs Worksheet with answers