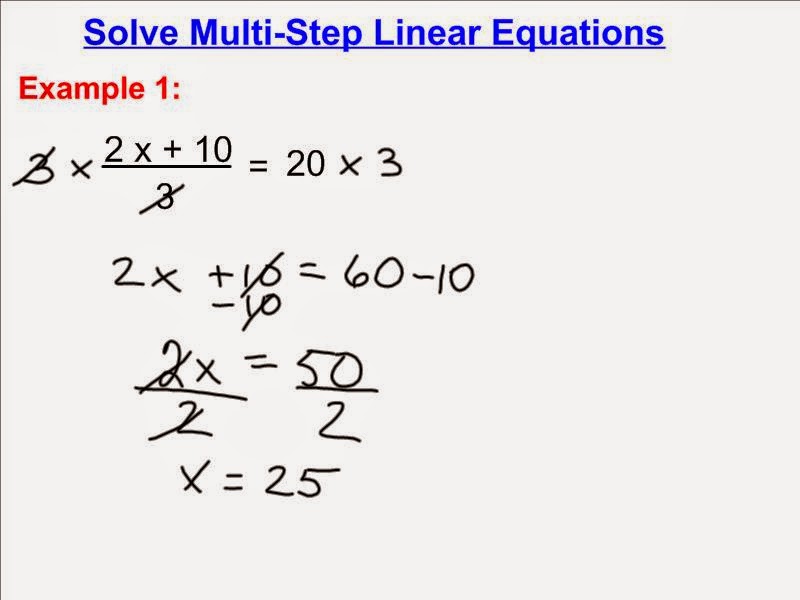
Solve with Ease: Multistep Equation Worksheet Help

Understanding the Basics of Multistep Equations
Multistep equations are a fundamental concept in mathematics, and mastering them is crucial for success in algebra and beyond. These equations require you to perform a series of operations to solve for the unknown variable. In this blog post, we will delve into the world of multistep equations, exploring the basics, solving strategies, and providing a worksheet to help you practice.
What are Multistep Equations?
A multistep equation is an equation that requires more than one step to solve. These equations often involve a combination of addition, subtraction, multiplication, and division operations. The goal is to isolate the variable, usually represented by a letter such as x or y, by performing a series of inverse operations.
Key Concepts to Remember
Before we dive into solving multistep equations, it’s essential to recall some key concepts:
- Inverse operations: These are operations that “undo” each other. For example, addition and subtraction are inverse operations, as are multiplication and division.
- Order of operations: This is a set of rules that dictates the order in which operations should be performed when there are multiple operations in an expression. The acronym PEMDAS is commonly used to remember the order: Parentheses, Exponents, Multiplication and Division, and Addition and Subtraction.
- Distributive property: This property states that a single value can be distributed to multiple values within parentheses.
Solving Multistep Equations: A Step-by-Step Guide
Now that we’ve covered the basics, let’s move on to solving multistep equations. Here’s a step-by-step guide to help you get started:
- Read the equation carefully: Take a moment to understand what the equation is asking. Identify the variable and the operations involved.
- Simplify the equation: Look for any opportunities to simplify the equation by combining like terms or removing parentheses.
- Perform inverse operations: Identify the operations that need to be “undone” to isolate the variable. For example, if the variable is multiplied by a coefficient, you’ll need to divide both sides of the equation by that coefficient.
- Use the distributive property: If the variable is within parentheses, use the distributive property to expand the expression.
- Check your work: Once you’ve solved the equation, plug the solution back into the original equation to ensure it’s true.
Common Challenges and Solutions
When solving multistep equations, you may encounter some common challenges. Here are a few solutions to help you overcome them:
- Dealing with negative numbers: When working with negative numbers, remember to keep track of the negative signs. A negative times a negative equals a positive, while a negative times a positive equals a negative.
- Working with fractions: When working with fractions, remember to find a common denominator before adding or subtracting. You can also multiply both sides of the equation by the least common multiple (LCM) to eliminate the fractions.
Multistep Equation Worksheet
Now that we’ve covered the basics and common challenges, it’s time to practice! Here’s a worksheet with 10 multistep equations for you to solve:
| Equation | Solution |
|---|---|
| 2x + 5 = 11 | |
| x - 3 = 7 | |
| 4x = 28 | |
| x/2 + 2 = 9 | |
| 3x - 2 = 14 | |
| 2x + 2 = 16 | |
| x + 4 = 19 | |
| 5x = 35 | |
| x - 1 = 10 | |
| 3x + 1 = 22 |
📝 Note: Remember to check your work by plugging the solution back into the original equation.
As you work through the worksheet, take your time and remember to apply the strategies we’ve discussed. With practice, you’ll become more confident and proficient in solving multistep equations.
In conclusion, solving multistep equations requires patience, attention to detail, and a solid understanding of mathematical concepts. By following the step-by-step guide and practicing with the worksheet, you’ll be well on your way to mastering these equations. Remember to stay calm, take your time, and don’t be afraid to ask for help when needed.
What is the difference between a single-step equation and a multistep equation?
+A single-step equation requires only one operation to solve, while a multistep equation requires a series of operations to solve.
How do I know which operation to perform first when solving a multistep equation?
+Use the order of operations (PEMDAS) to determine which operation to perform first. If there are multiple operations at the same level (e.g., multiplication and division), perform the operation that will isolate the variable first.
Can I use a calculator to solve multistep equations?
+While a calculator can be helpful, it’s essential to understand the mathematical concepts behind solving multistep equations. Try to solve the equations on your own before using a calculator to check your work.
Related Terms:
- Multi Step Equation Worksheet