Subtracting Mixed Numbers Made Easy With Like Denominators
Understanding the Basics of Subtracting Mixed Numbers
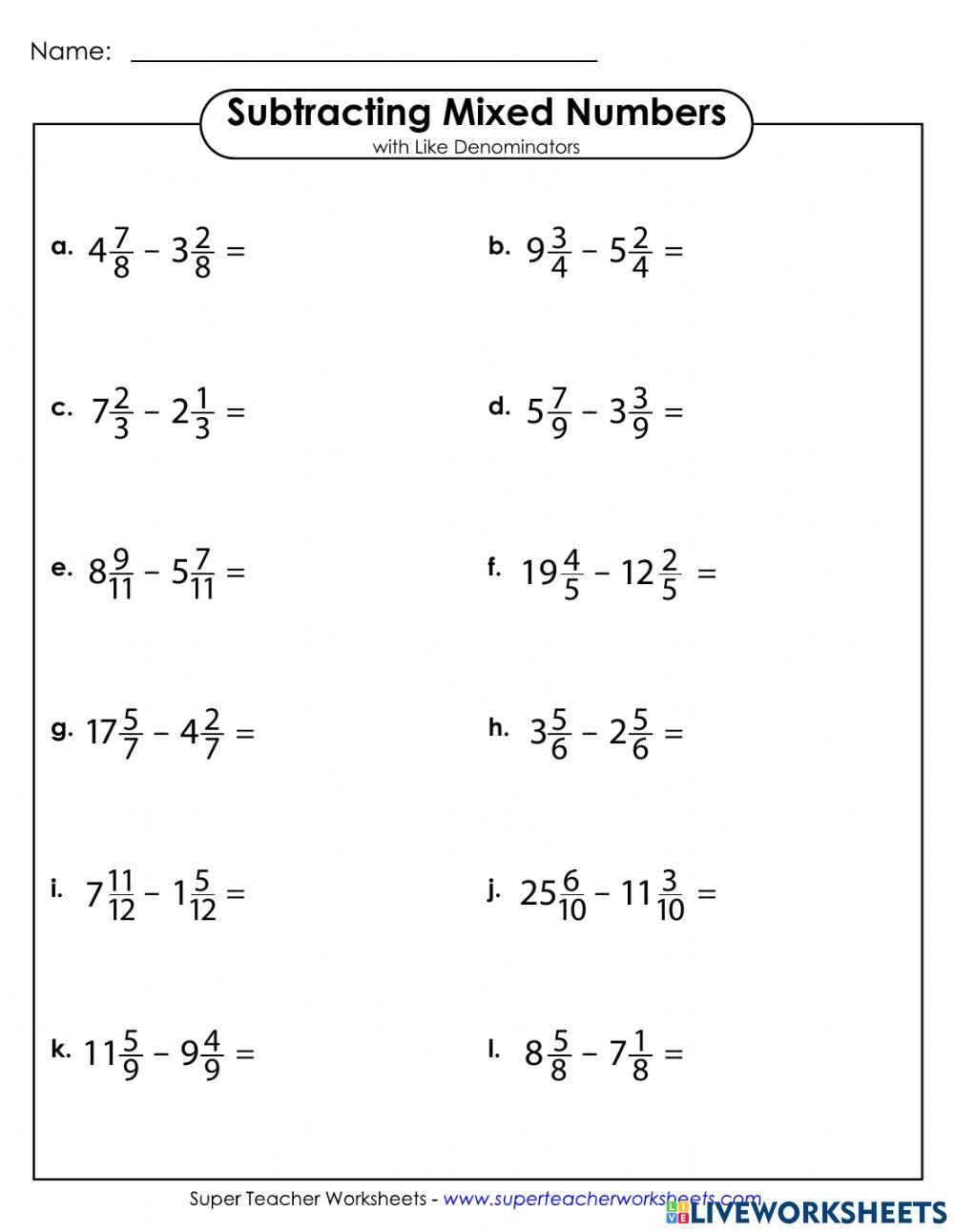
When it comes to subtracting mixed numbers, many students feel anxious or unsure about how to approach the problem. However, with a solid understanding of the basics and a step-by-step guide, subtracting mixed numbers can become a breeze. In this article, we will focus on subtracting mixed numbers with like denominators, which is a crucial skill to master in mathematics.
What are Mixed Numbers?
Before we dive into subtracting mixed numbers, let’s quickly review what mixed numbers are. A mixed number is a combination of a whole number and a fraction. For example, 2 1⁄2 is a mixed number, where 2 is the whole number and 1⁄2 is the fraction. Mixed numbers are commonly used in real-life scenarios, such as measuring ingredients for a recipe or calculating distances.
What are Like Denominators?
Like denominators refer to fractions that have the same denominator. For example, 1⁄4 and 3⁄4 are like fractions because they both have the same denominator, which is 4. When subtracting mixed numbers with like denominators, the process is relatively straightforward.
Step-by-Step Guide to Subtracting Mixed Numbers with Like Denominators
Here’s a step-by-step guide to subtracting mixed numbers with like denominators:
- Step 1: Write the mixed numbers with like denominators. For example, let’s say we want to subtract 2 1⁄4 from 3 3⁄4.
- Step 2: Convert the mixed numbers to improper fractions. To do this, multiply the whole number by the denominator and add the numerator. Then, write the result over the denominator. For example:
- 2 1⁄4 = (2 x 4) + 1 = 9⁄4
- 3 3⁄4 = (3 x 4) + 3 = 15⁄4
- Step 3: Subtract the fractions. Since the denominators are the same, we can subtract the numerators directly. For example:
- 15⁄4 - 9⁄4 = 6⁄4
- Step 4: Simplify the result. If possible, simplify the resulting fraction by dividing the numerator and denominator by the greatest common divisor (GCD). In this case, we can simplify 6⁄4 by dividing both numbers by 2, resulting in 3⁄2.
- Step 5: Write the result as a mixed number (optional). If desired, convert the improper fraction back to a mixed number. For example, 3⁄2 can be written as 1 1⁄2.
📝 Note: When subtracting mixed numbers with like denominators, make sure to follow the order of operations (PEMDAS) and perform any necessary calculations before subtracting the fractions.
Examples and Practice Exercises
To reinforce your understanding, let’s practice subtracting mixed numbers with like denominators using some examples:

| Example | Solution |
|---|---|
| 2 1⁄6 - 1 3⁄6 | 1 4⁄6 = 1 2⁄3 |
| 3 2⁄8 - 2 5⁄8 | 1 3⁄8 |
| 4 3⁄12 - 2 9⁄12 | 1 6⁄12 = 1 1⁄2 |
Try working through these examples on your own and then check your answers against the solutions provided.
Common Mistakes to Avoid
When subtracting mixed numbers with like denominators, be mindful of the following common mistakes:
- Forgetting to convert the mixed numbers to improper fractions
- Subtracting the numerators without considering the denominators
- Failing to simplify the resulting fraction
- Not following the order of operations (PEMDAS)
By avoiding these common mistakes and following the step-by-step guide outlined above, you’ll be well on your way to mastering the skill of subtracting mixed numbers with like denominators.
To recap, subtracting mixed numbers with like denominators involves converting the mixed numbers to improper fractions, subtracting the fractions, simplifying the result, and writing the answer as a mixed number (if desired). With practice and patience, you’ll become more confident and proficient in your ability to subtract mixed numbers with like denominators.
What is the difference between a mixed number and an improper fraction?
+
A mixed number is a combination of a whole number and a fraction, while an improper fraction is a fraction where the numerator is greater than the denominator.
Can I subtract mixed numbers with unlike denominators?
+
No, when subtracting mixed numbers, the denominators must be the same. If the denominators are different, you’ll need to find the least common multiple (LCM) before subtracting.
How do I simplify a fraction?
+
To simplify a fraction, divide the numerator and denominator by the greatest common divisor (GCD). If there is no common divisor, the fraction is already in its simplest form.