5 Ways to Master the Pythagorean Theorem
Unlocking the Secrets of the Pythagorean Theorem
The Pythagorean theorem is a fundamental concept in geometry and mathematics, enabling us to calculate the length of the hypotenuse of a right-angled triangle. This theorem has been widely used for centuries, and its applications extend beyond mathematics to various fields, including physics, engineering, and architecture. In this article, we will delve into the world of the Pythagorean theorem and explore five ways to master this essential mathematical concept.
Understanding the Theorem
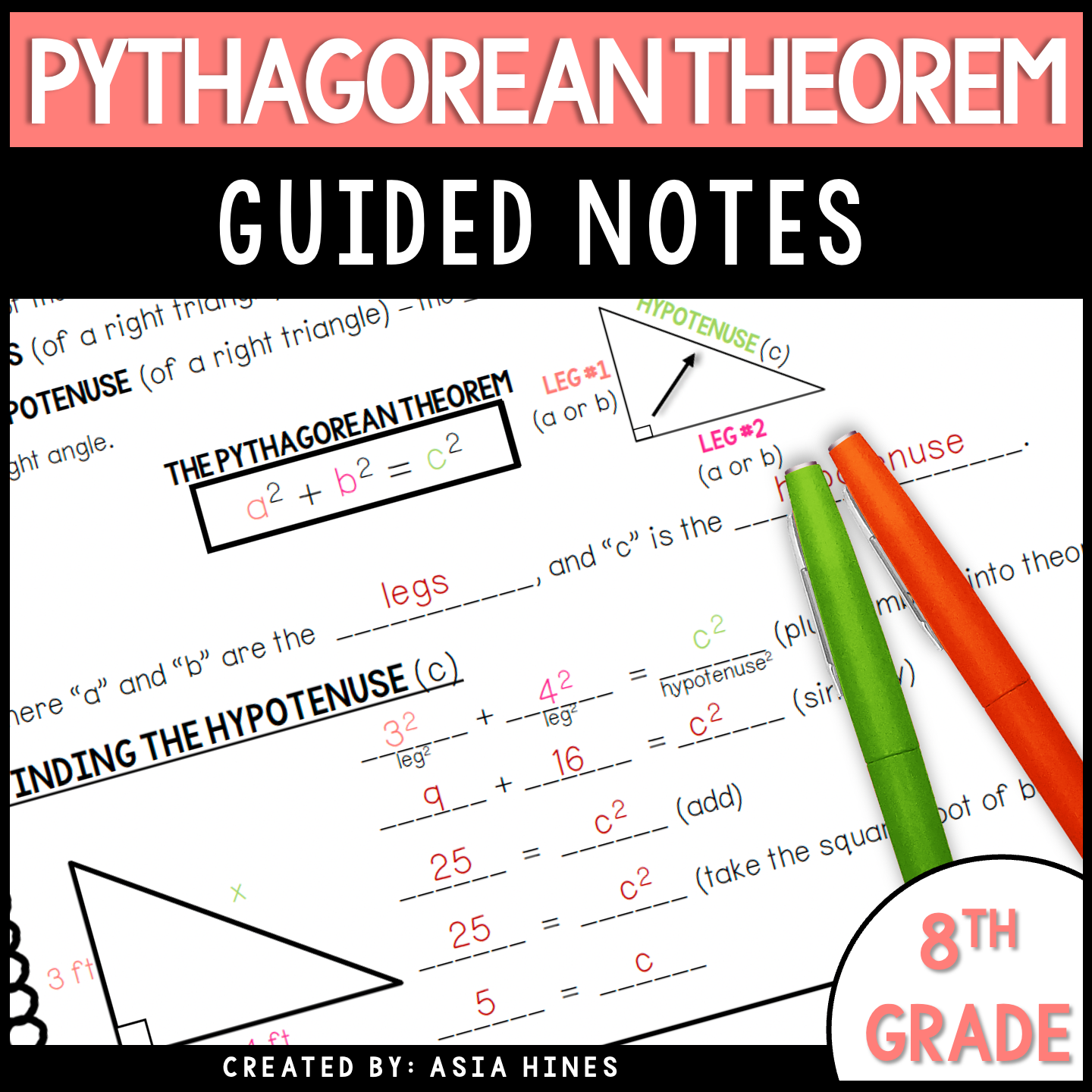
The Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This can be expressed mathematically as:
a^2 + b^2 = c^2
where a and b are the lengths of the two sides containing the right angle, and c is the length of the hypotenuse.
1. Visualizing the Theorem
One of the most effective ways to understand and master the Pythagorean theorem is to visualize it. By drawing diagrams and using graphical representations, you can see how the theorem works and how it can be applied to different types of triangles. Here are some tips for visualizing the theorem:
- Draw a right-angled triangle with the sides labeled as a, b, and c.
- Use a ruler or a straightedge to draw a square on each side of the triangle.
- Shade the squares to help you visualize the area of each square.
- Use the shaded squares to calculate the sum of the squares of the lengths of the two sides (a^2 + b^2).
- Compare the result to the square of the length of the hypotenuse (c^2).
By visualizing the theorem in this way, you can gain a deeper understanding of how it works and how it can be applied to different types of problems.
2. Practicing with Examples
Another way to master the Pythagorean theorem is to practice using it to solve examples. Here are a few examples to get you started:
- In a right-angled triangle, the length of one side is 3 inches and the length of the other side is 4 inches. What is the length of the hypotenuse?
- In a right-angled triangle, the length of the hypotenuse is 10 cm and one side is 6 cm. What is the length of the other side?
- In a right-angled triangle, the length of one side is 5 meters and the length of the other side is 12 meters. What is the length of the hypotenuse?
By working through these examples, you can gain a better understanding of how the theorem works and how it can be applied to different types of problems.
3. Using Real-World Applications
The Pythagorean theorem has numerous real-world applications, including physics, engineering, and architecture. By exploring these applications, you can gain a deeper understanding of the theorem and how it can be used in practical contexts. Here are a few examples:
- In physics, the Pythagorean theorem is used to calculate the distance between objects in space.
- In engineering, the theorem is used to design buildings and bridges.
- In architecture, the theorem is used to calculate the height of buildings and the length of shadows.
By exploring these real-world applications, you can see how the Pythagorean theorem is used in practical contexts and gain a deeper understanding of its importance.
4. Using Online Resources
There are many online resources available to help you master the Pythagorean theorem. Here are a few examples:
- Khan Academy: This website offers a range of video tutorials and practice exercises to help you learn the Pythagorean theorem.
- Mathway: This website offers an online calculator that can help you solve Pythagorean theorem problems.
- GeoGebra: This website offers interactive math software that can help you visualize and explore the Pythagorean theorem.
By using these online resources, you can gain access to a range of learning materials and practice exercises to help you master the Pythagorean theorem.
5. Creating Flashcards
Finally, creating flashcards is a great way to master the Pythagorean theorem. By creating flashcards with key terms and formulas, you can review and practice the theorem in a fun and interactive way. Here are some tips for creating flashcards:
- Write the formula for the Pythagorean theorem on one side of the card (a^2 + b^2 = c^2).
- Write a key term or concept on the other side of the card (e.g. “hypotenuse” or “right-angled triangle”).
- Use the flashcards to quiz yourself on the theorem and its applications.
- Review the flashcards regularly to reinforce your learning.
By creating flashcards, you can review and practice the Pythagorean theorem in a fun and interactive way.
Summing up, mastering the Pythagorean theorem requires a combination of visualization, practice, and application. By using the techniques outlined in this article, you can gain a deeper understanding of the theorem and its applications, and become proficient in using it to solve a range of problems.
What is the Pythagorean theorem?
+The Pythagorean theorem is a mathematical concept that states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
How can I apply the Pythagorean theorem in real-world contexts?
+The Pythagorean theorem has numerous real-world applications, including physics, engineering, and architecture. By exploring these applications, you can see how the theorem is used in practical contexts and gain a deeper understanding of its importance.
What are some online resources available to help me master the Pythagorean theorem?
+There are many online resources available to help you master the Pythagorean theorem, including Khan Academy, Mathway, and GeoGebra. By using these resources, you can gain access to a range of learning materials and practice exercises to help you master the theorem.
Related Terms:
- Pythagorean Theorem Challenge Problems pdf
- Pythagorean Theorem questions pdf