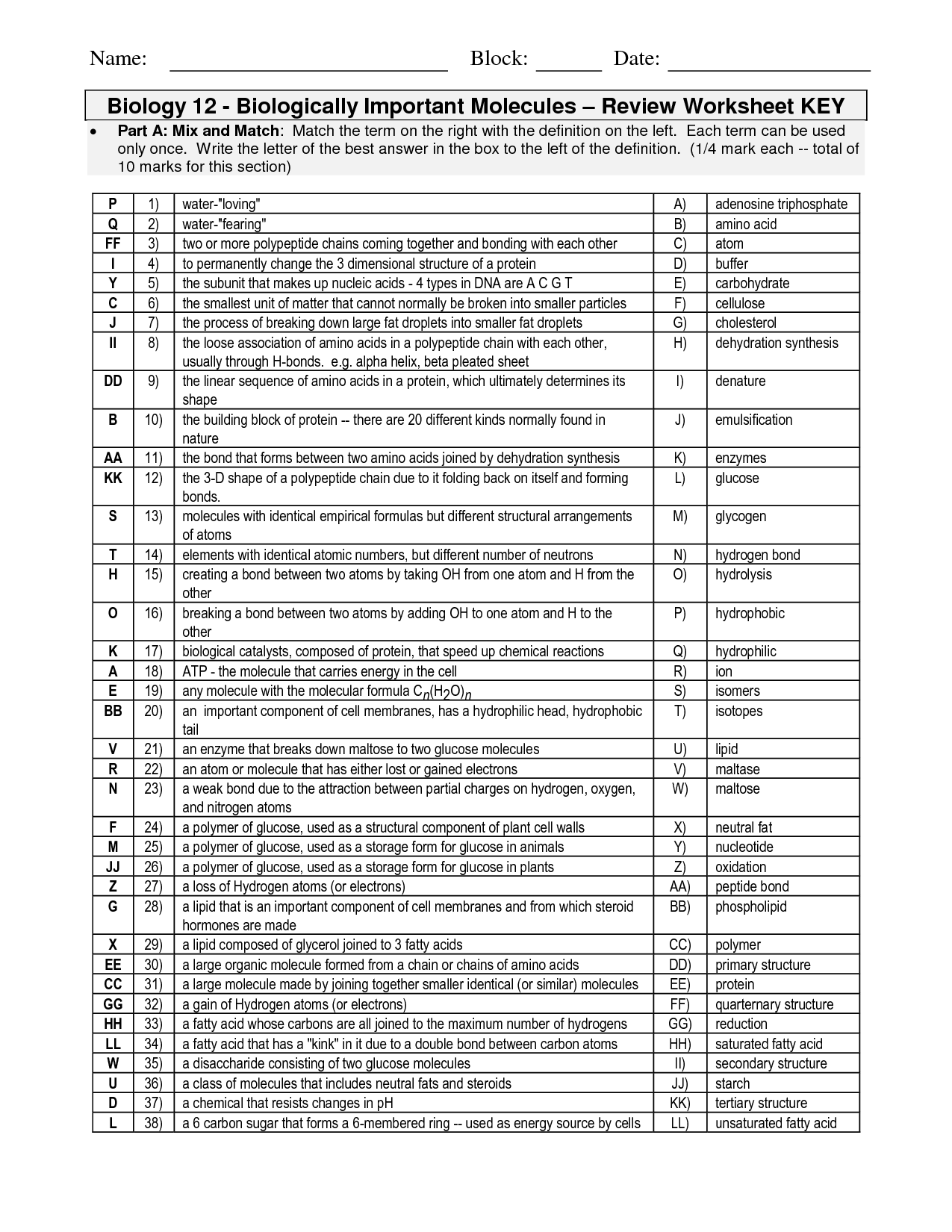
5 Ways to Master Proportional Relationships
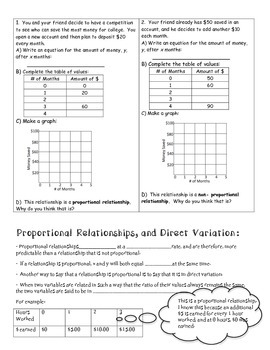
Understanding Proportional Relationships: A Key to Unlocking Math Potential
Proportional relationships are a fundamental concept in mathematics, and mastering them can open doors to solving complex problems with ease. From scaling recipes to calculating distances, proportional relationships are essential in various aspects of life. In this article, we will explore five effective ways to master proportional relationships, helping you to become more confident in your math skills.
1. Visualize the Relationship with Graphs
Visualizing proportional relationships can help you understand the concept better. Graphs are an excellent tool to illustrate the relationship between two quantities. By plotting points on a coordinate plane, you can see how the quantities change in relation to each other.
Consider the example of a recipe that requires a proportional amount of ingredients. If you want to make half the recipe, you’ll need half the amount of each ingredient. By plotting the points on a graph, you can see that the relationship between the quantities is linear.
| Ingredient | Quantity |
|---|---|
| Flour | 2 cups |
| Sugar | 1 cup |
| Butter | 1⁄2 cup |
If you want to make half the recipe, the quantities will be:
| Ingredient | Quantity |
|---|---|
| Flour | 1 cup |
| Sugar | 1⁄2 cup |
| Butter | 1⁄4 cup |
By analyzing the graph, you can see that the relationship between the quantities is proportional.
📝 Note: Graphs can help you identify proportional relationships, but they are not the only tool. Make sure to practice other methods as well.
2. Use Unit Rates to Simplify Complex Problems
Unit rates are a powerful tool to simplify complex problems involving proportional relationships. A unit rate is a rate with a denominator of 1. By converting a rate to a unit rate, you can easily compare and calculate quantities.
For example, consider a car that travels 250 miles in 5 hours. What is the car’s speed in miles per hour?
To find the unit rate, divide the distance by the time:
250 miles ÷ 5 hours = 50 miles per hour
Now, if you want to calculate the distance the car travels in 3 hours, you can use the unit rate:
50 miles per hour x 3 hours = 150 miles
Using unit rates can help you solve complex problems with ease and accuracy.
3. Practice with Real-World Examples
Practicing with real-world examples can help you master proportional relationships. Try to identify proportional relationships in your everyday life, such as:
- Scaling recipes
- Calculating distances
- Measuring ingredients
- Comparing prices
By practicing with real-world examples, you can develop a deeper understanding of proportional relationships and how to apply them in different contexts.
4. Use Proportional Relationship Tables
Proportional relationship tables are a useful tool to organize and analyze data. By creating a table with proportional relationships, you can identify patterns and make predictions.
For example, consider a table with the following data:
| Time (hours) | Distance (miles) |
|---|---|
| 2 | 100 |
| 4 | 200 |
| 6 | 300 |
By analyzing the table, you can see that the relationship between time and distance is proportional. You can use this information to make predictions and calculate unknown values.
5. Play with Proportional Relationship Games
Playing games that involve proportional relationships can make learning fun and engaging. You can try online games, puzzles, or activities that involve proportional relationships, such as:
- Proportional relationship bingo
- Proportional relationship puzzles
- Scaling games
By playing games that involve proportional relationships, you can develop your skills in a fun and interactive way.
As we conclude, mastering proportional relationships is a crucial aspect of mathematics. By visualizing the relationship with graphs, using unit rates, practicing with real-world examples, using proportional relationship tables, and playing games, you can develop a deep understanding of proportional relationships and become more confident in your math skills.
What is a proportional relationship?
+A proportional relationship is a relationship between two quantities that can be expressed as a ratio or fraction.
How can I identify proportional relationships?
+You can identify proportional relationships by analyzing graphs, using unit rates, and looking for patterns in data.
What is a unit rate?
+A unit rate is a rate with a denominator of 1. It can be used to simplify complex problems involving proportional relationships.
Related Terms:
- teacher synergy llc
- IXL
- Khan Academy
- BrainPOP
- Udacity
- Duolingo