6 Essential Steps for Mastering Order of Operations

Mastering the order of operations is a crucial aspect of mathematics that can significantly impact one’s ability to solve mathematical problems with accuracy and efficiency. The order of operations is a fundamental concept that provides a standardized approach to solving mathematical expressions, ensuring that calculations are performed in a consistent and predictable manner. In this article, we will explore the six essential steps for mastering the order of operations.

Step 1: Understanding the Order of Operations

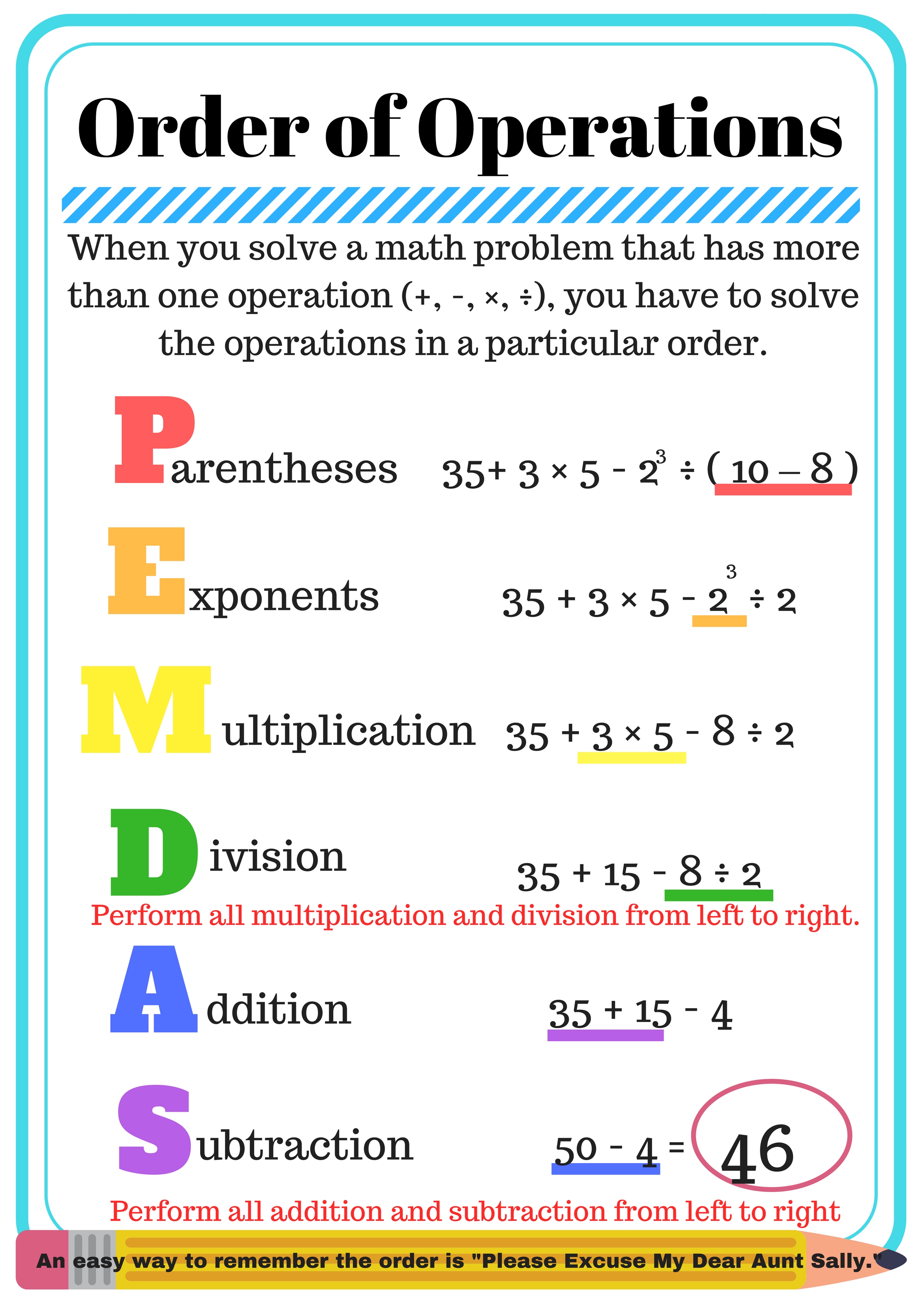
The order of operations is a set of rules that dictates the order in which mathematical operations should be performed when there are multiple operations in an expression. The acronym PEMDAS is commonly used to remember the order of operations:

• Parentheses: Evaluate expressions inside parentheses first.
• Exponents: Evaluate any exponential expressions next.
• Multiplication and Division: Evaluate multiplication and division operations from left to right.
• Addition and Subtraction: Finally, evaluate any addition and subtraction operations from left to right.

Step 2: Simplifying Expressions Inside Parentheses

When simplifying expressions inside parentheses, it is essential to evaluate any operations within the parentheses before moving on to the next step. This may involve evaluating exponential expressions, multiplication, division, addition, or subtraction within the parentheses.

📝 Note: When evaluating expressions inside parentheses, it is crucial to follow the order of operations within the parentheses.

For example:

(3 × 2) + 10

To simplify this expression, we need to evaluate the multiplication operation inside the parentheses first:

3 × 2 = 6

Then, we can add 10 to get the final result:

6 + 10 = 16

Step 3: Evaluating Exponents

Exponents are shorthand notation for repeated multiplication. For example, 2³ is equivalent to 2 × 2 × 2. When evaluating exponents, it is essential to follow the rules of exponentiation:

• A positive exponent indicates the number of times the base is multiplied by itself.
• A negative exponent indicates the reciprocal of the base raised to the positive exponent.
• A zero exponent indicates that the result is equal to 1.

For example:

2³ = 2 × 2 × 2 = 8

(-3)² = (-3) × (-3) = 9

Step 4: Evaluating Multiplication and Division Operations

Multiplication and division operations should be evaluated from left to right. This means that any multiplication or division operation that appears on the left side of the expression should be evaluated before any operation that appears on the right side.

For example:

6 ÷ 2 × 3

To evaluate this expression, we need to follow the order of operations:

1. Divide 6 by 2: 6 ÷ 2 = 3
2. Multiply 3 by 3: 3 × 3 = 9

Step 5: Evaluating Addition and Subtraction Operations

Finally, any addition and subtraction operations should be evaluated from left to right. This means that any addition or subtraction operation that appears on the left side of the expression should be evaluated before any operation that appears on the right side.

For example:

2 + 3 - 1

To evaluate this expression, we need to follow the order of operations:

1. Add 2 and 3: 2 + 3 = 5
2. Subtract 1 from 5: 5 - 1 = 4

Step 6: Combining All the Steps

To master the order of operations, it is essential to combine all the steps in a consistent and predictable manner. This involves evaluating expressions inside parentheses, exponents, multiplication and division operations, and finally, addition and subtraction operations.

📝 Note: The key to mastering the order of operations is to follow the same steps consistently, regardless of the complexity of the expression.

By following these six essential steps, you can ensure that you are evaluating mathematical expressions accurately and efficiently.

In conclusion, mastering the order of operations requires a deep understanding of the rules that govern mathematical expressions. By following these six essential steps, you can develop a strong foundation in mathematics and improve your ability to solve complex mathematical problems.